Assessment of the Influence of Water Saturation and Capillary Pressure  Gradients on Size Formation of Two-Phase Filtration Zone  in Compressed Low-Permeable Reservoir

Valentin A. Korotenko; Sergei I. Grachev; Nelly P. kushakova; Semyon F. Mulyavin

doi:10.31897/PMI.2020.5.9

Vol 245

Pages:

569-581

Download volume:

RUS ENG

Oil and gas

Assessment of the Influence of Water Saturation and Capillary Pressure Gradients on Size Formation of Two-Phase Filtration Zone in Compressed Low-Permeable Reservoir

Authors:

Valentin A. Korotenko¹

Sergei I. Grachev²

Nelly P. kushakova³

Semyon F. Mulyavin⁴

About authors

1 — Ph.D. Docent Tyumen Industrial University
2 — Ph.D., Dr.Sci. Professor Tyumen Industrial University
3 — Ph.D. Docent Tyumen Industrial University
4 — Ph.D., Dr.Sci. professor Tyumen Industrial University

Date submitted:

2020-05-05

Date accepted:

2020-10-05

Date published:

2020-11-24

Abstract

The paper examines the influence of capillary pressure and water saturation ratio gradients on the size of the two-phase filtration zone during flooding of a low-permeable reservoir. Variations of water saturation ratio s in the zone of two-phase filtration are associated with the pressure variation of water injected into the reservoir; moreover the law of variation of water saturation ratio s ( r , t ) must correspond to the variation of injection pressure, i.e. it must be described by the same functions, as the functions of water pressure variation, but be subject to its own boundary conditions. The paper considers five options of s ( r , t ) dependency on time and coordinates. In order to estimate the influence of formation and fluid compressibility, the authors examine Rapoport – Lis model for incompressible media with a violated lower limit for Darcy’s law application and a time-dependent radius of oil displacement by water. When the lower limit for Darcy’s law application is violated, the radius of the displacement front depends on the value of capillary pressure gradient and the assignment of s function. It is shown that displacement front radii contain coefficients that carry information about physical properties of the reservoir and the displacement fluid. A comparison of two-phase filtration radii for incompressible and compressible reservoirs is performed. The influence of capillary pressure gradient and functional dependencies of water saturation ratio on oil displacement in low-permeable reservoirs is assessed. It is identified that capillary pressure gradient has practically no effect on the size of the two-phase filtration zone and the share of water in the arbitrary point of the formation, whereas the variation of water saturation ratio and reservoir compressibility exert a significant influence thereupon.

Keywords:

low-permeable reservoir two-phase filtration displacement front radius gradients of capillary pressure and water saturation ratio reservoir compressibility

10.31897/PMI.2020.5.9

Introduction. In the process of oil field development, under reservoir stimulation on the boundary of three phases “solid substance – oil”, “solid substance – displacement agent” and “oil – displacement agent” surface tensions arise, which affect the processes of fluid filtration, formation of remaining oil in place and selection of technologies for its extraction [1, 6, 8, 11]. Therefore, in order to describe two-phase filtration in the flooding zone, it is necessary to take into account capillary pressure gradient, which varies with the saturation of pore space with displacement fluid. Capillary pressure gradient contributes to oil displacement in water-wet reservoirs and prevents it in oil-wet channels. If radii of capillary channels are small, then the lower limit for Darcy’s law application is violated, which is characteristic of low-permeable seams in structurally complex reservoirs.

Statement of the problem. A difficult task in the process of managing oil field development with flooding is control over the size of the two-phase filtration zone. The solutions for low-permeable reservoirs are ambiguous. For example, there are known experimental dependencies of capillary pressure p_k> on water saturation ratio s for high- and low-permeable water- and oil-wet formations [9]. Similar studies use Rapoport – Lis model, which takes into account the influence of capillary pressure gradient on the processes of two-phase filtration in terms of classical Darcy’s law implementation.

In high-permeable seams (HS) with large radii of menisci curvature, the capillary pressure gradient is neglected. In low-permeable seams (LS), the radii of pore channels are smaller than the radii of HS channels by a factor of tens or hundreds, the radii of menisci curvature are also significantly smaller, therefore under certain values of mobility ratio the lower limit for Darcy’s law application is violated. As capillary pressure is a function of water saturation ratio, dependent on time and coordinates, selection and assignment of s(r, t) functional dependency also affect filtration and oil displacement by water. Hence, the variance of capillary pressure gradient in the zone of two-phase filtration of oil displacement by water must affect the distribution of pressure, phase saturation and, eventually, well productivity and recovery of hydrocarbon reserves.

Methodology. In paper [5], basing on the analysis of mobility ratio $\ {k / {\mu}}$ of viscoplastic oil (VPO) and low-viscosity oil in low-permeable reservoirs, a violation criterion for the lower limit of Darcy’s law application is derived. If $\ {k / {\mu}}$ < 10 mD / mPa∙s, then the filtration equation corresponds to generalized Darcy’s law:

$$ v_i^{\omega}=- \frac {k_i}{\mu_i}(grad\ p_i-{\mathrm g_i^{\rho}}), {v_i^{\rho}}>0\ при\ grad\ p_i> {\mathrm g_i^{\omega}},\ i=1,2,v_i^{\omega}>0, \tag*{(1)}$$

где i = 1, 2 – for water and oil; v_i – are velocities of phase filtration; k_i – are phase permeabilities; $\ {{\mu}_i}$ – are dynamic viscosity coefficients; grad p_i – are gradients of phase pressure; grad g_i – are initial pressure gradients.

In studies [1, 5, 11, 14], the filtration equation for VPO, or generalized Darcy’s law, is expressed in different forms. They have the following common trait: fluid motion in the porous medium starts at current pressure gradient p, greater than some initial value g.

In the process of VPO filtration, initial pressure gradient g represents internal resistance between the layers of moving high-viscosity fluid. For fluid filtration in low-permeable reservoirs, the physical meaning of initial gradient is different – specifically, g corresponds to the capillary pressure gradient, i.e. takes into account surface phenomena on the interphase boundary. The influence of the initial gradient on gas displacement by water in low-permeable reservoirs is studied in paper [12].

In the monograph by V.A.Ivanov, V.G.Khramova and D.O.Diyarov “The Structure of Pore Space in Oil and Gas Reservoirs” (1974), a classification of pore channels depending on their size and the mechanism of mass transfer is presented. Research on physical adsorption, certain aspects of diffusion and agitation requires a fractional characteristic of internal structure in the range of micro- and ultramicropores.

Macropore capillary channels are of the greatest interest in the studies of oil displacement by water and aqueous solutions. Indeed, the radius of water molecule equals $\ r_в {\approx}2\cdot10^{-6}\ мм<< r_k $. In micropore and ultramicropore seams, the radius of water molecule is comparable to or less than the channel radii; diffusion processes, which occur there, require further research and are not addressed in this paper.

The value of capillary pressure is defined by the formula:

$$p_k=2\frac{\sigma cos\theta}{r_k},\tag*{(2)}$$

where $\ \sigma $ – is the surface tension on the boundary between liquid phases; $\ \theta $ – is the contact angle; $\ r_k $ – is the radius of the pore channel [7]. With a decrease in $\ r_k $, and, consequently, in permeability, capillary pressure increases.

In the monograph by Yu.Ya.Bolshakov “The Theory of Capillarity in Oil and Gas Accumulation” (1997), by means of processing experimental data an approximate formula of the dependency between capillary pressure and absolute permeability is obtained:

$$p_k=5,2\cdot10^{-2}(\frac{1}{k})^{0,49}\approx \frac{5,2\cdot10^{-2}}{\sqrt{k}} ,\tag*{(3)}$$

where $\ p_k $ – is the capillary pressure, MPa; k – is the coefficient of absolute permeability, mD. With a decrease in permeability, capillary pressure increases.

In order to solve the problem of oil displacement by water, the following experimental dependencies are used in underground hydrodynamics:

$$p_k=p_k(s)\ или\ p_k=\sigma cos \theta {\sqrt{\frac{m}{k}}J(s)} ,\tag*{(4)}$$

where m is the porosity coefficient; s is the water saturation ratio; J(s) is the Leverett J-function.

With an increase in s, capillary pressure decreases. For water-wet (imbibition) and oil-wet (drainage) reservoirs, quality dependencies of capillary pressure on water saturation are presented in papers [7, 9].

Formulas (2) and (3) reflect a static dependency of $\ p_k $ on permeability and characteristic dimensions of pore channels. Equations (4) allow to take into account the dependency between $\ p_k $ and water saturation ratio s, the value of which decreases as the share of water in the reservoir rises in the process of field exploitation. Distribution of water saturation ratio in the area of two-phase filtration is a necessary condition for the calculation of development parameters.

In the implementation of Darcy’s law there are several models that take into account the influence of capillary pressure. For incompressible media there is Rapoport – Lis model, in which a differential equation is obtained to estimate water saturation ratio. In order to study compressible media, a model by V.N.Nikolayevsky, K.S.Basniyev and A.T.Gorbunov is proposed [1, 3, 10], which contains two differential equations and an expression of a dependency between pressure and water saturation ratio (closing equation), obtained from the condition of single-phase mass conservation in the elementary volume.

Research results. The capillary pressure gradient for plane-radial filtration is:

$$ {\mathrm g}(r,t)=\frac{∂ p_k(r,t)}{∂r}=\frac{∂p_k}{∂s}\frac{∂s}{∂r}=Dp_k\frac{∂s}{∂r}, \tag*{(5)}$$

where r – is the coordinate; t is time; Dp_k is the s-derivative of capillary pressure; $\ \frac{∂s}{∂r} $ – is the gradient of water saturation ratio.

Consequently, for a p_k(s) function, plotted basing on data from laboratory studies, and a known dependency of water saturation ratio s(r,t) it is possible to estimate the value of capillary gradient pressure. This holds true for both water- and oil-wet reservoirs. The influence of constant initial pressure gradient on the filtration of low-viscosity fluids in low-permeable differences is addressed in paper [5]. Current paper studies the dependency of capillary pressure gradient on time and coordinates.

Detailed studies of physical properties of YuS₂-YuS₄ formations at Severo-Chupalskoye oilfield were carried out by V.V.Semenov, I.B.Ratnikov et al. in 2013. Dependencies of capillary pressure p_kf(s_b) on water saturation ratio (4) were plotted using centrifuge method. A relationship between capillary pressure and pore space structure was defined considering rock and fluid properties according to Laplace formula (2). Experiments on phase permeability estimation were performed in accordance with the industry standards (GOST 26450.0-85 Rocks. General requirements for sampling and sample preparation for determination of collecting properties. GOST 26450.2-85 Rocks. Method for determination of absolute gas permeability coefficient by stationary and non-stationary filtration). 281 core samples, collected in the well 226, were examined. The rocks were represented by fine- and coarse-grained aleurolites, intercalations of alurolites and argillites, medium- and coarse-grained sandstones. Total formation thickness amounted to 32 m. Permeability coefficients were estimated using ultracentrifugation and membrane methods. Absolute permeability of samples varied in the range of 0.1-11.2 mD. Open porosity coefficient varied from 0.07 to 0.18; ratio of residual water saturation – from 0.35 to 0.61.

A characteristic dependency of p_k(s) on water saturation ratio s(r,t) for low-permeable differences is presented in Fig.1, а.

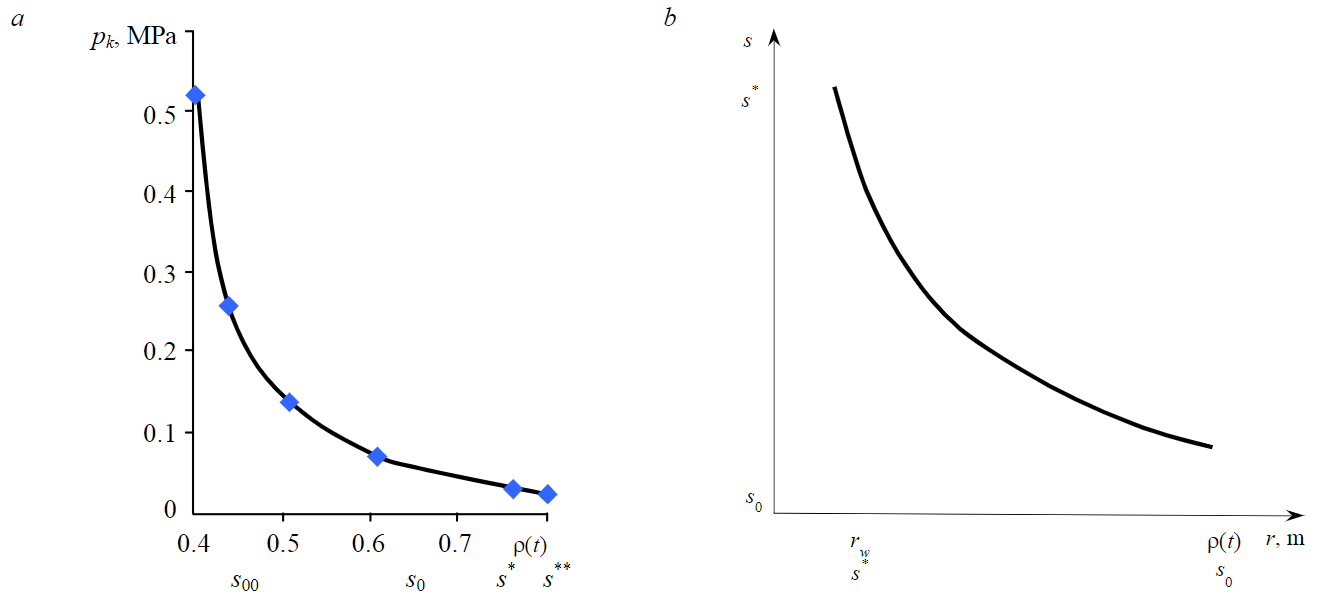
Fig.1. Dependency of capillary pressure on water saturation ratio for the sample 34766-14 (a) and the variation pattern of water saturation ratio during oil displacement by water in the zone of two-phase filtration (b) s₀₀ ≈ 0.4 is the ratio of residual water saturation; s** ≈ 0.8 is the ratio of cutoff water saturation; s₀ ≈ 0,627 is the ratio of water saturation, corresponding to the beginning of two-phase filtration; s* ≈ 0.749 is the ratio of water saturation, at which two-phase saturation stops; r_c is the well radius; ρ(t) is the radius of the two-phase filtration zone.

Water saturation ratio in the zone of two-phase filtration depends on pressure variation of the water, injected into the formation, and has to meet the following criteria. For r = r_c water saturation ratio equals s(r_c)=s*. At the displacement front, when r = ρ(t) water saturation ratio equals, respectively, s(ρ(t))=s₀. In the range $\ r_c\le r\le \rho (t)$ water saturation ratio s(r,t) is a decreasing function, its derivative $\ \frac{∂s}{∂r}<0$. The pressure of water, injected into the formation, p(r,t) also decreases from its maximum value at the bottomhole of the injection well to the initial reservoir pressure at the displacement front. Thus, the law of s(r,t) variation must correspond to the variation of injection pressure, i.e. for plane-radial filtration it must be described by the same functions r/ρ(t), ln(r/ρ(t)), as the function of water pressure variation, but s(r,t) must satisfy its own boundary conditions. s(r,t) or s(Δp) dependency is a closing equation in the description of two-phase filtration processes. Hence, capillary pressure gradient (5) depends on the selected functional dependency of water saturation ratio in the zone of two-phase filtration.

Paper [5], demonstrates that in water-wet reservoirs the lower limit for Darcy’s law application is violated, when $\ k_в/\mu_в\le10$ mD/mPa∙s. Here k_в=kk_в* – is the phase permeability of the reservoir to water; k is the absolute permeability; k – is the absolute permeability; k_в*(s) – is the relative phase permeability (RPP) to water.

The lower limit for Darcy’s law application equals the value of mobility ratio 10 mD/mPa•s [5]. Let us assume that k = 20 mD, $\ \mu_в$ = 1 mPa∙s, maximum value of 0,4. Then $\ k_в/\mu_в=20\cdot0,4/1=8$ mD/mPa∙s, therefore, a violation of Darcy’s law takes place. Consequently, in the area of oil drainage (water-free zone), given s=s₀ and maximum values k_н*= 0,7, $\ \mu_н$ = 1,5 mPa∙s, we have $\ k_н/\mu_н=20\cdot0,7/1,5=9,3$ mD/mPa∙s – i.e. the lower limit for Darcy’s law application is violated. Two-phase filtration zone corresponds to non-zero variation of relative phase permeability coefficients.

Fig.2 depicts the results of k_в*(s) от коэффициента водонасыщенности для образца 34766-14. dependency on water saturation ratio for the sample 34766-14. The two-phase filtration zone corresponds to the variation of water saturation ratio from s₀ = 0,627 to s* = 0,749.

Fig.2. Dependencies of RPP on the water saturation ratio for the sample 34766-14 1 is the Leverett’s J(s) function; 2 – k_в*(s)

Let us analyze oil displacement by water considering capillary pressure gradient; the origin of coordinates is set at the bottomhole of the injection well. In order to solve this task, motion (1) and continuity equations are utilized:

$$ {\frac{∂(m\rho_is_i)}{∂t}}+div(\rho_iv_i)=0, \tag*{(6)}$$

where index i = 1 stands for water, index i = 2 – for oil, m – is the porosity coefficient; ρ₁, ρ₂ – are the densities of water and oil. In order to estimate variable radius of the displacement front, Barenblatt’s method of integral equations is used [15].

Option 1. Let us consider Rapoport – Lis model. The formation and the fluids are incompressible, fluid densities ρ_i and porosity coefficient m are considered constant.

In paper [5], the distribution of water saturation ratio for plane-radial filtration is formulated as follows:

$$\begin{gathered}s=s_0+\Delta s\frac{f_1(r,t)}{f_{1c}(t)},\ f_1(r,t)=1-(\frac{r}{\rho(t)})^n+n\ln\frac{r}{\rho(t)} \le0, \\ f{_1c}(r_{c},t)=1-(\frac{r_{c}}{\rho(t)})^{n}+n \ln \frac{r_c}{\rho(t)} \le0; \ \frac{∂ s}{∂ r}= \frac{n\Delta s}{rf_{1c}}(1-\frac{r^n}{\rho(t)^n}), \end{gathered} \qquad\tag*{(7)}$$

where n – is a numeric parameter.

In order to derive the saturation equation, formula (1) is inserted into continuity equation (6). After all transformations, a differential equation for water saturation during plane-radial filtration is obtained:

$$ {\frac{∂ s}{∂t}}=-\frac{k_0}{m_0\mu_0}\frac{1}{r}\{q_1F-\frac{k_1^*r}{\mu_1\mu_0}[(1+\mu_0)(1+F)\mathrm{g}_1-(1-F)\mathrm{g}_2]\} , \tag*{(8)}$$

where s – is the water saturation ratio; k₀, m₀ – are the coefficients of absolute permeability and open porosity; $\ \mu_0= \mu_1/\mu_2, \mu_1\ and\ \mu_2 $ – are the coefficients of dynamic viscosity; q₁ – is the production rate for plane-radial filtration at a given well flow rate; k*₁(s) – is the coefficient of relative phase permeability to water; g₁, g₂ – are the gradients of capillary pressure for oil displacement by water (imbibition) and oil filtration (drainage); F is the Buckley-Leverett function,

$$\begin{gathered}F=\frac{k_1^*}{k_1^*+\mu_0k_2^*};\ q_1=\frac{Q(t)}{2\pi h}; \ \frac{∂ p_2}{∂ r}= \frac{∂ p_1}{∂ r}-\mathrm{g_1}; \\ \frac{∂ p_1}{∂ r}=\Biggl[\frac{v\mu_1}{k_0(k_{1}^*+\mu_0k_{2}^*)}+\mathrm{g_1}(2F-1)+\mathrm{g_2}(1-F)\Biggl];\ v(t)=\frac{Q(t)}{2\pi hr}, \end{gathered} \qquad\tag*{(9)}$$

h is the formation thickness; v(t)=v₁(t)+v₂(t) is the cumulative filtration velocity; v₁(t) is the water filtration velocity; v₂(t) is the oil filtration velocity; Q(t)=Q₁(t)+Q₂(t) are the volume velocities of fluid filtration, water and oil, respectively. At t=0, volume velocity Q=Q₀ and equals injectivity of the well.

With distance from the bottom of the injection well, water saturation ratio decreases; under constant injectivity (rigid elastic water drive) at the displacement front, if s=s₀, r=ρ(t) it follows that Q₀=Q₂.

Equation (8) is integrated for $\ r\in [r_c, \rho(t)]$, as a result a differential equation for the radius of two-phase filtration zone is obtained:

$$ -{\frac{\Delta sm_0n^2}{4(n+2)}\frac{d}{dt}\Biggl(\frac{\rho(t)^2}{f_{1c}(t)}\Biggl)}=q_1-\frac{2(1+\mu_0)k_0k_m^*}{\mu_0\mu_{10}}r_c\mathrm{g_c} . \tag*{(10)}$$

In the calculation of the right-hand side, it is considered that at r=ρ(t) water saturation ratio equals s=s₀, therefore $\ k_1^*(s_0)=F(s_0)=0 $. For $\ r=r_c $ water saturation ratio equals $\ s=s^* $, therefore $\ k_1^*(s^*)=k_m^* $ – the maximum value, $\ F(s^*)=1,\ \mathrm{g_1}(s^*)=\mathrm{g_c} $ take on values on the boundary of the filtration zone.

After integrating equation (10), a formula for the radius of the two-phase filtration zone in the incompressible reservoir is obtained:

$$ \rho(t)=\sqrt{r_c^2-\frac{4(n+2)}{n^2m_0\Delta s}f_{1c}(t) \Biggl(\int^t_0q_1(t)dt-\frac{2(1+\mu_0)r_ck_0k_m^*\mathrm{g_c}}{\mu_0\mu_{10}}t \Biggl) } . \tag*{(11)}$$

Capillary pressure gradient g is determined from formulas (5) and (7):

$$ \mathrm{g_1}(r,t)=Dp_k\frac{\Delta sn}{rf_{1c}(r_ct)}\Biggl(1-\frac{r^n}{\rho(t)^n}\Biggl);\ \mathrm{g_1}(r_c,t)=\mathrm{g_{c1}}=\frac{∂ p_k(r,t)}{∂ r} ❘_{r=rc}=Dp_{kc}\frac{n\Delta s}{r_cf_{1c}};\ \mathrm{g_{10}}(\rho(t),t)=0 . \tag*{(12)}$$

In transcendental equation (11), the function $\ f_{1c}(r_c, \rho(t))<0 $ for all argument values. Equation (11) is solved using an iterative method. For bounded areas of plane-radial filtration $\ \rho(t) \le R $ and a constant value of q₁ an approximate formula for the radius of a two-phase filtration zone is obtained:

$$ \rho(t)= \sqrt{r_c^2-\frac{4(n+2)}{n^2m_0\Delta s}f_{1c}(R)\Biggl(q_1-\frac{2(1+\mu_0)r_ck_0k_m^* \mathrm{g_{c1}}}{\mu_0\mu_{10}}t}. $$

Conditions for advancement of the displacement front – existence of two-phase filtration in low-permeable incompressible reservoirs – are the following inequalities:

$$ q_1>\frac{2(1+\mu_0)r_ck_0k_m^* \mathrm{g_{c1}}}{\mu_0\mu_{10}};\ Q>\frac{4\pi h(1+\mu_0)r_ck_0k_m^* \mathrm{g_{c1}}}{\mu_0\mu_{10}}. $$

Let us examine how violation of the lower limit for Darcy’s law application affects the filtration of compressible fluids in low-permeable reservoirs, depending on the methods for selecting a function of water saturation ratio s(r,t).

After omitting index 1 for the displacement fluid (water), rheological equations for pressure dependencies of fluid density, coefficients of porosity, permeability and dynamic viscosity are defined as follows:

$$ \rho_1=\rho_{10}\exp(\alpha_p\Delta p);\ m=m_0\exp(\alpha_m\Delta p); k=k_0\exp(\alpha_k\Delta p);\ \mu_1=\mu_{10}\exp(\alpha_{\mu}\Delta p), \tag*{(13)}$$

where $\ \alpha_{\rho},\ \alpha_{m},\ \alpha_{k},\ \alpha_{\mu} $ – are the coefficient that characterize dependency of density, porosity, permeability and viscosity on pressure variation, overbalance pressure Δp.

After inserting (13) and motion equation (1) into water continuity equation (6) for plane-radial filtration, we obtain:

$$\begin{gathered}m_0\rho_{10}\frac{∂ (s\ exp(\alpha_1 \Delta p))}{∂ t}=\rho_{10}\frac{k_0}{\mu_{01}}\frac{1}{r}\frac{∂ (r Φ_{2})}{∂ r};\ Φ_2=\exp(\alpha_2 \Delta p)k_1^*(s))\Biggl(\frac{∂ p}{∂ r}+\mathrm{g}\Biggl); \\ \alpha_1=\alpha_{\rho}+\alpha_m;\ \alpha_2=\alpha_{\rho}+\alpha_{k}-\alpha_{\mu}. \end{gathered} \qquad\tag*{(14)}$$

Taking into account that $\ \alpha_2 \Delta p<<1 $, we obtain that function $\ Φ_2 \approx k_1^*(s)\Biggl(\frac{∂ p}{∂ r}+\mathrm{g}\Biggl); $.

Pressure distribution is determined in the form:

$$ p(r,t)=\alpha_0+\alpha \ln \frac{r}{\rho(t)}+\alpha_n \frac{r^n}{\rho^n(t)}. $$

Boundary conditions are set as follows:

$$p(0,t)=p(\rho(t))=p_0;\ \frac{∂ p(t)}{∂ r}❘_{r=\rho(t)}=-\mathrm{g_0}, \qquad\tag*{(15)}$$

where g₀ is the capillary pressure gradient at the displacement front.

From conditions (15), pressure distribution is obtained as follows:

$$\begin{gathered} p(r,t)=p_0+a \ln\frac{r}{\rho(t)}+\frac{1}{n}(a+\mathrm{g_0}\rho)\Biggl(1-\frac{r^n}{\rho^n(t)}\Biggl), \\ \Delta p=a \ln \frac{r}{\rho(t)}+\frac{1}{n}(a+\mathrm{g_0}\rho)\Biggl(1-\frac{r^n}{\rho^n(t)}\Biggl).\end{gathered} \qquad\tag*{(16)}$$

Coefficient a is determined from the third boundary condition, assigned for the bottomhole of the injection well.

For the assigned injectivity Q at the bottomhole of the injection well, the following condition is satisfied:

$$r_c\frac{∂ p}{∂ r}❘_{r_c}=a=-(q+\mathrm{g_c}r_c);\ q=\frac{Q}{2\pi \epsilon};\ \epsilon=\frac{k_0k_m^*h}{\mu_{10}} \qquad\tag*{(17)}$$

where $\ \epsilon $ is the coefficient of hydraulic conductivity at the bottomhole of the injection well; K*_m – is the maximum value of RPP to water; h – is the formation thickness; g_c is the capillary pressure gradient at the bottomhole of the well. After inserting expressions (16), (17) into equation (14) and integrating the right-hand side, an equation for displacement front radius is obtained:

$$\int_{r_c}^{\rho(t)}r\frac{∂}{∂t}[s \exp(\alpha_1\Delta p)]dr=\frac{k_0k_m^*}{m_0\mu_{10}}q. \qquad\tag*{(18)}$$

Therefore, in order to estimate ρ(t) it is necessary to define rheological equations and the closing equation of s(r,t). Let us consider the following options.

Option 2. Rheological equations (13) and equations (7) are expressed in the form:

$$\begin{gathered} \rho_1=\rho_{10}(1+\alpha_{\rho}\Delta p);\ m=m_0(1+\alpha_{\rho}\Delta p); k=k_0(1+\alpha_{k}\Delta p); \mu=\mu_{10}(1+\alpha_{\mu}\Delta p); \\ s=s_0(1+\alpha_{s2}f_1(r,t)); \alpha_{s2}=\frac{\Delta s}{s_0f_{1c}(r_c,t)}. \end{gathered} \qquad\tag*{(13.1)}$$

Within the interval $\ r \in[r_c, \rho(t)] $ модуль параметра $\ \alpha_{s2} $ the absolute value of parameter αs2 does not exceed 0.02

As a result of inserting expression (13.1) and (16) into the left-hand side of equation (18) and applying the Leibniz rule after integration, a differential equation is obtained:

$$\frac{d}{dt} \Biggl (\alpha_1\mathrm{g_0}\rho^3+\Biggl(\alpha_1n(q+\mathrm{g_c}r_c)-\frac{n^2\alpha_{2s}}{4(n+2)f_{1c}}\Biggl)\rho^2\Biggl)=\frac{k_0k_m^*}{m_0\mu_{10}}q. $$

Taking into account conditions (12) after integration, the following transcendental equation, solved using iteration method, is obtained for a constant well flow rate:

$$\rho(t)=\sqrt{r_c^2+\chi{_2}qt};\ \chi_2=\frac{k_0k_m^*}{m_0\mu_{10}s_0\Biggl (\alpha_1n(q+{\mathrm g_c}r_c)-\frac{n^2\alpha_{2s}}{4(n+2)f_{1c}(r_c,\rho(t))}\Biggl)} \qquad\tag*{(19)}$$

Displacement front radius is proportional to the square root of time, depends on injectivity and coefficients of compressibility and obeys the assigned variation law of water saturation ratio. If, similarly to option 1, in $\ \chi_2 $ parameter the boundary of filtration area R is substituted for ρ(t), an approximate equation is obtained.

Option 3. In contrast to the dependency of water saturation ratio on Δp, addressed in papers [2, 10], taking into account (16), the closing equation can be written in the form:

$$\begin{gathered} s=s_0 \exp(\alpha_{3s}f_3);\ f_3(r,t)=\frac{1}{n}(a+{\mathrm g_0}\rho(t))\Biggl (1-\frac{r^n}{\rho(t)^n}\Biggl)+a \ln\frac{r}{\rho(t)};\ \alpha_{3s}=\frac{1}{f_{3s}}\ln\frac{s^*}{s_0};\\ f_{3s}(r_c,t)=\frac{1}{n}(a+{\mathrm {g_0}}\rho(t))\Biggl(1-\frac{r_c^n}{\rho(t)^n}\Biggl)+a \ln \Biggl(\frac{r_c}{\rho(t)}\Biggl);\ \frac{∂ s}{∂ r}=\frac{\alpha_{3s}s}{r}\Biggl(a-(a+{\mathrm {g_0}}\rho)\frac{r^n}{\rho^n}\Biggl). \end{gathered} $$

In this case capillary pressure gradient is:

$${\mathrm g}(r,t)=Dp_k\frac{\alpha_{3s}s}{r}\Biggl(a-(a+{\mathrm g_0}\rho)\frac{r^n}{\rho^n}\Biggl). \qquad\tag*{(21)}$$

At $\ r\to ∞ and r=\rho(t) it follows that,\ {\mathrm g}(r,t)\to0. $. Therefore, at the displacement front at s = s0 it is assumed that g0 = 0. For r = r_c и s = s* capillary pressure gradient g(r_c, t) = g_c. From expressions (20) and (21) it can be obtained that:

$${\mathrm {g_c}}(\rho)r_c=\frac{q}{2}\Biggl(\sqrt{1+\frac{4B}{q^2}}-1\Biggl);\ B=\frac{s^*qDpk_c \ln \frac{s^*}{s_0}}{\ln\frac{r_c}{\rho(t)}+\frac{1}{n}}, \qquad\tag*{(22)}$$

where В an auxiliary designation, which simplifies the formula.

Inserting formulas (20) and (22) into equation (18) and considering q a constant, after integration we obtain the formula:

$$\rho(t)=\sqrt{r_c^2+\Biggl(\frac{4(n+2)}{(n+4)}\Biggl)\frac{q}{(\alpha_1+\alpha_{3s}(\rho))(q+{\mathrm g_c}(\rho)r_c)}\chi_3t};\ \chi_3=\frac{k_0k_m^*}{\mu_{10}m_0}. \qquad\tag*{(23)}$$

In order to estimate the radius of displacement front, a set of equations (20), (22), (23) is derived, which can be solved using iteration method. Three iterations suffice to solve the set of equations.

In contrast to the first two options, functions f₃(r, ρ(t)) and f_3c(r, ρ(t)) depend on the assigned boundary conditions of the pressure function. Pressure dependency of s(r, t) function makes the estimation of capillary pressure gradient more difficult.

Option 4. In contrast to option 2, s(r, t) dependency is expressed in the form:

$$s=s_0 \exp(\alpha_{4s}f_4);\ \alpha_{4s}=\frac{1}{f_{4c}}\ln\frac{s^*}{s_0}, \qquad\tag*{(24)}$$

where f₄(r,t) and f_4c(t)=f₄(r_ct) are functions, which in contrast to (7), are assigned in the form:

$$\begin{gathered} f_4(r,t)=1-\Biggl(\frac{r}{\rho(t)}\Biggl)^n+\ln\frac{r}{\rho(t)}\le 0;\ \frac{∂f_4}{∂r}=\frac{1}{r}\Biggl(1-\frac{r^n}{\rho^n}\Biggl); \\ f_{4c}(r_c,t)=1-\Biggl(\frac{r_c}{\rho(t)}\Biggl)^n+\ln\frac{r_c}{\rho(t)}\le0;\ \frac{∂s}{∂r}=\frac{\alpha_{4s}}{r}\Biggl(1-n\frac{r^n}{\rho^n}\Biggl).\end{gathered} $$

Capillary pressure gradient equals:

$${\mathrm g}(r,t)=Dp_k\frac{\alpha_{4s}s}{r}\Biggl(1-n\frac{r^n}{\rho^n}\Biggl). \qquad\tag*{(25)}$$

At r = r_c capillary pressure gradient equals

$${\mathrm g_c}={\mathrm g}(r_c,t)=Dp_{kc}\frac{\alpha_{4s}s^*}{r_c}. \qquad\tag*{(26)}$$

At the displacement front:

$${\mathrm g_0}={\mathrm g}(\rho(t))=\frac{∂p_k}{∂s}|_{s_0}\frac{\alpha_{4s}s_0}{\rho}(1-n)=Dp_{k0}\frac{\alpha_{4s}s_0}{\rho}(1-n).$$

Equation (18) takes the form:

$$\frac{1}{4(n+2)}\frac{d}{dt}[2{\mathrm g_0}\rho^3\alpha_1+\rho^2(\alpha_1n(q+{\mathrm g_c}r_c)+\alpha_{4s}(n-2))]=\frac{k_0k_{max}^*}{\mu_{10}}q;\ \alpha_1=\alpha_{\rho}+\alpha_m. \qquad\tag*{(27)}$$

As a result of inserting g₀ after integration and introducing a designation А, a quadratic equation for ρ(t) is obtained:

$$\begin{gathered} \frac{d(A\rho^2)}{dt}=4(n+2)\frac{k_0k_{max}^*}{\mu_{10}}q;\\ A=2Dp_{k0}s_0\alpha_{4s}\alpha_1(1-n)+\alpha_1n(q+{\mathrm g_cr_c)+(n-2)\alpha_{4s}},\ Dp_{k0}=\frac{∂ p_k}{∂ s}|_{s=s_0},\end{gathered} \qquad\tag*{(28)}$$

from which

$$ \rho= \sqrt{r_c^2+4(n+2)\chi_4\int_0^tqdt},\ \chi_4=\frac{1}{A}. $$

For a constant q

$$ \rho(t)=\sqrt{r_c^2+4(n+2)\frac{k_0k_{max}^*}{\mu_{10}}\chi_4qt}=\sqrt{r_c^2+4(n+2)\frac{Q}{2\pi h}\chi_4t}. \qquad\tag*{(29)}$$

Here a dimensionless parameter χ₄ > 0 accounts for media compressibility and depends on capillary pressure gradient.

Option 5. In paper [5] the capillary pressure gradient g is considered a constant, variation of water saturation ratio is not taken into account. Therefore, formula (5) can be written as follows:

$$ {\mathrm g}=\frac{\Delta p_ks_{cp}}{\Delta s(R-r)_c},\ s_{cp}=\frac{s_0+s^*}{2},\ \frac{∂p_k}{∂s}=\frac{\Delta p_k}{\Delta s},\ \Delta p_k=p_{k_0}-p_{k^*}. \qquad\tag*{(30)}$$

This holds true for a linear dependency of p_k(s) and average value of the function s_cp , p_k₀ and p_k* are capillary pressure values in the points s₀ and s*.

As a result of inserting (30) into the equation (18) after integration and transformations, a cubic equation is obtained

$$ {\mathrm g}\rho^3(t)+n(q+{\mathrm g}r_c)\rho^2(t)=\frac{k_0k_m^*}{\alpha_1m_0\mu_{10}s_{cp}}qt;\ r_c\le\rho(t)\le R, \qquad\tag*{(31)}$$

from which

$$ \rho(t)=^3\sqrt{\Biggl(r_c+\frac{A}{3}\Biggl)^3+Bt}-\frac{A}{3},\ A=\frac{n(q+\mathrm gr_c)}{\mathrm g};\ B=\frac{Q}{2\pi hm_0\mathrm g\alpha_1s_{cp}}. \qquad\tag*{(32)}$$

Assuming that g = 0, from (31) a cubic equation is obtained, from which

$$ \rho(t)=\sqrt{r_c^2+\frac{1}{n}\chi_5t};\ \chi_5=\frac{k_0k_m^*}{\alpha_1\mu_{10}m_0s_{cp}}. \qquad\tag*{(33)}$$

In the five presented options, the radii of the zone of two-phase filtration displacement depend on the gradients of capillary pressure and water saturation. For further calculations it is required to find an s-derivative of capillary pressure at the boundaries of the two-phase filtration zone from Fig.1 and to apply the corresponding closing equation.

A pivot table of s(r, t) dependencies and their derivatives is compiled for five presented options (Table 1).

Table 1

Water saturation ratio s(r, t) and water saturation gradients for options 1-5

Option	Parameters
Option	s, α_is	∂ s/∂ r
1	$\ s=s_0+\Delta s\frac{f_1(r,t)}{f_{1c}(t)} $	$\ \frac{∂ s}{∂r}=\frac{n\Delta s}{rf_{1c}}\Biggl(1-\frac{r^n}{\rho(t)^n}\Biggl) $
2	$\ s=s_0(1+\alpha_{s2}f_1(r,t));\ \alpha_{s2}=\frac{\Delta s}{s_0f_{1c}(r_c,t)} $	$\ \frac{∂ s}{∂ r}=\frac{n\Delta s}{rf_{1c}}\Biggl(1-\frac{r^n}{\rho(t)^n}\Biggl) $
3	$\ s=s_0\exp(\alpha_{3s}f_3);\ \alpha_{3s}=\frac{1}{f_{3c}}\ln\frac{s^*}{s_0}$	$\ \frac{∂ s}{∂r}=\frac{\alpha_{3s}s}{r}a\Biggl(1-\frac{r^n}{\rho^n}\Biggl) $
4	$\ s=s_0\exp(\alpha_{4s}f_4);\ \alpha_{4s}=\frac{1}{f_{4c}}\ln\frac{s^*}{s_0}$	$\ \frac{∂ s}{∂r}=\frac{\alpha_{4s}s}{r}\Biggl(1-n\frac{r^n}{\rho^n}\Biggl) $
5	$\ s_{cp}=\frac{s_0+s^*}{2}$	$\ \frac{∂ s}{∂r}=\frac{s_{cp}}{(R-r_{c})} $

In options 1, 2, 4, closing equations of s(r, t) are obtained using the same functions, as the function of water pressure distribution, but satisfy their own boundary conditions. In option 3 f₃, f_3c functions depend on the injection pressure, which in its own turn depends on well injectivity – parameter a= –q–g_cr_c. In option 5, water saturation gradient is defined as a ratio of average water saturation to the size of the filtration zone.

In order to identify the two-phase filtration zone, a time variation of displacement front radii ρ(t), estimated in options 1-5, is analyzed.

Example. Suppose we are given:

$$\begin{gathered} h = 15 м; Q = 20 м^3 / сут; r_c = 0,1 м; k_0 = 2,1 мД; k_m^*= 0,05;\\ \mu_{10} = 0,305 мПа∙с; \mu_{20} = 1,53 мПа∙с; m_0 = 0,16; \alpha_1 = 2∙10^{–9}\ 1 / Па;\\ R = 2000 м; s_0 = 0,627; s^* = 0,749; Dp_{kc} = –0,215 МПа; Dp_{k0} = –0,254 МПа; \\p_{ks0} = 0,067 МПа; p_{ks*} = 0,034 МПа.,\end{gathered} $$

Table 2 contains estimation results of displacement front radii using formulas from different options.

Table 2

Estimation of radius ρ(t) using formulas (11), (19), (23), (29), (32), (33) for n= 1, 2

t, days	ρ(t), m
t, days	(11)	(11)	(23)	(29)	(32)	(33)
1	23	10	7	7	0,11	11
50	197	72	59	56	0,51	75
100	287	103	85	80	0,93	106
200	416	146	123	116	1,75	150
365	574	197	169	158	3,11	203
730	830	280	243	228	6,13	287

For the same time point, displacement front radius in the incompressible medium (11) is by several times greater than values corresponding to the size of the compressible reservoirs, which take into account the variation law of water saturation ratio (19), (23), (29). Thus, for t = 200 days ρ(t) of the incompressible reservoir exceeds the radius of compressible reservoirs by 3-3.5 times. If the capillary pressure gradient and the water saturation ratio are constant, then at g = const it follows from (32) that propagation of the two-phase filtration zone occurs at a much slower pace. It is identified that for a fixed value of time at n< 1 the values of radii increase, whereas at n > 1 they decrease. Parameter n is determined by comparison of actual and estimated characteristics. Assuming that g = 0, the values of radii stay practically the same, excluding formula (33) of option 5, where the radius is determined using a quadratic equation and its values are comparable to the radius in formula (19) of option 2. Hence, variation (influence) of capillary pressure gradient in the two-phase filtration zone of low-permeable differences can be neglected. The greatest values of capillary pressure gradient are by two orders of magnitude lower than current gradients of water pressure. Therefore, it can be considered that fluid filtration obeys classical Darcy’s law. If a constant value of R ρ(t) in f_ic functions in formulas (11), (19), (23), (29), then for n = 1, 2 the values of displacement radius exceed the results obtained using iterative formulas. Percentage error in the time interval t = 1-730 days varies: from 41 to 5 % according to formula (11); from 7 to 1 % according to (19); from 41 to 9 % according to (23); from 36 to 8 % according to (29).

Interval boundaries of p_k and k* variation due to water saturation ratio differ from each other (Fig.1, 2). The same holds true for other examined samples as well. For the sample 34766-14, at the bottomhole of the injection well at r_c = 0,1 m water saturation ratio equals s** = 0,8, whereas two-phase filtration zone starts at s* = 0,764, which corresponds to r₀, the value of which is determined from the closing equation of s(r,t), addressed in options 1-4. Performed calculations demonstrate that at 7 m ≤ ρ(t) ≤ 2000 m the values of $\ r\in [0,16; 0,32]$ m. Therefore, substitution of r_c by r₀ in the formulas of displacement radii has practically no effect. The interval of $\ r\in[r_c;r_0]$ is characterized by piston displacement of oil by water. Two-phase filtration starts at $\ r \ge r_0 $.

Let us estimate water cut ratio $\ \omega $ of the operating production well, located at the distance r = L. In paper [2] a calculation method is proposed, in which capillary pressure is estimated by means of the Leverett J-function (4) and capillary pressure gradient depends on the coordinate derivative of the Leverett J-function. For the capillary pressure gradient, defined by formula (5), the water cut ratio equals:

$$ \omega=\frac{k^*(s)}{k^*(s)+\mu_0Dk_2^*(s)};\ D=1+\frac{{\mathrm g}(r,t)}{\frac{∂ p}{∂ r}+{\mathrm g}(r,t)}=1+\frac{{\mathrm g}(L)L}{a\Biggl(1-\frac{L^n}{\rho^n}\Biggl)+{\mathrm g}(L)L}, \qquad\tag*{(34)}$$

where g(r, t) is the variable capillary pressure gradient for oil displacement by water k*₂(s) is the relative phase permeability of the reservoir to oil. Parameter D takes into account the influence of capillary pressure gradient on the water cut.

In order to estimate the share of water at the fixed point of the formation, functional dependencies of RPP coefficients on water saturation ratio are plotted. For the sample 34766-14 (Fig.2) the following results are obtained:

$$\begin{gathered} \frac{d(A\rho^2)}{dt}=4(n+2)\frac{k_0k_{max}^*}{\mu_{10}}q;\\ A=2Dp_{k0}s_0\alpha_{4s}\alpha_1(1-n)+\alpha_1n(q+{\mathrm g_cr_c)+(n-2)\alpha_{4s}},\ Dp_{k0}=\frac{∂ p_k}{∂ s}|_{s=s_0},\end{gathered} \qquad\tag*{(35)}$$

Table 3 contains variations of water saturation and water cut ratios for compressible media at the distance L = 80 m from the injection well.

Table 3

Variations of water saturation and water cut ratios

t, days	Option 2		Option 3		Option 4		Option 5
t, days	s(L, t)	ω	s(L, t)	ω	s(L, t)	ω	s(L, t)	ω
100	0,628	0,013	0,627	0,010	0,627	0,000	0,688	0,675
365	0,633	0,043	0,631	0,031	0,629	0,020
730	0,637	0,069	0,635	0,053	0,633	0,038

The choice of the option for s(r, t) function assignment exerts a significant influence on the water cut ratio. In the fifth option, water saturation ratio is a constant, therefore the water cut does not change, although the radius of two-phase filtration front increases with time.

If the values of capillary pressure gradient are neglected, in formula (34) parameter D = 1, as a result a standard formula is obtained, which coincides with the Buckley-Leverett function. Calculations show that the values of ω, indicator, presented in Table 3, stay practically the same. Hence, if the lower limit for Darcy’s law application is violated, capillary pressure gradient does not exert any influence on the technological indicator – water cut ratio, or on the process of two-phase filtration.

Estimated values, presented in Table 3, depend on the choice of s(r, t) closing equation. In options 2, 4, 5, s(r, t) and injection pressure are interrelated due to a common parameter – the radius of two-phase filtration zone ρ(t), which depends on the coefficients of reservoir compressibility. In option f_3c function equals overbalance pressure at the bottomhole of the injection well.

When solving applied problems of underground hydrodynamics for closed pools, the filtration area is limited by a radius R. It is common to consider a limited filtration area [13], e.g., with constant pressure on the external boundary. Limitation of the filtration area by radius R can be dictated by geological structure of the formation – the distance to the roof in the anticlinal part of the formation or to the bottom in the synclinal part. Boundary conditions imposed on p(r, t) and s(r, t), functions will be different. The filtration velocity at r = R equals zero, water saturation ratio increases proportionally to the B(t) function:

$$ s_1(r,t)=B(t)s(r,R);\ \frac{∂ s_1}{∂ r}=B(t)\frac{∂ s}{∂ r};\ t\ge t_1,B(0)=1. \qquad\tag*{(36)}$$

Unknown function B(t) is determined from equation (18) after integration over the interval from r_c to R. Function s(r, R) is a distribution of water saturation ratio for the time point t₁, when ρ(t₁) = R. For option 2, functions that determine s(r, R) values take the form of: f₁(r, R) f_1c(r_c, R). Time t₁ is determined from formula (19) and, according to Table 3, its value is quite large B(t) parameter for r = L equals:

$$ B(t)=\frac{2k_0k_m^*q}{R^2m_0\mu_{10}\alpha_{s2}f_1(L,R)}t+1. \qquad\tag*{(37)}$$

Total water encroachment ω = 1 for r = L occurs at t = t* и s₁>(L, t*) = s*, therefore B(t*) = s*/s(L, R). From formula (36) it is obtained that t* = 27 days. So, for a closed pool the time of water encroachment is much shorter. A sharp increase in the water cut of a specific production well allows to identify the boundary of the filtration area. In this case D parameter equals:

$$ D=1+\frac{{\mathrm g}(L)LB(t)}{a\Biggl(1-\frac{L^n}{\rho^n}\Biggl)+{\mathrm g}(L)LB(t)}. $$

Calculations show that the value of D parameter varies in the range 0,99-1. In this case, when estimating ω the capillary pressure gradient can be neglected, which means that the developed method is applicable to compressible media that obey classical Darcy’s law.

If under constant injectivity Q overbalance pressure exceeds the breaking strength of the rocks, then man-made fractures and channels of low filtration resistance appear in the formation, which enable water inrush to the bottomholes of production wells. In such case, increasing water cut should be controlled by means of tracer investigations; a method to process their results is developed in paper [4]. In order to prevent formation of low filtration resistance channels it is recommended to change the operation mode of the injection well: the formation should be subject to constant overbalance pressure, which does not exceed breaking strength of the rocks.

Conclusions

1. During plane-radial filtration, capillary pressure gradient depends on the variation law of water saturation ratio s(r, t) in the zone of two-phase filtration, which corresponds to the variation function of water injection pressure. A connecting link between injection pressure and water saturation ratio is the radius of the displacement front.

2. The radius of the two-phase filtration zone during oil displacement by water depends on the variation of water saturation gradient. For a fixed time point of 200 days, its value in the incompressible media is 3-3.5 times greater than the radius in compressible reservoirs.

3. When the lower limit for Darcy’s law application is violated, the influence of capillary pressure gradient on the size of the two-phase filtration zone and the water cut can be neglected.

4. The developed method allows to identify, by a sharp increase of water cut ratio of well products, a boundary of the filtration area for a specific production well, taking into account compressibility of the pore space and the fluid, as well as utilized dependency of water saturation ratio on time and coordinates.

References

1. Baturin A.Yu. Geologic-Engineering Modeling of Oil and Gas Field Development. Moscow: OAO “VNIIOENG”, 2008, p. 116 (in Russian).

2. S.I., Korotenko V.A., Zonova O.P. To the Question of Two-Phase Filtration in Porous Medium. Burenie i neft 2016. N 5, p. 50-54.

3. Kanevskaya R.D. Mathematical Modeling of Hydrodynamic Processes of Hydrocarbon Field Development. Moscow-Izhevsk: Institut kompyuternykh issledovanii, 2002, p. 140 (in Russian).

4. Korotenko V.A., Grachev S.I., Kryakvin A.B. Interpretation of the Tracer Investigation Results Considering Convective Mass Transfer. Journal of Mining Institute. 2019. Vol. 236, p. 185-193. DOI: 10.31897/PMI.2019.2.185

5. Korotenko V.A., Kushakova N.P. Specific Features of Oil Filtration and Displacement from Anomalous Reservoirs. Tyumen, 2018, p. 150 (in Russian).

6. Lysenko V.D. Innovative Development of Oil Fields. Moscow: OOO “Nedra-Biznestsentr”, 2000, p. 516 (in Russian).

7. Mirzadzhanzade A.Kh., Ametov I.M., Kovalev A.G. Physics of Oil and Gas Formations. Moscow-Izhevsk: Institut kompyuternykh issledovanii, 2005, p. 280 (in Russian).

8. Mikhailov N.N. Physical and Hydrodynamic Problems of Residual Oil Recovery from Flooded Formations. Materialy Vserossiiskoi nauchnoi konferentsii, posvyashchennoi 30-letiyu IPNG RAN, 11-13 oktyabrya 2017, Moskva, Rossiya. Moscow: OOO “Analitik”, 2017, p. 110-111 (in Russian).

9. Mikhailov N.N. Hysicogeological Problems in Modeling of Reserves Assessment and Hydrocarbon Reservoir and Production Engineering. Vestnik CKR Rosnedra. 2014. N 6, p. 31-34.

10. Nikolaevskii V.N. Collected Papers. Geomechanics. Vol. 1. Damage and Dilatancy. Oil and Gas. Moscow-Izhevsk: Institut kompyuternykh issledovanii, 2010, p. 640 (in Russian).

11. Zakirov S.N., Indrupskii I.M., Zakirov E.S., Zakirov I.S. et al. New Principles and Technologies of Oil and Gas Field Development. Part II. Moscow-Izhevsk: Institut kompyuternykh issledovanii, 2009, p. 484 (in Russian).

12. Mirzadzhanzade A.Kh., Kuznetsov O.L., Basniev K.S., Aliev Z.S. The Basics of Gas Extraction Technology. Moscow: Nedra, 2003, p. 881 (in Russian).

13. Telkov A.P., Grachev S.I. Hydromechanics of the Formation Applied to Petroleum Industry Problems of Field Development with Inclined and Horizontal Shafts. St. Petersburg: Nauka, 2012, p. 160 (in Russian).

14. Chernykh V.A. Mathematical Hydrogeomechanics of Formations and Wells. Moscow: Neft i gaz, 2012, p. 330 (in Russian).

15. Barenblatt G.I., Patzek T.W., Prostokishin V.M., Silin D.B. Oil deposit in diatomites: a new challenge for subterranean mechanics. Society of Petroleum Engineers Thirteenth Symposium on Oil Recovery, 13-17 April 2001, Tulsa, Oklakhoma, USA, 2002, p. 1-9 DOI: 10.2523/75230-MS

Option	Parameters
Option	s, α_is	∂ s/∂ r
1	\(\ s=s_0+\Delta s\frac{f_1(r,t)}{f_{1c}(t)} \)	\(\ \frac{∂ s}{∂r}=\frac{n\Delta s}{rf_{1c}}\Biggl(1-\frac{r^n}{\rho(t)^n}\Biggl) \)
2	\(\ s=s_0(1+\alpha_{s2}f_1(r,t));\ \alpha_{s2}=\frac{\Delta s}{s_0f_{1c}(r_c,t)} \)	\(\ \frac{∂ s}{∂ r}=\frac{n\Delta s}{rf_{1c}}\Biggl(1-\frac{r^n}{\rho(t)^n}\Biggl) \)
3	\(\ s=s_0\exp(\alpha_{3s}f_3);\ \alpha_{3s}=\frac{1}{f_{3c}}\ln\frac{s^*}{s_0}\)	\(\ \frac{∂ s}{∂r}=\frac{\alpha_{3s}s}{r}a\Biggl(1-\frac{r^n}{\rho^n}\Biggl) \)
4	\(\ s=s_0\exp(\alpha_{4s}f_4);\ \alpha_{4s}=\frac{1}{f_{4c}}\ln\frac{s^*}{s_0}\)	\(\ \frac{∂ s}{∂r}=\frac{\alpha_{4s}s}{r}\Biggl(1-n\frac{r^n}{\rho^n}\Biggl) \)
5	\(\ s_{cp}=\frac{s_0+s^*}{2}\)	\(\ \frac{∂ s}{∂r}=\frac{s_{cp}}{(R-r_{c})} \)

Assessment of the Influence of Water Saturation and Capillary Pressure Gradients on Size Formation of Two-Phase Filtration Zone in Compressed Low-Permeable Reservoir

Abstract

Similar articles