Conducting industrial explosions near gas pipelines
- 1 — Ph.D. Sainr Peterburg mining university ▪ Orcid ▪ Scopus ▪ ResearcherID
- 2 — postgraduate Student Saint Petersburg Mining University ▪ Orcid
- 3 — Ph.D. Saint Petersburg Mining University ▪ Orcid ▪ Scopus ▪ ResearcherID
- 4 — Ph.D. Senior Lectures Sami Shamoon College of Engineering ▪ Orcid
Abstract
The problem to ensure the safety of objects which are in the area of blasting operations, ensuring the destruction of hard rocks, remains relevant. The article presents the results of a large-scale experiment to determine the safe conditions for conducting drilling and blasting operations near the active gas pipeline. The simplest and most reliable way to ensure the safety of the protected object from seismic impact is to reduce the intensity of the seismic wave, which is achieved by changing the parameters of drilling and blasting operations. This requires research to determine the impact of blasting operations on the parameters of seismic waves and the development of methods for measuring these parameters. The paper presents a detailed analysis of the seismic blast wave impact on the displacement of the ground and the model gas pipeline. The features of seismic monitoring during blasting operations near the active gas pipeline are shown. The seismic coefficients and attenuation coefficient of seismic waves are determined. It is proved that the readings of the seismic receivers on the surface and in the depth of the massive differ by two or more times.
Introduction. After the explosion of the explosive charge, shock waves and strain waves begin to propagate through the medium in the form of elastic waves. These waves in the elastic zone are known as seismic blast waves (SBW) [12, 22]. The wave motion propagates from the explosion site in all directions and decreases as the fixed energy spreads over a large mass of material [25, 35]. Despite the fact that the intensity of ground vibrations decreases exponentially with distance, it can remain quite high and cause significant damage to nearby constructions and structures.
As a rule, the construction of an additional branch of the gas pipeline is carried out at a distance of 15-20 m from the existing main line [4], which is due to a reduction in capital costs. In areas where the projected trench is laid in rocks, there is no alternative to the explosive method of drifting. When conducting drilling and blasting operations (DBO) in cramped conditions, there is a risk of negative impact on the protected pipeline by a seismic blast wave, shock-air waves and scattering of blasted rock pieces of the exploded rock mass [13, 23, 29]. In practice, the shock-air wave and fragment distribution are minimized or completely eliminated [39, 40, 41], using effective methods of protection – various types of shelters
The seismic impact of the explosion on the gas pipeline laid in the rocks is reduced only by changing the DBO parameters, namely, the mass of the simultaneously exploding charge, the deceleration stage and the deceleration interval itself. Therefore, studies aimed at determining the impact of blasting operations on the parameters of seismic waves are relevant [3, 31].
Formulation of the problem. All studies related to SBW can be divided into four main areas: investigation of the SBW impact on nearby structures and identification of damage criteria; prediction of SBW at the observation point; reducing the level of negative impact of SBW at the observation point; determination of the impact of various parameters on the level of SBW.
Investigation of the SBW impact on nearby structures and identification of damage criteria. In recent decades, many damage criteria have been identified, defined, and studied. The first stud-ies on the damage criteria were conducted in 1927 by H.M.Rockwell and became known as the Rockwell energy formula [8]. H.Nicholls [20] summarized the results of previous surveys and conducted additional studies to establish the rela-tionship between the level of SBW and the amount of damage to buildings. After studying the reac-tion of structures and the magnitude of their damage as a result of the impact of SBW, D.E.Siskind plotted a schedule for assessing the possibility of safe drilling and blasting operations, taking into account the peak velocity of ground particles vibration and the frequency of vibrations, known as the standard of the US Bureau of Mines (USBM, abolished in 1996) [20, 21]. Their publications also present the results of work on determining the damage criteria. In 1983, the US Office of Open Mining (OSM) published regulations for the control of ground vibrations (the OSM instruction differs from the USBM rules). In 1986, safety standards (DIN) for blasting opera-tions were published [34]. The USB, OSM, and DIN standards are applicable only if the peak ground vibration velocity and the frequency of vibrations at the observation point are known.
Prediction of SBW at the observation point. Some researchers have done work on predicting the SBW amplitude. These studies can be divided into two groups: scaled distance modeling (SD) [2, 10, 15] and simulation modeling [28, 30, 32], which includes the finite element method (FEM) and artificial neural network (ANN) models [19, 27].
SD-modeling. According to the majority of researchers, the peak velocity of ground particles vibration in SBW is a function of the scaled distance, i.e., it relates the distance before the explosion to the mass of explo-sives at the deceleration stage. The most widely used equation of the peak velocity of particle vibra-tion, created by USBM [8]:
where SD (Scaled Distance) – scaled distance, m/kg1/2; K – additional coefficient; В – additional factor.
Many researchers [28, 36], based on these dependencies, also proposed modified equations for predicting the peak velocity of particle vibration at the observation point. For example, in Russia, an analog of this dependence is the equation of the peak velocity of particle vibration, known as the M.A.Sadovskiy equation [6]:
The proposed equations are based on statistical dependencies. To determine the additional co-efficients using multiple regression analysis, field tests should be conducted. Many studies have been published to determine specific coefficients for SBW management [17, 25, 26, 38].
Artificial neural network modeling (ANN modeling). A number of researchers have tried to predict ground vibrations using an artificial neural network that includes a large number of parameters [7, 22, 33]. To predict the PPV and the vibration frequency, the diameter, length and number of wells, the bottom hole, the charge in the deceleration stage, and the horizontal and radial distances were taken into account. The authors affirm that the ANN models provide better PPV prediction compared to other methods.
Finite Element Method (FEM): some researchers [18, 37, 39] have developed a FEM model for predicting PPV. In their model, they tried to simulate the factors that affect vibration. Thus, artificial vibrations were created to analyze real complex waves.
Reducing the level of negative impact of SBW at the observation point. To eliminate or reduce the negative impact of SBW at the point of observation, a huge number of studies have been conducted. The aim in one of the research areas was to reduce SBW at the site of origin by changing the number, type of explosives, geometry of blast wells, etc. Another direction was devoted to reducing the level of vibrations at the observation point by creating artificially con-centrated inhomogeneities between the seat of the blast and the observation point – such as cutting the ground, a barrier from wells, trenches. In the first group, scaled distance modeling was used to determine the additional coefficients. After determining the additional coefficients for a specific area, the number of explosives in the deceleration stage that meets the conditions for safe DBO management was calculated [17, 24, 38].
Studies with the use of artificial dissections, barriers from wells, trenches [11, 14] between the seat of the blast and the observation point in DBO have shown that these artificial voids behave as a barrier before the blast wave and reduce the level of SBW [9].
Determination of the impact of various parameters on the level of SBW. The intensity of ground vibrations depends on various parameters. These parameters can be divided into controlled and uncontrolled [13, 35]. The controlled parameters are related to the design of the DBO and the blast characteristics of the explosives. Uncontrolled parameters include the properties of geological structures and rock characteristics, which are taken into account in additional coefficients, and their influence on the PPV is determined by regression analysis.
The generalized results of studying a group of parameters that affect the PPV [20, 22], showed that the levels of ground vibration near the seat of the blast and at the observation point differ, and the PPV near the seat affects the PPV at the observation point. These studies have shown a relationship between the DBO parameters that affect SBW. The main dependencies between the parameters of ground vibrations are presented in the form of a relation diagram [16]. It follows from the diagram that the main influence on the ground vibrations near the seat of the blast are the amount of one-time blasted explosives, the line of least resistance (LLR) and the bottom hole. The main influence on the intensity at the observation point is provided by the PPV near the seat of the blast and the geological structures located between the seat of the blast and the ob-servation point. Such parameters as the rock characteristic and the distance between two points cannot be changed. Geologic structures also cannot be changed, but artificial voids created in the path of SBW progress can reduce the level of its impact. In this case, the best way to reduce the level of SBW impact at the observation point is to reduce the PPV near the seat of the blast, or to create artificial voids between the seat of the blast and the observation point.
Methodology. The value of the ground displacement velocity at the base of the protected object is a generally accepted criterion for assessing the seismic hazard of an explosion. Thus, a report from the U.S. Bureau of Mines, based on decades of research, shows that residential damage from blasting is more closely correlated with the resulting ground displacement velocity than with acceleration or displacement.
In the Russian Federation, the safety criterion is considered to be the permissible vector velocity or the permissible total velocity for three components. In the USA, such criteria are the permissible vector energy and total energy. However, the quantitative parameters in both Russia and the USA are in the same range of values: for industrial buildings and structures it is 1-12 cm/s (for the United States, the permissible velocity at which a safe level of seismic energy is provided is 3-12 cm/s) [4, 7]. The vector velocity and energy are calculated accordingly:
where x, y, and z are the radial, tangential, and vertical components, respectively.
When choosing permissible velocities in continuous media, the general assessment of the rocks deformation properties is of great importance. In such conditions, compression and unloading of rocks occur elastically, i.e. according to the same law without residual deformation, with a signifi-cant prevalence of the effective stress over the structural strength
The relationship between the dependence of the ground vibration rate on the elastic-plastic properties of rocks and their relative deformation [5] is described by the formula:
where
The permissible deformations at the base of the protected object are determined by the classifi-cation of the structure, the service life and the importance of the object [1]. The critical vibration velocity can be defined as the two permissible velocities V0.
The determination of the quantitative parameters of the seismic effect of an explosion under certain mining and geological conditions is based on the solution of the equality of the permissible velocity and the velocity that occurs in the medium when a charge of mass Q explodes at a distance R from the structure [6], according to the formula (2). The seismic vibration velocity under certain mining and geological conditions can be determined experimentally, using special seismic studies.
Permissible displacement velocities are determined for most structures and rock types [6]. In the case of pipelines, these displacements can only be estimated approximately by calculation methods.
ОThe permissible displacement velocity at the base of the pipeline determined in this way does not give the exact values of the resulting seismic explosion wave. This is due to various factors that affect the nature of the distribution of seismic vibrations. In this regard, there is a need to constantly monitor the parameters of the seismic blast wave (velocity, acceleration and displacement) in the rock using instrumental methods. Seismic monitoring in the vicinity of a specially protected object is carried out after determining the parameters of the environment (the seismicity and attenuation coefficients).The need for this kind of monitoring is due to a possible violation of the conducting blasting operations technology: violation of the installation scheme of the explosive network, overes-timation of the charge mass at the deceleration stage, underestimation of the established intervals between the deceleration stages.
When conducting such monitoring of underground pipelines, there are features that must be taken into account when developing a measurement methodology. The research given in the article is devoted to the identification and consideration of these characteristics.
Research method. A full-scale experiment was performed to calculate the attenuation coefficient n, which determines the attenuation parameters of the ground displacement velocity in a seismic explosion wave and to determine the parameters of the seismic explosion wave that came directly to the gas pipeline walls. The created model gas pipeline in the process of experimental blasts carried out in the close vicinity, took on seismic waves.
The main purpose of the experiment was to obtain data on the ground vibrations attenuation in SBW with distance and to determine the correction coefficient for monitoring DBO with the sur-face placement of the sensor.
The model was a piece of pipeline with a length of 11.6 m, a diameter of 1.22 m, a weight of 6.2 tons and a wall thickness of 17.8 mm, the material of the design is steel of strength class K60. This piece was laid in a trench that exactly repeats the parameters of the trench for the main pipeline and meets all the requirements of the current norms and standards used in the construction of underground gas pipelines. One side of the model trench was chosen in a zone of strongly fractured granites overlying almost monolithic granites. The other side of the trench was created by pouring sand and sandy loam to ensure the predominant distribution of seismic blast waves along the border of monolithic granites (Fig.1, a).

1 – well; 2 – monolithic granites; 3 – fractured granites; 4 – sand, sandy loam; 5 – dropout (sand cushion); 6 – measurement points (in front of the pipe, in the pipe, behind the pipe)

1 – explosive bore: depth of 2 m, the charge mass of 1 kg, explosive – Nitronit PAS 60, shelter – sand H of 1.5 m; 2 – surface sensors; 3 – deepened sensors; 4 – sensor number
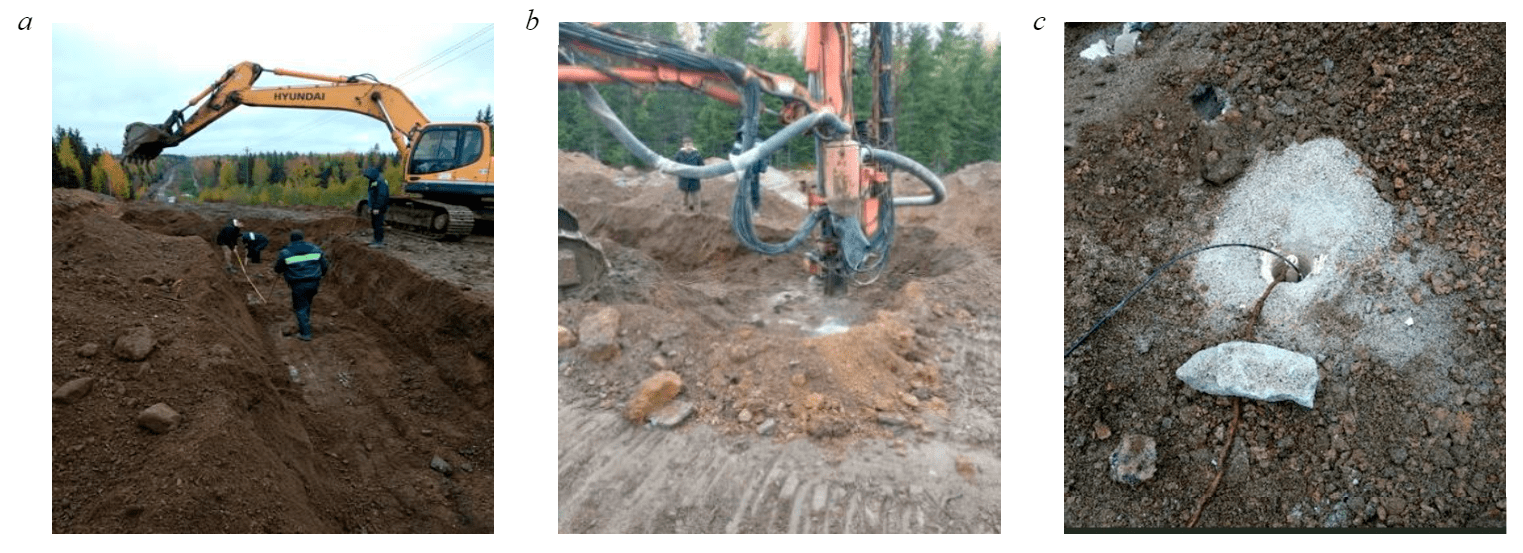
The borehole charges were located at a distance from the model pipe in accordance with the scheme shown in Fig.1, b.
In similar mining and geological conditions, during the reconstruction of the North European gas pipeline, the second experiment was conducted to determine the seismic coefficient and the attenuation coefficient, as well as to determine the correlation between the amplitude parameters of the seismic explosion wave and the depth of the signal registration. For this purpose, five explosions of single explosive charges weighing 1 kg were organized, the distance between the exploding wells was 3 m, the maximum distance to the sensors was 25 m, and the minimum was 13 m (Fig.2).The process of preparing the experiment is shown in Fig.3.

1 – calculated value of the seismic vibration velocity using the values of the seismic coefficients K = 542 and attenuation n = 2.2; 2 – experimental values of the seismic vibration velocity
For measurements, the Blastmate III and Minimate Plus recording devices were used, as well as the Zet 048-C N 2156 seismic station, and single-component piezosensors were downed into the measuring wells.
The approximate values of the seismicity coefficient Ks = 546 and the attenuation coefficient n = 2.4 obtained during the monitoring of the North European gas pipeline construction in the Vyborg district of the Leningrad region were verified and recalculated for the conditions of this construction.
Results. The results of measuring the displacement velocities during the first experiment at three recording points (T1, T2, T3) from four explosions are shown in Table 1.
The attenuation coefficient of the seismic blast wave for these mining and geological conditions was calculated from the experimental results (Table 1).
The analysis of the results showed that the value of the total velocity vector on the surface (T1) is at least three times higher than the velocity in the pipe (T2).
Based on the fact that during the first explosion, SBW distribution occurred in undamaged rock, and the attenuation coefficient value was minimal, to assess the seismic effects on the deepened object, the results of SBW impact measurements obtained with ground-based sensors should be reduced by three times.
Table 1
Displacement velocity distribution
| Explosion number | Total weight of explosives, kg | Т1 (before the pipe) | Т2 (in the pipe) | Т3 (behind the pipe) | ||||||
| vcalc, sm/s | vm, sm/s | R, m | vcalc, sm/s | vm, sm/s | R, m | vcalc, sm/s | vm, sm/s | R, m | ||
| 1 | 86,4 | 8,15 | 2,21 | 15,9 | 4,23 | 4,05 | 20,9 | 2,07 | 2,94 | 24,9 |
| 2 | 14,4 | 17,5 | 1,83 | 10,2 | 6,72 | 6,02 | 15,2 | 3,83 | 4,61 | 19,2 |
| 3 | 32,5 | 37,0 | 3,64 | 9,8 | 13,7 | 9,97 | 14,8 | 7,73 | 6,32 | 18,8 |
| 4 | 14,4 | 49,8 | >25,4 | 6,6 | 12,9 | 4,90 | 11,6 | 6,31 | 2,67 | 15,6 |
Notes: vcalc – – the calculated value of the ground displacement velocity, calculated by the formula (2); vm – the measured value of the ground displacement velocity; R – – the distance from the sensor to the corresponding explosion.
The analysis of the second experiment results (Fig.4) showed a good convergence of the experimental and calculated data at the values of the attenuation and seismicity coefficients of 2.2 and 542, respectively (Table 2).
Measurement of the vibrational acceleration of rock mass particles depending on the depth of the sensors (see Fig.2, b) showed that the value of the reducing coefficient for accelerations recorded on the surface varies from 2 to 4 (Table 3).
Table 2
Velocity distribution
| Distance to the sensor, m | Vibration velocity, mm/s | Error value, % | |
| experimental | calculated | ||
| 13 | 19,8 | 19,20 | 3 |
| 16 | 9,39 | 12,16 | 22 |
| 17 | 9,6 | 10,64 | 10 |
| 19 | 9,3 | 8,33 | 10 |
| 20 | 6,9 | 7,44 | 7 |
| 22 | 6 | 6,03 | 1 |
| 25 | 3,9 | 4,56 | 14 |
Table 3
Distribution of accelerations of rock mass particle vibrations
| Sensor number | Depth, m | Acceleration value, g |
| 1 | 2 | 0,12 |
| 2 | 2 | 0,13 |
| 3 | 1 | 0,22 |
| 4 | 1 | 0,26 |
| 5 | 0 | 0,49 |
Discussion. The results of measurements on the unbroken granite rock mass and in the pipe correspond to the results of calculations performed according to the formula (2), which is calculated to ensure seismic safety in the project of drilling and blasting operations on the 2nd line of the North European gas pipeline construction (Table 1, T2, T3).
The results of measurements on the broken granite rock mass sharply differ in a smaller direction (about an order of magnitude) from the calculated values (Table 1, T1).
The coincidence of the calculated and measured values of the velocities in the pipe and on the granite rock mass is explained by the fact that the steel pipe weighing 6.2 tons was laid on a sand cushion 250 mm thick, which, in turn, was laid on an unbroken rock mass. It can be assumed that the steel pipe pushed through the sand cushion, which created a contact of steel with the granite rock mass. A similar situation can very rarely occur during the gas pipeline construction, since the sand cushion will necessarily be located on the broken granite rock mass after the explosions that are used for the trench construction. Thus, the seismic waves that come the active gas pipeline line will be attenuated in approximately the same way as it was recorded at the measurement point T1.This is due to the fact that around the built trench with the help of explosions, a fairly powerful layer of destroyed mass of eroded rocks is formed
The results suggest that the measured parameters of seismic waves during monitoring directly at the existing pipeline will be incorrect, not reflecting their true values on the pipe wall. Therefore, measurements when monitoring the parameters of seismic and explosive waves should be carried out at a distance of no closer than 5 m from the axis of the existing gas pipeline in the direction of the explosion
Based on the measured values of the ground displacement velocities during monitoring, the maximum permissible ground displacement velocity of 20 cm/s should be assumed, calculated according to the method based on the tensile strength of the pipe. Therefore, at a distance of 10 m from the blast site, the displacement velocity recorded on the surface should not exceed 40 cm/s. Based on this, the ratio between the measured surface displacement velocity and the ground displacement velocity at the location of the pipe of the protected gas pipeline should be taken equal two.
Conclusions. A physical model of the active gas pipeline has been created: a piece of steel pipe is placed in a trench of standard dimensions, passed through the rock, so the real conditions for laying pipelines were modeled. The explosive loading modeling of the experimental pipe was carried out in the conditions of the landfill, which meets the characteristics of the conditions of laying the gas pipeline under construction and provides the most severe seismic impact on the pipe.
SBW parameters were measured at three points: in front of the pipe, in the pipe, and behind the pipe. In addition, to clarify and test the results of the model experiment during the gas pipeline reconstruction, an industrial experiment was conducted under real conditions. SBW parameters were measured at different distances from the blast site. At the same time, SBW parameters were measured at the surface, at a depth of 1 and 2 m. Data on the ground vibrations attenuation with distance were obtained and correction factors were determined for defining SBW parameters at depths of 1 and 2 m from the measured values of these parameters obtained on the surface.
Analysis of the results of the seismic impact of explosions on deep objects allows to draw the following conclusions:
• installation of seismic sensors should be carried out at a distance of no closer than 5 m from the object;
• the velocity (acceleration) of ground vibrations at a depth of 2 m is three times less than the velocity (acceleration) of ground vibrations at the surface;
• the seismic and attenuation coefficients should be determined experimentally from the data of previously conducted explosions.
The seismic monitoring of large-scale explosions carried out according to this method when conducting blasting operations in the close vicinity of deep gas pipelines guarantees compliance with the conditions that ensure the safety of these objects from the seismic impact of the explosion.


