Sorption of rare earth coordination compounds

Introduction. Rare earth metals (REMs) are an integral part of many high-technology industrial sectors, such as laser technology, military equipment, pharmacy, electronics, metallurgy and automobile industry.

As a result of REM market analysis, a conclusion can be drawn that in 2014-2017 REM extraction rate was relatively stable, and starting from 2018 it dramatically increased from 132 to 170 thousand tons [3]. In terms of resources, the key leader of the last decade still is China with a market share of 37.89 %, Brazil and Vietnam follow with equal shares of 18.94 %. Russia is also not the last country on this list with a share of 10.33 %. Other countries worth mentioning are Australia, the USA, Malaysia and India, which also have estimated REM reserves [3].

Presently Russia does not produce but rather imports rare earth elements (REEs), which negatively affects the economy of the country and its industrial sector in particular.

The main target source of REEs in Russia is located in Murmansk oblast, at Lovozero deposit. There is also associated extraction of REMs as a component of other ores and waste products [6, 15, 16]. However, in Russia there are no deep processing facilities, so it impossible to obtain the final product in the form of individual REMs. As a result, extracted REMs are exported as cheap collective concentrates.

The main methods of REE recovery are precipitation, extraction, and sorption [5, 8, 12, 13, 18]. Sorption processes are the most promising method of selective REM recovery from multisalt solutions, which allows to separate elements from their associated components, as well as to separate REMs with similar chemical properties [10].

Authors of paper [7] studied recovery of Nd, Gd and Ho on synthesized resins, the functional group of which is represented by primary amine surface groups with REM-binding ligands: phosphonoacetic acid (PAA), N, N-bis (phosphonomethyl) glycine (BPG) and diethylenetriaminepentaacetic dianhydride (DTPADA). In order to estimate thermodynamic characteristics, a series of tests was performed with solutions, containing 0.3-300 mg/l of REEs at constant HCl concentration of 0.5 mol/l and a fixed pH value. Solutions were mixed at 300 rpm for 24 hours with ion exchange resin (10 g/l) at three temperatures: 20, 60 and 100°С. Sorption isotherms were obtained in constant presence of NaCl (0.5 mol/l) and fixed pH values, dependent on synthesized anion resin: 6.5 for amine, PAA, BPG or 2.5 for DTPADA, at temperatures 20, 60 and 100 °С. Thermodynamic description was made using Langmiur and Freundlich models. As a result, the authors calculated values of equilibrium constants, Gibbs free energy, enthalpy and entropy of Nd, Gd and Ho extraction.

In paper [14] the authors examined separation of metals based on ion exchange displacement of metal ions (Se, Re, Mo, La, Sc, Ce (IV)) from the solid phase on ion exchange resins Purolite A170, Lewatit M500 and Lewatit SP112. Effective separation of cerium (IV) and lanthanum was observed on Lewatit MonoPlus SP 112 (Na⁺). Ion exchanger saturation was conducted under dynamic conditions with a lanthanum nitrate solution. Then elution with cerium (IV) nitrate solution was carried out. In the course of experiment, it was observed that cerium (IV) displaced 96-97 % of lanthanum from the solid phase of the resin. The process of frontal-gradient displacement of lanthanum by cerium (IV) was a part of the developed technology. Total coefficient of cerium and lanthanum separation using designed extraction method was 4300. Distribution coefficients for cerium and lanthanum were 1000 and 0.23, respectively. This method allows to extract cerium (IV) with the purity of 99.9 %.

Likewise, for extraction of such metals as thorium (IV) and uranium (IV) in the study [17] authors used solid sorbents with a resin matrix in the form of monomer 2, 4, 6-triformylphloroglucine and 2,5-diaminobenzenesulfonic acid (COF) and functional groups COF-SO₃H and \(\mathrm{[NH_4]^+[COF-SO_3^-]}\). The paper estimates the impact of pH on thorium (IV) sorption capacity in the interval 1-2.8. A conclusion was drawn that for sorbent \(\mathrm{[NH_4]^+[COF-SO_3^-]}\) with the growth of pH its capacity increased from 98.8 (рН = 1) to 168 mg/g (рН = 2.8). A similar tendency was observed for sorbent COFSO₃H, however, a decrease in pH level resulted in declining capacity values. Research on kinetic dependencies demonstrated that within 10 minutes of the experiment capacity value reached 232 mg/g from the solution with thorium (IV) concentration of 125 ppm at pH = 2.8 on the sorbent \(\mathrm{[NH_4]^+[COF-SO_3^-]}\) and 100 mg/g on the sorbent COF-SO₃H. Sorption isotherms obtained in the interval of concentrations 50-400 ppm were analyzed using Langmuir model. Resulting capacity value was higher for sorbent \(\mathrm{[NH_4]^+[COF-SO_3^-]}\) than it was for COF-SO₃H. The authors separated thorium (IV), uranium (IV), cerium (III) and europium (III) and obtained the following coefficients of element separation: S_Th/U = 9,4, S_Th/Eu = 9,4 и S_Th/Ce = 10,3.

Problem statement. Analysis of literature references allows to come to a conclusion that REM recovery from multisalt systems [11] is a relevant topic due to the complex character of REM sources. Values of obtained thermodynamic characteristics of the sorption process, namely of ion exchange, define not only conditions of running the process but also technological parameters of ion exchangers for REE separation and removal of associated components. Basing on Gibbs free energy of ion exchange process, it is possible to predict the efficiency of lanthanide recovery from aqueous salt solutions. Taking into account that REE recovery is usually performed from solutions of mineral inorganic acids, used as leaching solutions for phosphogypsum or other REM-containing materials, the paper focuses on ytterbium recovery in the form of anionic complexes with Trilon B on weakly basic anion exchange resin D-403 from nitric acid solutions.

Experiment methodology. Static sorption examination. Examination of REE sorption was carried out from model solutions, containing anion complexes of ytterbium with Trilon B (EDTA ytterbiate ions), obtained by adding Trilon B to ytterbium nitrate solution with a precise mole ratio of 1:1 in accordance with the reaction stoichiometry [9].

Variable concentrations method was used at L/S ratio = 5 (solution – 20, anion resin – 4 cm³), рН = 3 and constant ionic strength I, equal to 1 mol/kg (NaNO₃). Sorption was performed at temperatures T, equal to 298 and 343 K in the interval of ytterbium concentrations 0.1082-0.0056 mol/kg.

As an ion exchange resin, the authors utilized anionite D-403 in a nitrate form (China), which is a polystyrene chelate anionite with a divinylbenzene-styrol matrix and a functional group in the form of tertiary nitrogen atom with oxyhydrilic groups in positions β, γ and δ, which decrease the mobility of a lone-pair nitrogen electrons as a result of negative inductive effect [9]:

The solution and anionite were mixed at the rate of 120 rpm in a GFL thermostated cabinet (Germany) for 5-6 h until it reached equilibrium. Ytterbium concentration in the initial and equilibrium solutions was measured using spectrophotometric method with arsenazo (III) and X-ray fluorescence method on Epsilon 3 spectrometer (produced by PANalytical).

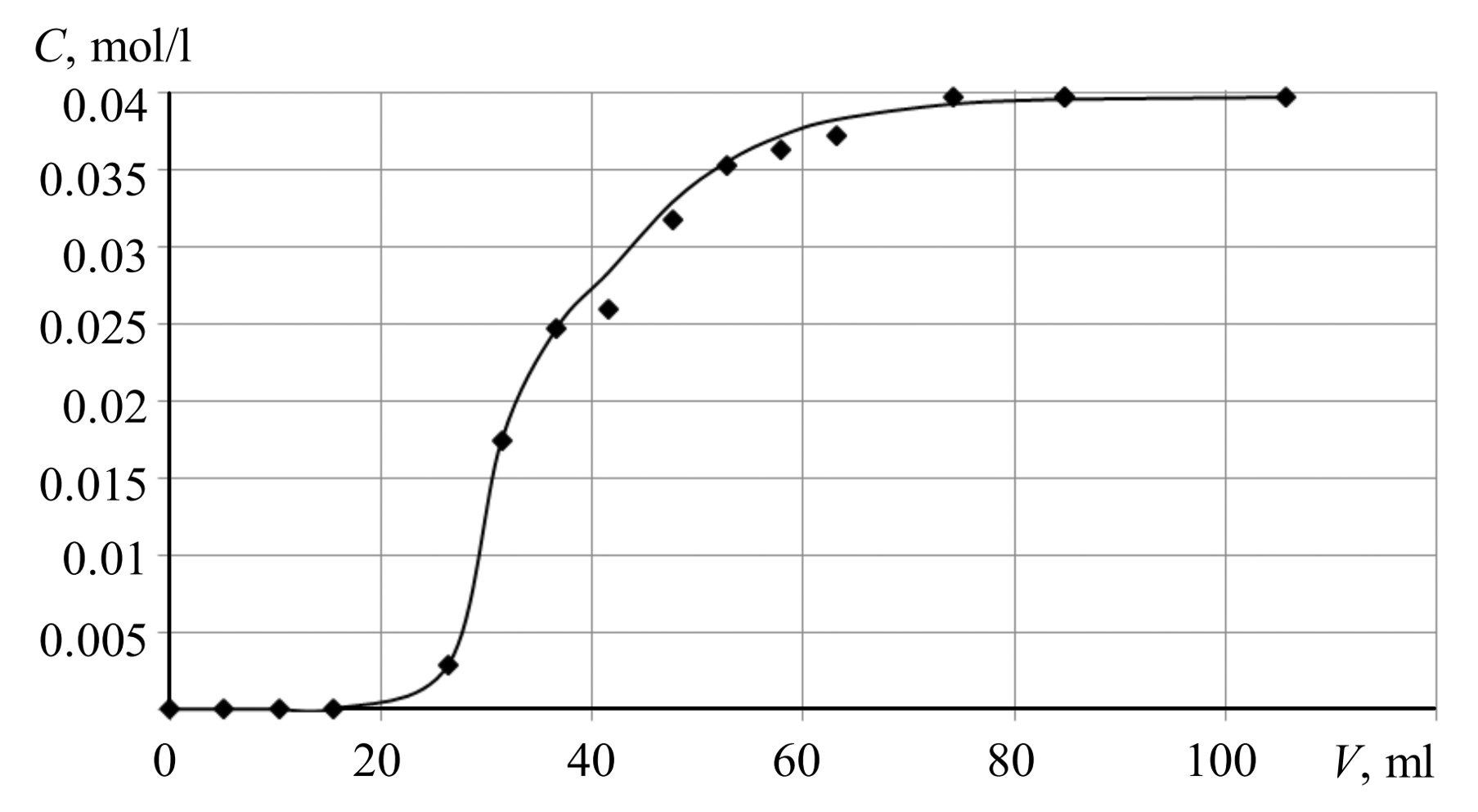
Dynamic sorption examination. In order to confirm the values of limiting sorption, saturation of D-403 anionite with EDTA ytterbiate ions was performed under dynamic conditions. The experiment took place in a sorption column with diameter of 1.2 cm and anionite layer height of 31.5 cm, the mass of anionite was 11.3 g. Solution containing 0.0398 mol/l of EDTA ytterbiate ions was passed through the column at a rate of 1 ml/min using peristaltic pump LOIP LS-301 (JSC “Laboratory Equipment & Instruments”, Russia). On column exit the solution was sampled by 5 ml until the breakthrough and by 10 ml after the breakthrough until the initial concentration was reached.

Experiment results. Estimation of equilibrium constant, Gibbs free energy of ion exchange and total anionite capacity. Data obtained in the process of sorption at temperatures 298 and 343 K are presented in Table 1. Sorption value was calculated using formula

where \(C\)₀ и \(C\)_∞ are initial and equilibrium concentrations of complex ions in the solution, mol/kg; \(V\) is volume, ml; m is dry anionite mass, g.

Table 1

Experimental data on the sorption of EDTA ytterbiate ions on D-403 anionite in nitrate form under рН = 3

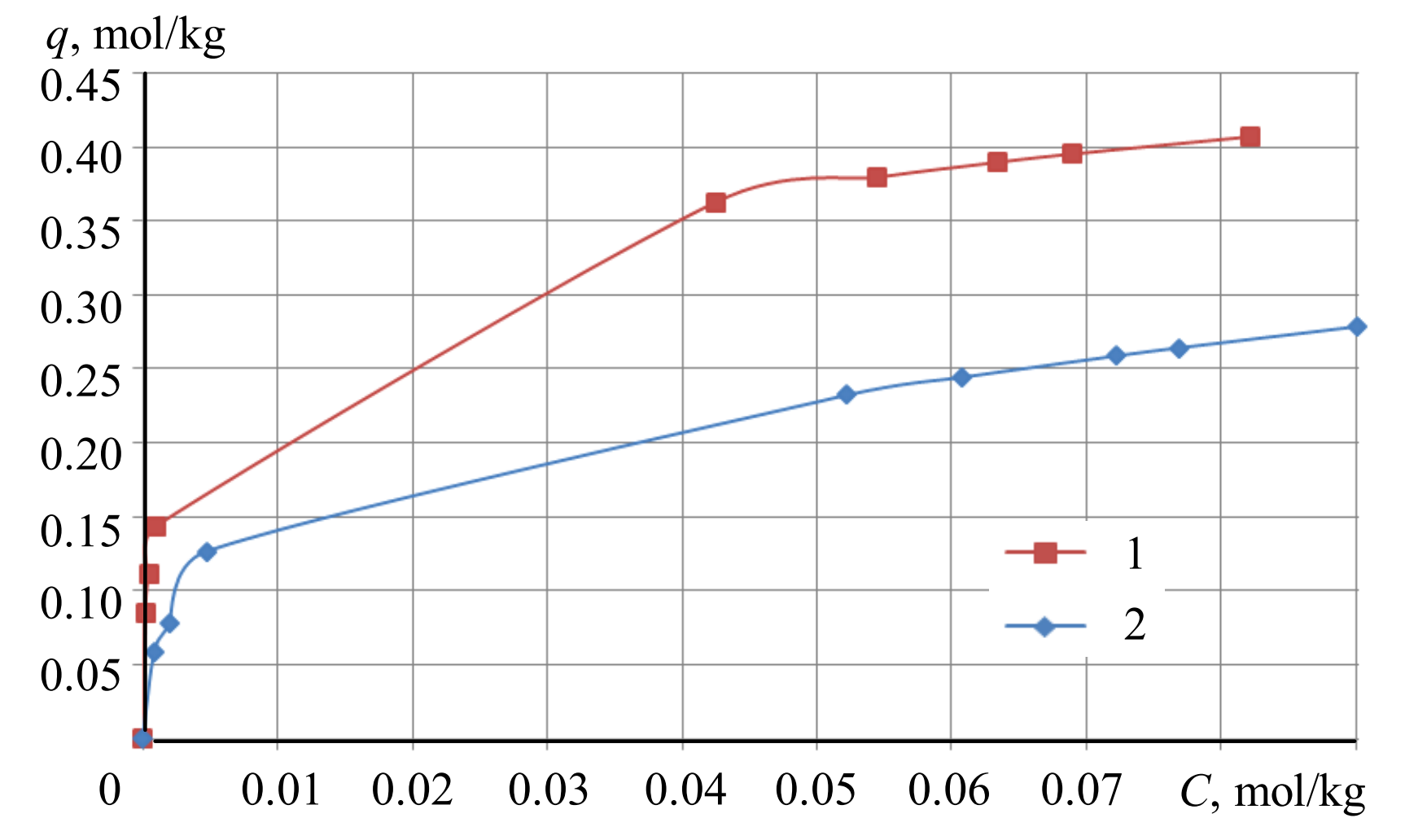
Sorption isotherms are presented in Fig.1.

In order to derive thermodynamic data, authors mathematically transformed the mass action law,

used in ion exchange equation

where \(K\) is the constant of ion exchange equilibrium; \(\Large{\mathrm{\Gamma_{YbTr^–}}}\), \(\Large{\mathrm{\Gamma_{{NO^3}^{–}}}}\) – are values of ion concentration in solid phase of the anionite, mol/kg; \(\Large{\mathrm{a_{{NO^3}^{–}}}}\), \(\Large{\mathrm{{a_{YbTr^-}}}}\) indicate activity of nitrate and EDTA ytterbiate ions, mol/kg; \(\mathrm{[NO{_3^-}]}\), \(\mathrm{[YbTr^-]}\) are concentrations of nitrate and EDTA ytterbiate ions, mol/kg; \(\Large{\mathrm{\gamma_{[NO{_3^-}]}}}\), \(\Large{\mathrm{\gamma_{[YbTr{_3^-}]}}}\) are activity coefficients of nitrate and EDTA ytterbiate ions; \(R\) is organic matrix of ion exchanger.

Assuming that limiting sorption is composed of equilibrium sorption values of nitrate and EDTA ytterbium ions:

the mass action law can be converted to linear form, similar to Langmuir sorption isotherm

where \(\Large{f(C)=\frac{\mathrm{[NO{_3^-}]}\gamma{_{\pm\mathrm{NaNO_3}}^2}}{[\mathrm{YbTr^-}]\gamma{_{\pm\mathrm{NaYbTr}}^2}}}\) is x-axis of concentration \(\Large{\frac{1}{\Gamma_{\mathrm{YbTr^-}}}}\) is y-axis of inverse sorption, \(\Large{\gamma{_{\pm}}}\) are average ion coefficients of electrolyte activity, expressed through activity coefficients of individual ions:

Average ion activity coefficients were estimated using reference data and mathematical description of dependence between activity coefficients and ionic strength of the solutions. Under given ionic strength, for \(\mathrm{NaYbTr}\) compound, γ± was considered equal to CH₃COONa due to strong dependency of activity coefficients upon ion charge and ionic strength of the solution, as well as weak dependency on anion individual character, including the complex ion. It is established that complex ions do not demonstrate any specific features in terms of activity coefficients as compared to simple electrolytes [4].

Taking into account the impact of temperature, activity coefficients were re-estimated using formula

where γ_±,343 and γ_±,298 are values of average ion activity coefficients at respective temperatures; A3₃₄₃ and А₂₉₈ are coefficients of Debye – Hückel equation for temperatures 343 and 298 K, respectively (А₃₄₃ = 0,5562, А₂₉₈ = 0,5107 [1]).

Calculated and experimentally obtained data for mathematical description of linear-form sorption isotherms of ytterbium on D-403 anionite from nitrate solutions at temperatures 298 and 343 K are presented in Table 2.

Table 2

Data for plotting linear-form sorption isotherms of EDTA ytterbiate ions on anionite

Linear forms of sorption isotherms are presented in Fig.2.

Using equations of linear form approximation (Fig.2), values for equilibrium constants, and Gibbs free energy, total anionite capacity were calculated, as well as limiting sorption of a complex EDTA ytterbiate ion (Fig.3).

Thermodynamic and sorption characteristics are presented below:

Estimation of sorption enthalpy and entropy. Basing on obtained values of equilibrium constants in the temperature interval 298-343 K, enthalpy of ion exchange was calculated using isobar equation

where Т₁ and Т₂ are temperatures of sorption process, К₁ and К₂ are constants of ion exchange equilibrium at respective temperatures. Likewise, ion exchange entropy was estimated:

The following values were obtained:

Estimation of total dynamic exchange capacity (TDEC) and dynamic exchange capacity (DEC). According to results, presented in Fig.2, limiting sorption of a complex ion under static conditions at 298 K was estimated at 0.279 ± 0.014 mol/kg. Sorption curve for dynamic conditions is presented in Fig.3.

TDEC and DEC values were calculated using formulas:

where m is sorbent mass, g; С₀ and С_n are initial and current cerium concentrations on column exit, mg/ml; V_n+1, V_n are volumes of solution, corresponding to cerium concentrations on column exit С_n+1 и С_n, мл; С_br is the volume of solution before the breakthrough, ml.

TDEC and DEC were estimated at 0.12 ± 0,01 and 0,09 ± 0,01 mol/kg, respectively.

Discussion of experimental results. According to calculated Gibbs free energy of ion exchange at temperature 298 K, EDTA ytterbiate ions have greater chemical affinity to the solid phase of ion exchange resin than light-group REEs:

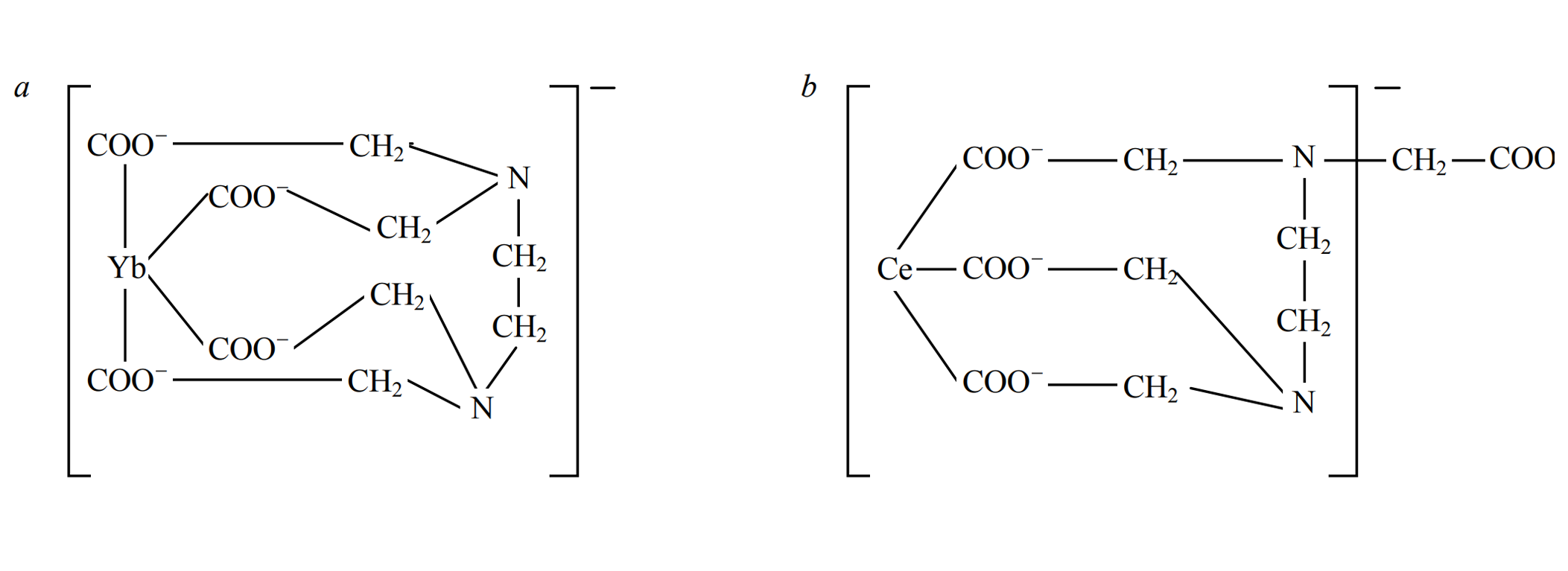
It happens due to the specific character of ligand coordination against REE ion of light and heavy groups [2]. The inner sphere of [YbTr]^– and [ErTr]^– complexes is composed of two nitrogen atoms and four carboxyl groups, whereas [CeTr]^– and [YTr]^– complexes contain two nitrogen atoms and only three carboxyl groups (Fig.4). An increase in effective negative charge of complex erbium and ytterbium ions leads to an increase in electrostatic interaction with the matrix of ion exchange resin. As a result, with diminishing atomic radius and elevating effective charge of the complex, chemical affinity to the solid phase of anion exchange resin increases.

Decreasing Gibbs free energy in the array ‘cerium-erbium-ytterbium’ correlates with lanthanide contraction, diminishing atomic radius and elevating effective charge of the complex, which leads to greater affinity, intensification of electrostatic interaction with anion exchange resin and higher selectivity.

Limiting sorption value of complex ion agrees with the values of total anionite capacity at both temperatures. With rising temperature the sorption value increases, as the equilibrium shifts in the direction of nitrate ion displacement from the matrix of ion exchange resin by EDTA ytterbiate ions.

Enthalpy value is positive, but the defining factor of decreasing Gibbs energy \({\Delta{G_{T}^{0}}}\lt0\) in the course of ion exchange with rising temperature is an entropy criterion. Obtained thermodynamic data characterize selectivity of ion exchange between EDTA ytterbiate ions in the REE array and \(\mathrm{{NO_{3}^{-}}}\) on the surface of ion exchanger. The fact that complex ion sorption does not reach the value of limiting sorption is explained by the large geometric size of EDTA ytterbiate ion.

Conclusion. The paper examined ytterbium sorption in the form of anionic complexes with Trilon B from nitrate solutions at constant ionic strength of 1 mol/kg, рН = 3, L/S ratio =5 and temperatures 298 and 343 K on weakly basic anion exchange resin D-403.

Obtained values of limiting sorption of complex EDTA ytterbiate ion equal 0.28 ± 0,01 at temperature 298 K and 0.41 ± 0,02 mol/kg at 343 K under static conditions, TDEC = 0.12 ± 0,01 and DEC = 0.09 ± 0,01 mol/kg under dynamic conditions.

Estimations were made for total anionite capacity at temperatures 298 and 343 К: Г_∞ = 0,27 ± 0,01 and Г_∞ = 0,38 ± 0,02 mol/kg, respectively.

Mathematical transformation of mass action law was performed, as it was converted to a linear form similar to Langmuir equation, the graphic solution of which allowed to calculate thermodynamic parameters of ion exchange process.

Equilibrium constants and Gibbs free energy of ion exchange at temperatures 298 and 343 K were K₂₉₈ = 103,02 ± 5,15; \(\Delta_r{G_{298}^{0}}\) = 11,45 kJ/mol; K₃₄₃ = 448,69±22,43 and \(\Delta_r{G_{343}^{0}}\) = –17,42±8,71 kJ/mol.

Using Gibbs free energy values, enthalpy and entropy of ion exchange process were calculated: \(\Delta{G_{T_{2}-T_{1}}^{0}}\) = 13,88±6,94 kJ/mol, \(\Delta{S_{298}^{0}}\) = 85,08±4,25, \(\Delta{S_{343}^{0}}\) = 91,23±4,56 J/mol∙K. Obtained thermodynamic data characterize the feasibility of using D-403 anion exchange resin to recover REEs and separate them into individual components.

Reference