Deformations assessment during subway escalator tunnels construction by the method of artificial freezing of soil for the stage of ice wall formation
Abstract
The work is devoted to the study of the processes of displacement and deformation of the surface during the escalator tunnels construction of the subway by the method of artificial freezing of soils. The features of the construction and freezing technology, the rocks characteristics in which the escalator tunnels made are considered. The data of specially organized, full-scale surveying observations of deformations on the earth surface are presented. The main factors influencing deformation processes in the frozen strata of a layered inhomogeneous rock mass with inclined tunneling are determined, the complexity of the predictive task and the need to simplify the design scheme are shown. The work is focused on the assessment of the least studied geomechanical processes of soil heaving-uplifts and deformations during the periods of active and passive freezing stages. When studying the displacements processes of the earth surface and rock mass, the finite element method and analysis of the obtained data using field observations of displacements were used. A simplified calculation scheme is proposed for modeling, which allows taking into account the uneven influence of frozen rocks of an inhomogeneous layered rock mass with a large inclined tunneling. The satisfactory convergence of the data of field surveying observations on the earth surface and the results of modeling geomechanical processes for the period of active and passive freezing stages is shown. The proposed calculation scheme is recommended for the prediction of deformation at the stages of underground construction, characterized by the development of the most dangerous tensile deformations of buildings and structures on the surface.
Introduction
The development of modern megacities is impossible without improving their transport infrastructure. One of the reliable and proven solutions in the field of public transport system development is the design and construction of subways. A large cross-section of subway workings, often flat-laying and complex layout create unfavorable conditions for the deformations occurrence on the earth surface and determine the problem of protecting buildings and structures from the harmful effects of mining, especially for areas with dense historical buildings.
Of the well-known complex of deep-laid underground workings, the construction of escalator tunnels connecting the station to the earth surface has the most significant impact on the earth surface and the buildings being worked on. For example, in the construction of the main station tunnel complex (according to available field observations), subsidence on the surface, as a rule, does not exceed 100 mm, while during the construction of escalator tunnels, these values can reach 400-450 mm [15]. In difficult engineering and geological conditions (in the presence of weak, unstable rocks and several aquifers in the thickness), the driving of such inclined workings is carried out only by special methods, for example, by freezing. For the mining and geological conditions of St. Petersburg and Moscow, the construction of escalator tunnels by freezing is economically more profitable and technically well-developed in comparison with the new method based on tunneling mechanized complexes (TMC) [13, 16].
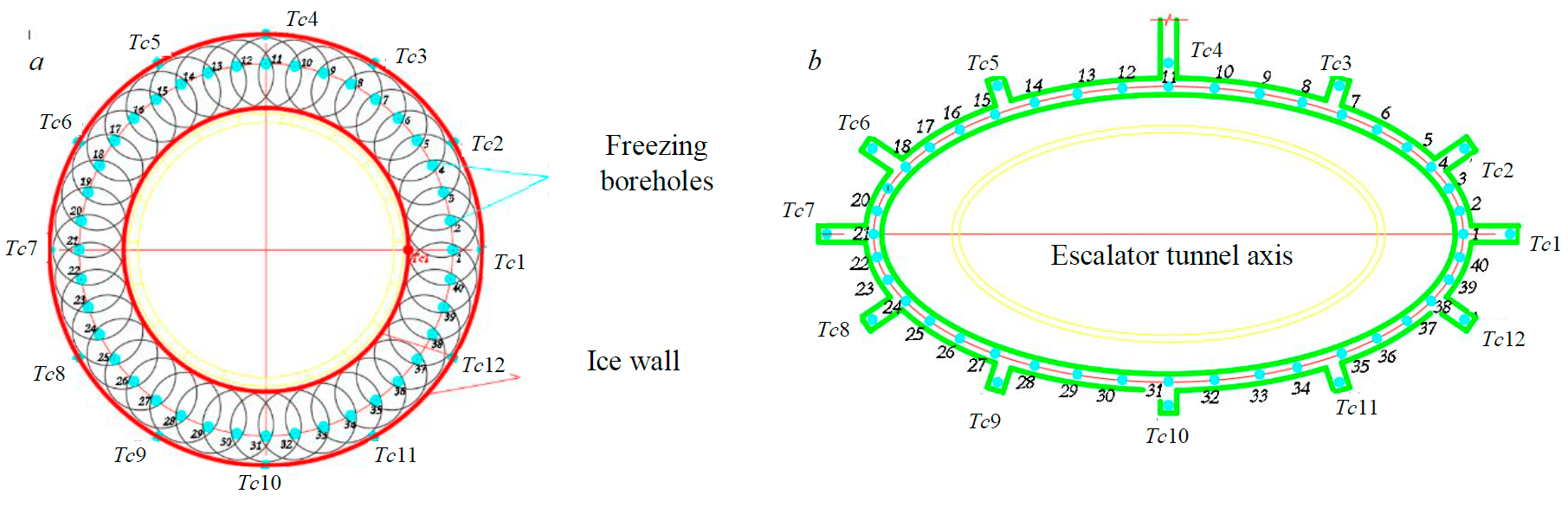
Fig.1. Location of freezing thermometric boreholes perpendicular to the axis of the tunnel (a) and on the earth surface (b) [27]
Underground construction is always accompanied by a violation of the stress-strain state of the rock mass, and during the construction of escalator tunnels by freezing, these violations are associated not only with the driving of the workings, but also with the processes of the ice wall growth, and then the thawing of rocks.
With the classical method of soils freezing, a temporary ice- wall is artificially created around the future structure [7, 17]. This wall is formed from frozen rock cylinders formed around boreholes drilled along the outer contour of the underground structure (Fig.1). Technologically, there are three stages: active freezing (formation of an ice wall in the rock mass), passive freezing (maintenance of the soil in a frozen state for the construction period) and thawing of the ice wall (after finishing the driving).
At the first stage, deformations and uneven soil heaving of rocks in the rock mass begin to occur and develop. They also occur on the earth surface, leading to the development of the most dangerous tension deformations over the tunnel axis. The second stage is also characterized by the appearance of the soil heaving development (they do not stabilize and continue to increase). The rate of soil heaving-uplifts development on the earth surface is two to three times less than at the active stage of freezing, however, the total maximum values of soil heaving on the surface can reach 200-250 mm [4]. The process of natural rocks thawing at the third stage lasts up to 4-5 years after finishing construction. The stage is characterized by the slow development of subsidence and complex, heterogeneous deformations in the rock mass due to the imposition of the processes of recovering the volume of rocks during thawing, secondary redistribution of stresses and deformations, reduction of strength and deformation properties of rocks, migration of groundwater and leaching of rock particles with it.
Despite a large number of studies on the mechanics of heaving soils and permafrost, artificial soils freezing, geomechanical control and assessment of displacements, the problem of reliable quantitative assessment of deformations using this technology is still relevant, since in studies in the field of heaving soils mechanics [26, 28] cyclic processes of thawing and freezing of near-surface layers of soils are mathematically described, but deformation processes with artificial (controlled) freezing are practically not considered. In the field of artificial soils freezing [1, 5, 6], the main attention is paid to the assessment of the freezing front movement through the description of thermophysical and geomechanical processes [11], the assessment of the thickness of ice walls, their strength and changes in the modulus of deformation, but not the assessment of deformations during freezing-thawing of rocks. Numerous studies on geomechanical estimation of displacements in underground construction [3, 22, 25], focused specifically on the quantitative description of deformation processes on the surface, often operate with horizontal workings [29, 30] and do not consider freezing as a force or mechanical factor in the calculated estimates [3, 22].
The most profound and comprehensive study on assessing movements during the construction of escalator tunnels was done by S.N.Silvestrov*. Despite a number of obvious advantages of S.N.Silvestrov's complex approach, who used theoretical methods, field data and physical modeling, it should be noted that his method does not take into account the influence of deformation processes during the rise of the ice wall and the discrete heterogeneity of the rock mass, also, the approaches to determining the position of the point of maximum subsidence in the shift trough, assessing the shape and position of the boundaries of the deformation zone on the surface are too simplified.
In modern works based on numerical modeling by the finite element method [2, 20, 21], there were also attempts to estimate the displacement of rocks and the earth surface for escalator tunnels. However, comparisons of such modeling data with the data obtained by the traditional methodology and field data revealed significant discrepancies [16], which were explained by the significant soil freezing that often takes place in practice, as well as the overlap of two different processes – caused by freezing-thawing of rocks and mining operations during tunneling. The main disadvantage of such model studies should be recognized as the insufficient use of the surveying observations results, which are able to correctly verify such finite element models.
The presented review allows noting the following main disadvantages of the previously used approaches to the assessment of deformations during the construction of escalator tunnels by freezing:
- the absence in most works of a comprehensive approach combining a theoretical assessment of the movement of the freezing front, empirical methods and modeling, to identify patterns of displacement and assess the real parameters of the ice wall;
- unacceptable averaging of the parameters of the ice wall by lithological differences and by the cross section of the workings;
- ignoring in the calculated estimates of deformations for the stage of the ice wall formation, at which large tensile deformations develop on the surface;
- the absence of dependencies for determining the parameters of the displacement process for specific mining and geological conditions that are used in engineering methods.
The difficult task of quantifying the parameters of the displacements and deformations process in the rock mass during the construction of escalator tunnels by freezing is associated with the simultaneous consideration of processes of different physical nature, complex three-dimensional and asymmetric nature of force, physical, thermophysical and hydrogeological processes, the parameters of which vary over time. It is necessary to divide the task into a number of simpler sequential ones, reasonably exclude secondary factors and parameters from the calculation, involve theoretical methods and generalize field data, simplify calculation schemes.
Due to the lack of knowledge of the processes of rock mass deformation at the first two technological stages of construction of escalator tunnels (stages of development of freezing zones), as well as the occurrence of the most dangerous tensile deformations for buildings and structures, the task of constructing a predictive assessment is most relevant. The simpler physical nature of the processes of the first stages allows to consider the possibilities of developing modern methods for reliable and accessible forecasting of the occurrences of these processes. Next, the processes associated with the freezing stages are considered, for which it is necessary to justify the design scheme and methodology for assessing displacements and deformations, taking into account the main factors and typical data of engineering surveys.
Formulation of the deformation assessment problem
Setting up a forecast calculation
The complex geomechanical problem of forecasting deformations in modern conditions can be successfully solved on the basis of modeling by numerical methods. These methods have been tested and are quite common in research practice [12, 18, 24]. They allow to take into account in the calculations the geological heterogeneity of the rock thickness, changes in the properties of rocks during freezing, physical nonlinearity, temperature deformations of rocks, deformations during excavation, including by stages (breaking and outcropping of rocks, temporary support, permanent support, etc.), moreover, at the level of a correct description of physical and mechanical processes. This approach also has disadvantages – the calculations are approximate and directly depend on the input properties, parameters and models of the environment. These disadvantages can be minimized by using multivariate modeling and model adjustments based on field data (i.e. model verification). As field data, it is proposed to use data on deformations of the earth surface and contours of workings as output parameters of mathematical models.
The use of complex medium models that take into account, in addition to mechanical properties and properties of heat and mass transfer, is not always justified. Therefore, in most cases, it is possible to do with single-phase physical models of rocks and the correct assignment of theoretically calculated soil heaving deformations in the mode of modeling specified deformations.
The key role in the development of deformations in an inhomogeneous rock mass and on the surface for the considered freezing technology is played precisely by volumetric expansion-compression deformations (soil heaving-compaction) during the phase transition and the degree of their development along the thickness.
Assessment of the ice wall formation
The beginning of the freezing aquifers process is associated with the organization of artificial heat exchange in the rock mass, in which zones of negative temperature are formed around freezing boreholes, where frozen cylinders originate and develop, which have an inhomogeneous thickness in each layer, and the freezing front moves unevenly (with slowing down) and inhomogeneously across layers. The parameters of the frozen cylinder and its changes over time are determined by a whole group of independent parameters: temperature (t), type of rocks, their natural humidity (W), density (ρ), thermal conductivity, etc. The formation of an ice wall during the active stage of freezing is associated with the rock swelling, the formation of ice layers, the migration of water to the freezing front, etc., which ensures the development of deformations of volumetric expansion (soil heaving) in the rock mass and on the earth surface [10, 23, 34].
From a technological point of view, the main quantitative indicator of freezing is the thickness of the ice wall. Freezing boreholes intersect many geological layers with various physical-mechanical and thermophysical characteristics that affect the movement speed of the freezing front. The use of means of controlling the parameters of the wall comes to the fore in conditions of difficultly predictable thermophysical and hydrogeological processes (observational thermometric boreholes, geophysical, etc. methods), when the thickness of the wall is provided by varying of the freezing mode experimentally. Observations show that clay water-saturated soils are the hardest to freeze [20, 21], therefore, during the active stage of freezing, the period of reaching the design thickness of the ice wall of one of these “heavy” layers is forcibly taken, at this time, soil over freezing is observed in other layers and the actual thickness of the ice wall exceeds the design values.
For a preliminary assessment of the freezing parameters, researchers propose various models of the development of the freezing front and dependencies for the thickness of the ice wall, including temporary ones [21]. In practice, these preliminary technological parameters are adjusted based on the results of control observations of freezing, and the model indicators can be refined for specific mining and geological conditions.
Therefore, to solve the problem of deformation forecasting, two main determining parameters should be numerically estimated: the thickness of the ice wall (or the volume of frozen rock) and the relative volumetric deformation of these frozen rocks separately by lithological differences.
Methodology and research methods
Theoretical method for assessing the development of the freezing front
Assessment of the thickness of the ice wall can be obtained on the basis of known dependencies. For example, for the conditions of construction of escalator tunnels, D.A.Potemkin proposed a dependence that determines the thickness of the ice wall for each lithological difference [21]:
where λ – coefficient of thermal conductivity, kJ/m∙h∙°С; W0 – humidity, %; с – specific heat capacity, kJ/kg∙°С; γ – volume weight, t/m3; Тcw – freezing column wall temperature, °С; Тs – sublimation temperature, °С; Тf – freezing temperature, °С; Еw – latent heat of ice formation of water, kJ/kg.
The dependence is derived from the general equation of thermal conductivity, takes into account the temperature fall zone during cooling before the ice wall formation, the used parameters are estimated for rocks of the quaternary thickness. Dependence (1) allows to calculate the thickness of the ice wall at time t (i.e. it can potentially allow solving problems taking into account the time factor), takes into account the real thermophysical properties of quaternary rocks.
Justification the simplified method of forecasting the development of deformations for the stages of formation and maintenance of the ice wall
The determining force factor in the design scheme for the first stages of the construction of escalator tunnels are the loads associated with volumetric expansion – soil heaving of frozen rocks. Therefore, the task of its correct accounting for different geological layers is primary. The main initial parameters in this calculation are the geometric dimensions of the frozen rock zone and the parameter determining the volumetric expansion. If the first parameter is evaluated fairly strictly through known dependencies of type (1), then it is difficult to evaluate the second with strict methods. It is for the second parameter that it is proposed to justify a simplified approach.
The primary model analysis undertaken in the study using full-scale displacement data for tunnels in St. Petersburg has showed that the specified heaving deformation differentiated by the layers of the model makes it possible to ensure that the model calculation corresponds to full-scale data, and the deformation indicators correlate with the specific indicators of the fluid content in the pores of specific rocks (which was estimated by the natural humidity of rocks). As one of the options for estimating volumetric deformations, a simplified approach was proposed, based on taking into account the known effect of volumetric expansion of water (disconnected water in rocks) during its crystallization, taking into account a differentiated assessment of the geometric parameters of a real ice wall.
The implementation of the geomechanical problem of volumetric expansion can be based on the use of numerical modeling (for example, the finite element method) in the mode of specified volumetric deformations, which allows to correctly consider the geometry of an inhomogeneous ice wall for an escalator tunnel. With this simplified method, the calculation is performed at a given moment of freezing time through ξ = ξ(t). The calculation allows to take into account the measurement of the thickness of the ice barrier along the rock layers and the parameters of the volumetric expansion εv (estimated, for example, by the natural humidity of the rocks), and most importantly, the additional mechanical load on the rock mass from freezing and the dynamics of its complex heterogeneous deformation. The calculation does not allow to take into account the complex processes of humidity migration associated with freezing or changing the filtration properties of rocks, the heterogeneity of temperature fields in the under-worked thickness and the dynamics of its changes (especially after the start of tunneling) and other factors and processes accompanying this technology, however, the nature of their influence on the deformation processes under consideration, according to our estimates, is more related to the indicator of natural humidity of rocks. The latter statement refers to specific mining and geological conditions and in other conditions requires justification or empirical confirmation.
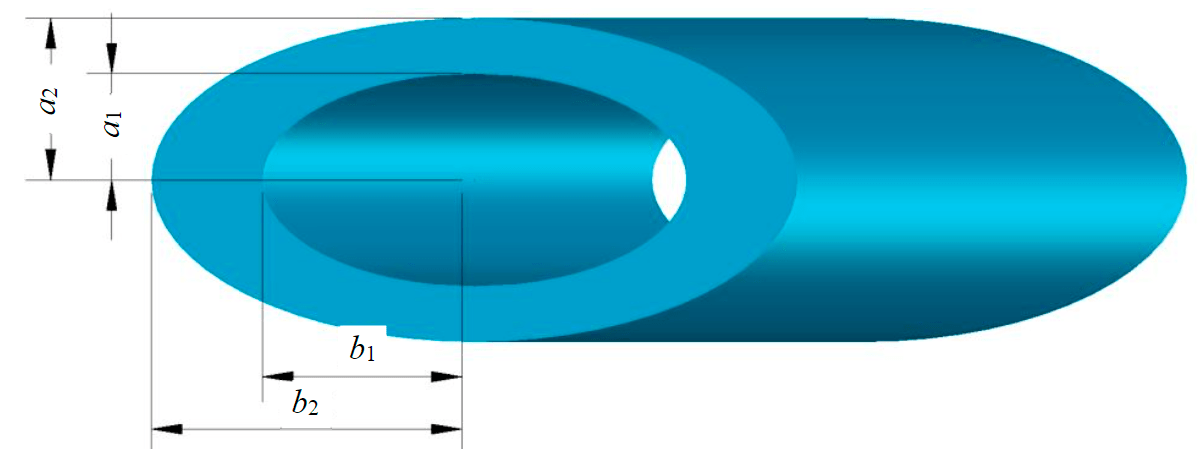
Fig.2. The sector of the frozen cylinder with thickness h within one layer (top view)
To account for specific humidity indicators, the standard rock tests during surveys can be used. The main parameter is the relative natural humidity by weight, so in calculations it should be reduced to volumetric indicators.
To account for the size of zones of frozen rocks of complex geometric shape, analytical geometry formulas can be used if the non-horizontal nature of geological contacts is neglected. In a layered, discretely inhomogeneous rock mass with different physical-mechanical and thermophysical properties, the volume of the growing ice wall at a given time will be calculated for each layer separately by sectors of a flat-lying truncated cylinder (Fig.2).
The volume of frozen rock for the sector can be calculated using the formula
where h – the thickness of the geological layer, m; a2 – the outer radius of the ice wall, m; a1 – the inner radius of the ice wall, m; a2 – a1 – the design thickness of the ice wall, m; b2 – b1 – projection of the thickness of the ice wall taking into account the slope, m.
Since the axis of the escalator tunnel runs at an angle of 30°, the dimensions of the semi-axes of the ellipses b can be expressed as
Substituting expressions (3) into formula (2), the following formula is obtained:
Therefore, the volumes of the sectors of the frozen cylinder with the design thickness for each geological layer with the thickness h are calculated.
The specificity of model calculations (when it is technically difficult to differentiate and set in a model the size of freezing zones according to lithological differences) does not simply allow the individual thicknesses of walls by rock to be included in the calculation, usually one have to deal with a uniform (the entire length of the tunnel) thickness of the ice wall. To simplify such model calculations, it is more logical to take the design thickness as a conditional uniform thickness. Therefore, in order to correctly assess the deformations in the calculations, it is necessary to additionally take into account the discrepancy between the design (averaged) volume of the ice wall and the actual one (for example, estimated by the method of D.A.Potemkin). Such accounting is possible through the calculation of an indicator that takes into account the difference in these volumes for each rock layer selected in the model.
An important parameter for geomechanical calculations of ice wall is the asymmetry of the development of frozen cylinders around boreholes due to the heterogeneity of thermophysical parameters along the inner and outer contour of the wall. Observations of the development of ice walls during the construction of escalator tunnels show that the inner part accounts for 60 % of the total thickness and the outer part – for 40 % (see Fig.1, a).
To account for this asymmetry and transition to the actual thickness of the wall, the parameter r (radius of the axial surface of the freezing boreholes) is introduced:
The resulting expressions (5) are substituted into the formula (4), the volume of the sector for each geological layer with a thickness of h is obtained:
where ξtw – the thickness of the ice wall, calculated according to the formula (1) for each geological layer with a thickness of h.
Now the expansion parameter of the frozen rocks is estimated based on the known parameter for crystallizing water, which increases in volume by 9 % [21]. Traditionally, in the practice of surveys, the main parameter characterizing the water content in rocks is the specific humidity content of rocks W, i.e. the ratio of the water mass to the mass of dry rock (rock skeleton),
The total mass of the frozen rock should be considered as the sum of the masses of water and the rock skeleton, it can also be estimated through the rock density
An expression is obtained for the water mass in the considered volume of the frozen rock mass (sector):
Next, the volume of water in the sector is considered:
where – water density, = 1000 кг/м3.
The relative change in the volume under consideration or the specified volume deformation indicator (for model calculations) will be determined by the following expression:
The assessment of this indicator for the types of rocks in demand showed the following levels: for Quaternary deposits 4-5 %, for Proterozoic rocks 2-3 %.
Modeling of geomechanical processes by the finite element method (FEM)
Field data are usually limited and allow to assess and predict the development of deformations only on the earth surface and in the constructed tunnel. The implementation of monitoring observations through boreholes is associated with serious technical problems, the intensive development of large alternating deformations will lead to a significant violation of the well shafts of observation boreholes. Therefore, in the absence of opportunities to organize modeling on equivalent materials, the most accessible means of assessing the impact of active and passive freezing on the rock mass are mathematical modeling methods [8, 14, 25].
The FEM allows calculating the stress-strain state of an inhomogeneous, anisotropic, physically nonlinear rock mass with varying physical and mechanical properties. It allows taking into account the parameters of the support and the possibility of step-by-step modeling of the driving. With the integrated use of data from field surveying observations and modeling of geomechanical processes to assess the harmful effects of underground construction on the earth surface, it becomes possible to ensure the reliability of the results obtained at the level of quantitative indicators of deformations [18, 31].
In this paper, the construction and calculation of models were implemented in the PLAXIS 3D software package. This is one of the most popular and adapted software systems for geotechnical calculations, implementing FEM. The problems were solved in a spatial formulation (for problems with escalator tunnels a flat formulation is not able to provide an adequate result), with the symmetry of the design scheme, half models were mainly considered, standard boundary conditions were set (horizontal movements were prohibited along the lateral faces and the axis of symmetry, vertical movements – along the lower boundary), the main type of finite elements was a multi – node (isoparametric) tetrahedron.
The main models used in modeling tunneling in this study are: linear-elastic (for modeling the support); ideal elastic-plastic Coulomb – Mohr; elastic-plastic with soil hardening (Hardening Soil).
As a result of a series of calculations using these types of models for modeling escalator tunnels, due to the better convergence of modeling results and field data of surveying observations, it was decided to use an elastic-plastic model with soil hardening with a drained type of soil.
The elastic-plastic model with soil hardening (HS) better describes the behavior of the rock mass under conditions of sequential loading and unloading. Unlike the Coulomb – Mohr model, here the yield surface is not fixed in the main stress space and can expand due to plastic deformation. In this model, soil hardening occurs both during shear and compression. The elastic-plastic model with soil hardening is intended for soil modeling. The stiffness, which depends on the stresses according to the power law, is given by three different input parameters. These are the deformation modulus at three-axis loading Е50, at unloading Еurand the odometric module Еoed [9, 32].
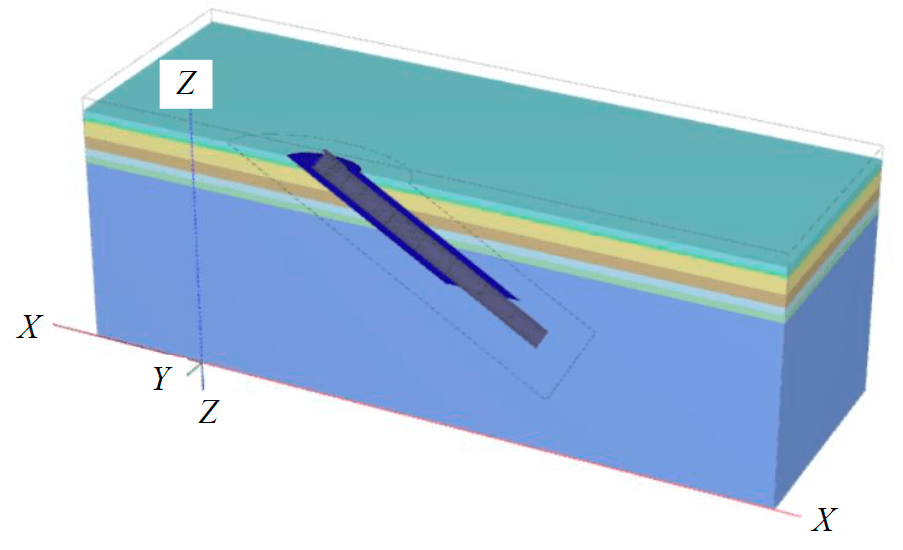
Fig.3. Example of half of the design scheme of the rock mass with the thickness of an ice wall and an escalator tunnel
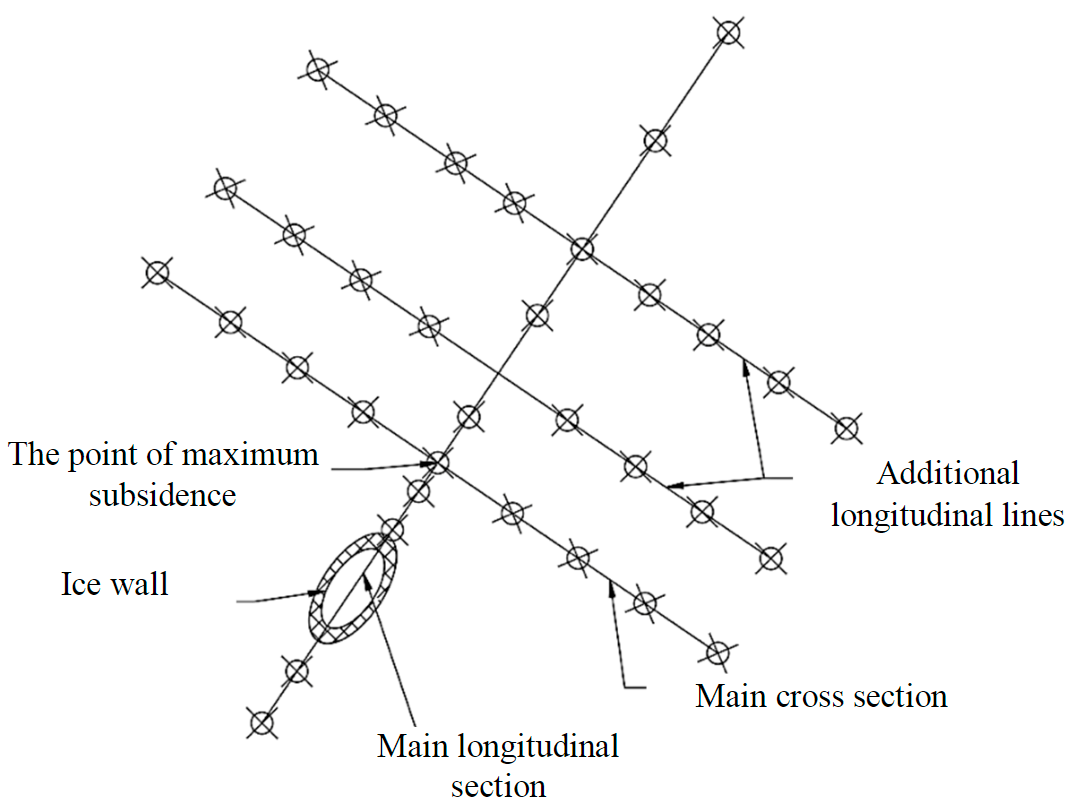
Fig.4. Observation station during the construction of an escalator tunnel passed with soils freezing
The dimensions of the rock mass models were taken taking into account the minimization of the boundary effects influence on the calculation results. As the main options for modeling, models with dimensions of 300 and 150 m, respectively, along the horizontal projection of the axis of the escalator tunnel and across it are accepted. Rock layers are presented in a simplified way – in the form of a set of horizontal layers (since the thickness of geological elements was relatively sustained).
The main objects in modeling the tunneling of escalator tunnels were elements modeling rocks, the area of the ice wall (in the form of a truncated hollow cylinder with a given thickness) and the lining of the escalator tunnel, modeled by two-dimensional elastic elements of the plate type (Fig.3).
Due to the complexity of the actual volume increase in the models, the thickness of the ice wall was assumed to be constant (corresponding to the design). To simulate real deformations for each geological element, its own (real) thickness of the ice wall was calculated using the formula (1). In accordance with the proposed simplified methodology for the implementation of a given deformation mode for each layer, the volumetric deformation indicator was calculated according to the formula (11).
The main test object was the escalator tunnel of the Kazakovskaya metro station in St. Petersburg, where an impressive series of full-scale data on soil heaving on the surface above the tunnel was obtained.
The physical and mechanical properties of the rocks of the Quaternary deposits of the sites under consideration vary widely. According to engineering and geological tests, the deformation modulus E varies from 7 to 20 MPa, the coupling c from 13 to 80 kPa, the angle of internal friction j from 14 to 20°. The rock masses in which escalator tunnels are constructed by freezing soils are characterized by significant heterogeneity of granulometric composition and consistency, the humidity of rocks varies within 14-25 %. For each escalator tunnel, it is necessary to build its own unique mathematical model, taking into account all the physical-mechanical and thermophysical properties of rocks.
For the second stage of construction, tunneling is simulated (rock development and installation of support in a frozen rock mass).
Methods of field research
The main source of reliable data in the study of the processes on the earth surface displacement are the results of field surveying observations. Before the start of work on the future tunnel, special observation stations are laid. An observation station on the earth surface should be a network of mutually perpendicular lines of reference points (well-fixed points on the surface) located along and across the axis of the escalator tunnel. Due to the previously identified features of the shift trough development over escalator tunnels [19], in order to ensure reliable results for displacements and deformations on the earth surface in the transverse direction, it is necessary to focus not only on the point of maximum subsidence, but also on additional profile lines perpendicular to the axis of the tunnel, for example, the point of maximum width of the shift trough (Fig.4).
Based on these principles, an observation station was designed and laid for the escalator tunnel of the Kazakovskaya metro station of the Lakhtinsko-Pravoberezhnaya Line of the St. Petersburg subway. The active freezing stage lasted 54 days here (on average, the active freezing period lasts 50-60 days, depending on the depth of the freezing boreholes). The altitude position of the initial reference points is determined by leveling them, with the accuracy of leveling class III [33].
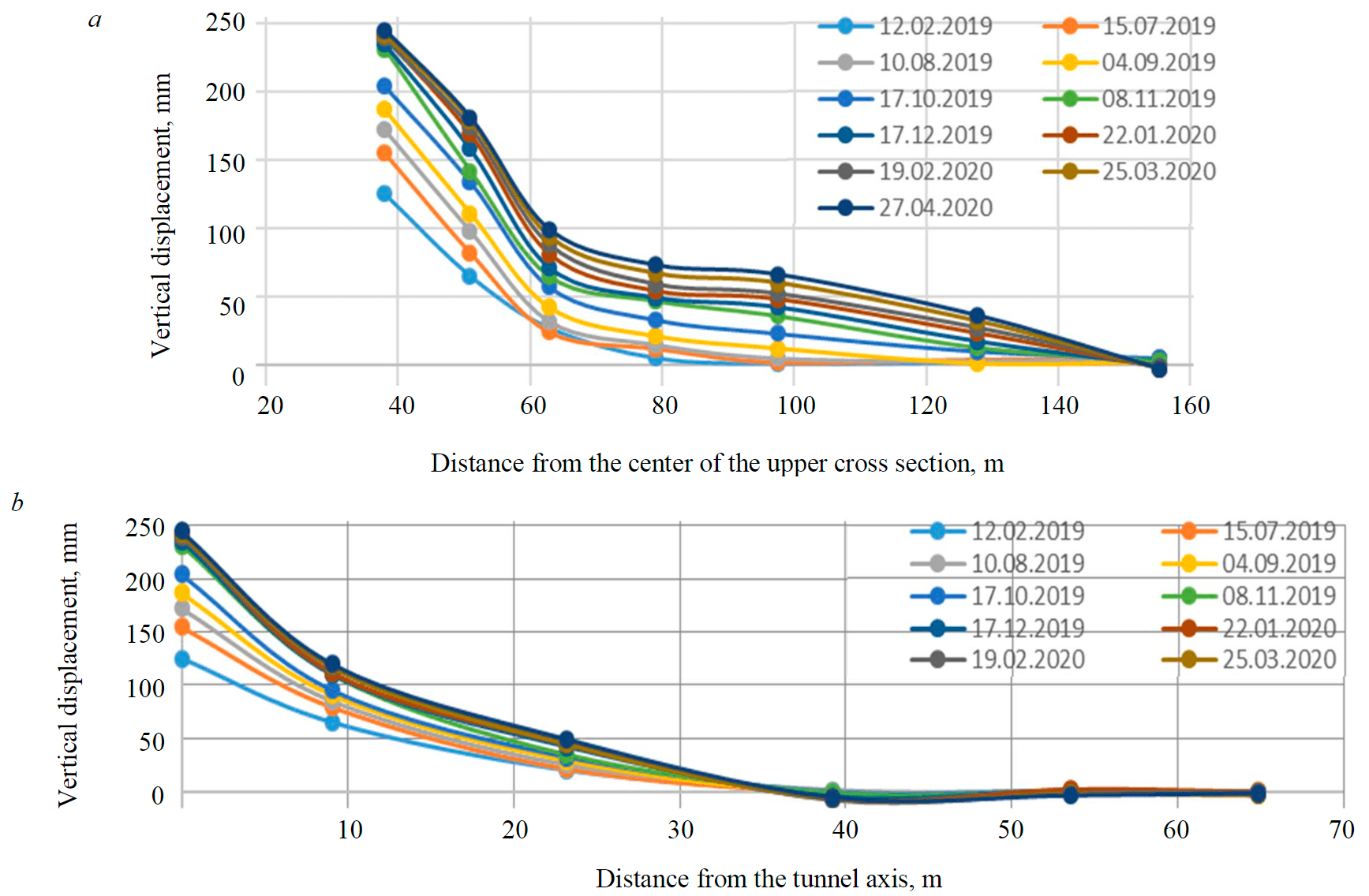
Fig.5. The half-shift trough of the main longitudinal section (a) and the main cross-section (b) in the St. Petersburg metro station “Kazakovskaya”
The main results of the deformation assessment
Displacements in the rock mass and on the earth surface according to field observations
Field observations during active and passive freezing showed that the uplifts (soil heavings) increase systematically without transition to subsidence, the rates of soil heaving increase for the reference points are not the same and heterogeneous in time, the rates and levels of soil heaving are higher for the reference points located above the axis of the tunnel. At 35-40 m from the axis of the tunnel, soil heaving on the surface was practically not manifested. The development of multiple displacements (soil heavings) in the main longitudinal and main cross-section for the escalator tunnel of the Kazakovskaya metro station are shown in Fig.5.
At the end of the active freezing stage and the beginning of driving, the maximum soil heaving on the earth surface was 98 mm, at the time of shutdown of the refrigeration unit – about 245 mm. Therefore, most of the final displacements (about 60 %) were initiated at the stage of passive freezing and mine driving, which was not initially expected and was not predicted.
A significant unevenness of displacements (uplifts) along the reference points determined the occurrence of large horizontal tensile deformations on the surface, which were estimated at a level of up to 0.03-0.04. This level exceeds by an order of magnitude the known limit of deformation for the earth surface. In real conditions of the presence of asphalt pavement, cracks with an opening of up to 3-7 cm were recorded in it.
Displacements and deformations based on the simulation results
Modeling at the freezing stages showed an intensive development of deformations and displacements of rocks in the quaternary thickness from the tunnel tray to the earth surface (along the entire overlying thickness), the maximum concentrations of deformations and the largest vertical displacements in the rock mass were recorded in the upper third of the tunnel length in the upper zone of the rock mass (Fig.6, a). The maximum vertical displacement – soil heaving (yellow zone) is confined to water-saturated layers with the lowest deformation characteristics, which is explained not so much by these properties as by deformations specified in the model (since the thickness of the ice wall is the largest here).
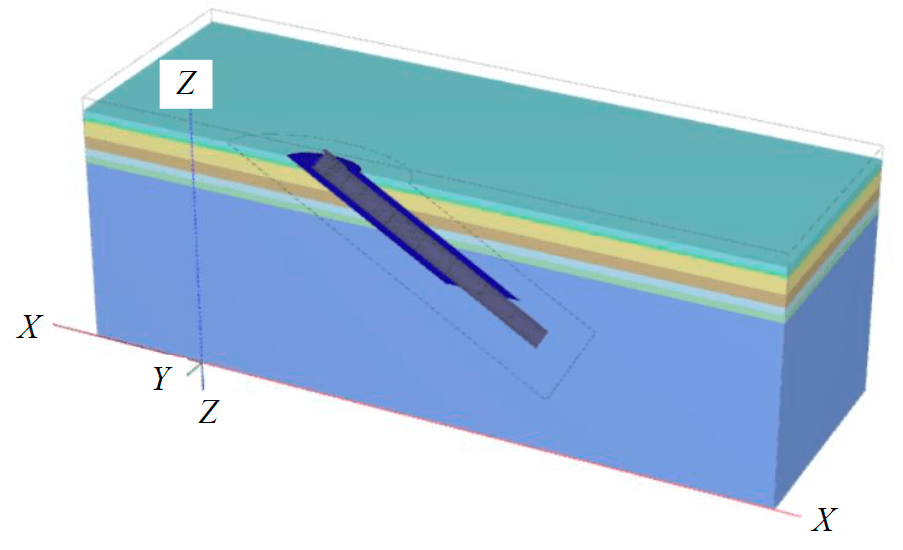
Fig.6. Modeling of vertical displacement distributions at the time of shutdown of the refrigeration unit (a) and driving in the frozen rock mass (b) for the escalator tunnel of the Kazakovskaya metro station
Modeling has shown that the influence of step-by-step rock development and installation of cast-iron lining also has a non-standard effect on changing the SSS of the rock mass. Soil heaving on the earth surface and in the rock mass continued to develop (at a slower rate compared to the period of the active freezing stage), the transition to subsidence did not occur (Fig.6, b). Partial intensification of vertical displacements – surface uplifts was also determined by the effect of “surfacing” of the structure being constructed (the weight of the rock is 5-6 times higher than the weight of cast-iron tubing rings – the main weight load) [14].
According to the simulation results, the maximum soil heaving on the earth surface during the transition from the active stage of freezing to the passive stage was 100-105 mm, at the end of the driving with freezing – 260-265 mm.
The step-by-step tunnel driving showed a gradual uneven increase in vertical displacements of the lining to a value of about 100 mm in the upper zone. The unevenness of such displacements led to the lining deformations: the curvature of the tunnel axis in the vertical plane increased, longitudinal (along the tunnel axis) stretchings in the lining and other secondary effects developed.
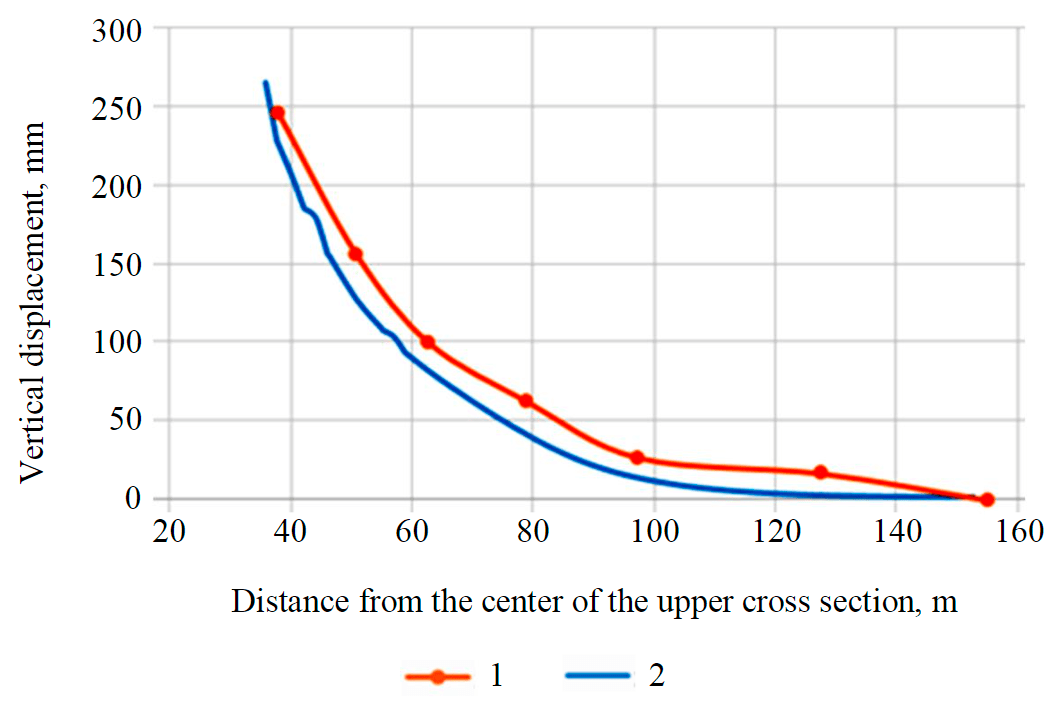
Fig.7. Comparison of the half-shift trough of the full-scale data (1) and modeling (2) at the time of shifting the refrigeration unit
Discussion and results analysis
It can be seen from the field data that the level of uplifts (heavings) on the surface can reach very significant values, and their unevenness leads to very large deformations of curvature and horizontal stretching deformations up to 0.03-0.04. These levels are 10-20 times higher than the limit values (2∙10–3). Such deformations are very dangerous and destructive for ground objects, their neglect in the design, even with the temporary nature of the occurrence, is unacceptable.
The proposed step-by-step model calculation of deformations and the scheme for estimating the level of specified expansion deformations from freezing are able to satisfactorily describe even the increasing dynamics of displacements in a layered rock mass with various physical-mechanical and thermophysical properties of rocks. The models showed similar dynamics of the increase of uplifts by stages (Fig.7). In general, according to the simulation results, the maximum uplifts (at the point of expected maximum subsidence) at the time of shutdown of the refrigeration unit were 264 mm, and according to full-scale data 245 mm (Fig.7), for the main uplift zone such discrepancies did not exceed 10-20 %. The explanation of the paradoxical effect of the prevalence of displacements in the passive stage revealed in the study (which should not increase the uplift at all) may be the well-known effect of refreezing the rock mass and the duration of this stage, which is many times longer than the duration of the active freezing stage.
In addition to identifying the main mechanism of the development of uplifts (soil heavings) of the surface, modeling allowed to assess the picture of the development of deformations in the entire rock thickness. The uneven distribution of displacements in the rock mass is determined by the concentrations of deformations both in the freezing zones (the zone of volumetric expansion) and in the zones directly adjacent to them, especially in the zone of the “upper triangle” above the tunnel (the zone of the under-worked rock mass). The distribution of displacements is characterized by the spread of an extended zone of maximum displacements over the roof of the workings (the zone is localized about 2-3 m above the tunnel in the upper quarter along its length), here the displacements reach 400-460 mm. With a distance from this zone, the displacements fade, to the surface of the displacement significantly (1.5-2 times) decrease and do not exceed 200-250 mm. Freezing zones and adjacent zones with a depth closer to the end of freezing boreholes (i.e. reaching Proterozoic clays) no longer exceed 100-150 mm and quickly fade along the overlying thickness to the surface. Such displacement distributions (with maxima inside the rock mass above the tunnel) are explained by the development of high values of residual (plastic) compression deformations in local zones of the weakest rocks of the Quaternary layer caused by the action of strong soil heaving pressure of frozen rocks.
The reliability of the approach to the deformations assessment justified in the work is confirmed by the good convergence of the simulation results with field surveying observations and to the parameter of shift trough volumes, where the calculated volumes from the field data and the simulation results have a discrepancy of 6-10 %.
Conclusion
The construction of subways is a necessary measure for the megacity. The developed infrastructure and historical development of cities obliges to assess the harmful impact of such underground construction on the earth surface. With the deep laying of subway lines, the most technologically difficult and dangerous task in terms of deformations is the construction of an escalator tunnel using freezing technology.
The technology of artificial freezing of unstable and flooded soils, traditionally used for escalator tunnels, on the one hand, is characterized by lower cost and refinement of the technology, on the other hand, reveals itself in an aggressive effect on the rock mass, which at the first stages of work under freezing experiences large and dangerous soil heaving deformations, many times exceeding the standard level of dangerous deformations, and at the last – significant subsidence. Subsidence on the surface reaches 450 mm.
The task of assessing the deformation state of a rock mass and a surface using such technology is one of the most complex geomechanical tasks, simultaneously developing processes of different physical nature over time are determined by several dozen parameters. A large number of works have been devoted to the problem of assessing the impact of these processes on the surface, but the least studied are the first stages of construction of escalator tunnels with an increasing ice wall, for which there are still no reliable assessment methods.
The assessment of the main parameters of the displacement process was made on the basis of specially organized and standard data, methods of field measurements, which showed the development of very high uplifts (soil heaving) of the surface up to 200-250 mm and extreme deformations at the first stages of the work. More than 60 % of the value of these uplifts was obtained at the passive freezing stage during the mine driving, which is very unusual.
To solve the problem of forecasting the assessment of displacements and deformations, taking into account the main identified factors, a simplified approach is proposed based on the use of numerical modeling by the finite element method with a layer-by-layer assessment of the parameters of the ice wall by two basic parameters: the thickness of the wall (estimated by theoretical dependence) and volumetric deformation (estimated by the level of natural humidity of rocks) with its input into the model in the mode of specified deformations. To assess these parameters, it is possible to use the data of direct field methods for evaluating the freezing operation (by thermometry and strain sensors in the rock mass).
The results of finite element deformation modeling for escalator tunnels in the stages of active and passive freezing phases constructed in the mining and geological conditions of St. Petersburg showed good convergence with the data of field observations. Deviations in displacement amounted to about 10-20 %, which gave reason to recommend such a simplified approach in the forecast assessment of tensile deformations dangerous to the earth surface and tunnel lining, both at the design and construction stages, taking into account the actual data on freezing parameters. Modeling allowed to identify the causes of the paradoxical development of soil heaving deformations by freezing stages and analyze the mechanisms of deformation development in the rock mass.
References
- Zabenkova N.A., Galkin N.S., Stoyakova K.L., Besfamilnaya E.M. Analysis of variable soil freezing methods. Tekhnika. Tekhnologii. Inzheneriya. 2018. Vol. 1 (7), p. 32-35 (in Russian).
- Belyakov N.A. Development of a method for predicting the stress-strain state of transport tunnel linings in a disturbed rock mass: Avtoref. dis. … kand. tekhn. nauk. St. Petersburg: Sankt-Peterburgskii gosudarstvennyi gornyi universitet, 2012, p. 24 (in Russian).
- Volokhov E.M., Zelentsov S.N., Khutskii V.P. Standard and methodical support of estimation of adverse effect of underground construction and monitoring of deformations for conditions of Saint Petersburg. Journal of Mining Institute. 2012. Vol. 199, p. 260-262 (in Russian).
- Volohov E.M., Mukminova D.Z. The Problem of Evaluation Harmful Effects Mining Works During Construction of Subway Escalator Tunnels with the Help of a Freezing of Soils. Mine Surveying Bulletin. 2019. Vol. 2 (129), p. 47-55 (in Russian).
- Igolka D.A., Igolka E.Yu., Luksha E.M., Kologrivko A.A. Influence of an ice wall temperature at calculation of the shafts lining system. Gornaja mehanika i mashinostroenie. 2014. N 2, p. 36-41 (in Russian).
- Brovka A.G., Brovka G.P., Dedulya I.V., Agytin K.A. The Dependence of the Thermophysical and Strong Characteristics of Frozen Soils from Temperature. Nature Management. 2017. Vol. 31, p. 45-49 (in Russian).
- Freezing of rocks during the construction of mines. URL (date of access 10.07.2020) (in Russian).
- Karasev M.A., Potemkin D.A. Theoretical basis of geomaterial accounted for induced and inherent anisotropy. Journal of Mining Institute. 2013. Vol. 204, p. 263-268 (in Russian).
- Kireeva V.I. Assessment of displacements and deformations of rocks during the construction of large-diameter main line tunnels by tunnel-boring mechanized complexes in difficult mining and geological conditions: Avtoref. dis. … kand. tekhn. nauk. St. Petersburg: Natsionalnyi mineralno-syrevoi universitet “Gornyi”, 2016, p. 20 (in Russian).
- Kotov P.I. Compression deformation of beach-submarine frozen soils during thawing (European North of Russia, Western Siberia): Avtoref. dis. … kand. geol.-mineral. nauk. Мoscow: Moskovskii gosudarstvennyi universitet im. M.V.Lomonosova, 2014, p. 23 (in Russian).
- Mishedchenko O.A. The history of the development of the artificial rocks freezing method. Gornyi informatsionno-analiticheskii byulleten. 2010. N 2, p. 226-231 (in Russian).
- Protosenya A.G., Potemkin D.A., Ogorodnikov Yu.N. et al. Mechanics of underground structures. Spatial models and monitoring. St. Petersburg: Sankt-Peterburgskii gornyi universitet, 2011, p. 355 (in Russian).
- Methods of escalator tunnels construction. URL (date of access 15.12.2019) (in Russian).
- Mosolov D.A. Effective design parameters of lightweight cast-iron lining of subway tunnels: Avtoref. dis. … kand. tekhn. nauk. Мoscow: Nauchno-issledovatelskii institut transportnogo stroitelstva, 2007, p. 28 (in Russian).
- Mukminova D.Z. Assessment of displacements and deformations of rocks using mathematical modeling methods in the construction of escalator tunnels by freezing: Avtoref. dis. … kand. tekhn. nauk. St. Petersburg: Sankt-Peterburgskii gornyi universitet, 2021, p. 20 (in Russian).
- Novozhenin S.Yu., Bogdanova K.A. Experience of Escalator Tunnel Construction Modeling of “Mining Institute” St. Petersburg metro station. Mine Surveying Bulletin. 2020. Vol. 3 (136), p. 40-44 (in Russian).
- Organization of construction and basic means of mechanization of works. URL stroitelstvo_metropolitenov/478/ (date of access 05.11.2020) (in Russian).
- Panfilov D.V. Methodology for predicting deformations of the Earth surface during the construction of transport tunnels based on spatial modeling: Avtoref. dis. … kand. tekhn. nauk. Мoscow: Nauchno-issledovatelskii institut transportnogo stroitelstva, 2005, p. 20 (in Russian).
- Mukminova D.Z. Patent N 2738633 RF. A method for monitoring the development of soil heaving caused by the construction of inclined underground workings passed with soil freezing. Publ.15.12.2020. Bul. N 35 (in Russian).
- Potemkin D.A. Influence of changes in constructive elements of protective constructions on behavior of the soil massif near deep ditches. Journal of Mining Institute. 2013. Vol. 204, p. 240-247 (in Russian).
- Potemkin D.A. Substantiation of the ice wall thickness in a layered rock mass, taking into account the thermophysical properties of rocks and technological parameters of freezing: Avtoref. dis. … kand. tekhn. nauk. St. Petersburg: Sankt-Peterburgskii gosudarstvennyi gornyi institut, 1999, p. 20 (in Russian).
- Gusev V.N., Volokhov E.M., Bak N.S., Novozhenin S.Yu. Calculation of expected displacements and deformations from the construction of underground tunnels in Quaternary deposits of Ho Chi Minh City. Marksheiderskii vestnik. 2012. Vol. 5 (91), p. 51-53 (in Russian).
- Sergeev A.S., Yushkov B.S. On the Freezing of Clay Soil and Water Migration Into the Pavement Design. Russian Journal of Transport Engineering. 2014. Vol. 1. N 1, p. 2. DOI: 10.15862/02TS114 (in Russian).
- Strokova L.A. Determination of parameters for numerical modeling of soil behavior. Izvestiya Tomskogo politekhnicheskogo universiteta. 2008. Vol. 1, p. 68-74 (in Russian).
- Khutskii V.P. Displacement of the Earth surface during the construction of subway transfer hubs in the conditions of St. Petersburg: Avtoref. dis. … kand. tekhn. nauk. St. Petersburg: Sankt-Peterburgskii gosudarstvennyi universitet putei soobshcheniya, 2003, p. 28 (in Russian).
- Shesternev D.M., Shesternev D.D. Soil heaving of rocks under conditions of cryolithozone degradation. Yakutsk: Izd-vo Instituta merzlotovedeniya, 2012, p. 194 (in Russian).
- Shuplik M.N., Borisenko V.N. Modern methods of freezing soils using solid cryoagents. Gornyi informatsionno-analiticheskii byulleten. 2004. N 1, p. 227-232 (in Russian).
- Andersland O.B., Ladanyi B. Frozen Ground Engineering: 2nd Edition. NY: Jonh Wiley & Sons, 2003, p. 384.
- Haibing Cai, Pengfei Li, Zhifeng Wu. Model Test of Liquid Nitrogen Freezing-Temperature Field of Improved Plastic Freezing Pipe. Journal of Cold Regions Engineering. 2020. Vol. 34. Iss. 1. N 04020001. DOI: 10.1061/(ASCE)CR.1943-5495.0000204
- Haibing Cai, Zheng Liu, Sheng Li, Tenglong Zheng. Improved analytical prediction of ground frost heave during tunnel construction using artificial ground freezing technique. Tunnelling and Underground Space Technology. 2019. Vol. 92. N 103050. DOI: 10.1016/j.tust.2019.103050
- Kazanin O.I., Sidorenko A.A., Meshkov A.A. Reproduction of the longwall panels: Modern requirements for the technology and organization of the development operations at coal mines. Eurasian Mining. Vol. 2020. Iss. 2, p. 19-23. DOI: 10.17580/em.2020.02.05
- Kovshov S.V. Application of Computer Modeling for the Accident Rate Assessment on Separate Sites of the Mohe–Daqing Oil Pipeline in Permafrost Conditions. Transportation Infrastructure Geotechnology. 2020. Vol. 7. Iss. 4, p. 605-617. DOI: 10.1007/s40515-020-00109-8
- Novozhenin S., Vystrchil M. New method of surface settlement prediction for Saint-Petersburg metro escalator tunnels excavated by EPM TBM. Procedia Engineering, 2016. Vol. 150, p. 2266-2271. DOI: 10.1016/j.proeng.2016.07.283
- Protosenya A.G., Karasev M.A., Belyakov N.A. Method of predicting earth surface subsidence during the construction of tunnels using TBM with face cantledge on the basis of multivariate modeling. International Journal of Civil Engineering and Technology. 2018. Vol. 9. Iss. 11, p. 1620-1629.
- Shikhov A.I., Potapov A.I. The pattern of changes in the velocity of propagation of ultrasonic waves in frozen soil samples during thawing. High-Tech and Innovations in Research and Manufacturing (HIRM-2020) 28 February 2020, Siberia, Russia. Journal of Physics: Conference Series, 2020. Vol. 1582. N 012079. DOI: 10.1088/1742-6596/1582/1/012079


