Complete extraction of conditioned ores from complex-structured blocks due to partial admixture of substandard ores
- Ph.D., Dr.Sci. Professor Kazakh National Research Technical University named after K.I.Satpayev (Satbayev University) ▪ Orcid
Abstract
The paper presents mining-technological substantiation of complete extraction of conditioned ores from complex-structured blocks of benches by mixing a layer of substandard ores of certain sizes. The relevance of the work consists in the development of innovative methods of establishing the parameters of the substandard layer of ores to be added to the conditioned ores. The main problem is to ensure complete extraction of useful components into concentrate from shipped ore with acceptable deviations from the required ones. A new typification of complex-structured ore blocks of the bench has been carried out. Analytical dependences of mining and geological characteristics of complex-structured ore blocks were obtained. Theoretical dependences for determining the main indicators of mineral processing are derived. Analytical dependences for determination of the content of useful component in shipped ore α' – mixture of conditioned ore with the content of useful component α and admixed layer of substandard ore with the content of useful component α'' are offered. For the first time in mining science, a new approach of complete extraction of conditioned ores from complex-structured blocks of benches by grabbing a certain part of substandard ores during excavation, increasing the volume of extracted ore and expanding the extraction of useful components in the concentrate has been substantiated. The increment of useful components can reach 10-15 % of the total volume of extraction, which allows predicting a significant increase in the completeness of mineral extraction from the Earth's interior.
Funding
The article was prepared as part of a project funded by the Ministry of Science and Higher Education of the Republic of Kazakhstan 2023/AP19676591 “Development of innovative technologies for the complete extraction of scattered conditioned ores from complex-structured blocks of benches”.
Introduction
Complete extraction of minerals from the subsurface, including conditioned ores, reduction of their losses and dilution during the exploitation of various deposits is one of the most acute and urgent tasks of mining science and industry [1-3]. The problem becomes especially important in the development of complex-structured ore deposits [4-6], which are usually represented by a diverse combination of ore bodies and host rocks (substandard ores) of complex configuration, different sizes and physical, technical and geological characteristics. Contacts between conditioned and substandard ores are visually indistinguishable and are probabilistic in nature. The share of complex-structured blocks at non-ferrous metallurgy enterprises of CIS countries is 60-90 %, and operational losses of ore during their development can reach 20-35 % [7-9].
The main reasons for the high level of losses and dilution during open pit mining of complex-structured minerals are insufficient study of the geological and morphological structure of complex-structured blocks of benches; inconsistency of the applied technologies of excavation and loading operations with the real mining and geological conditions of occurrence of complex-structured minerals in the massif and in the blasted state; use of private methods of determining and rationing of losses and dilution, focused on mining and geological objects with clear geological boundaries – veins, lenses, layers and stratification of deposits. Quantitative and qualitative losses of minerals in them are usually established for the contour areas of ore blocks.
The majority of non-ferrous, noble and rare metal ore deposits in Kazakhstan are complex-structured. Ore areas have complex geological and morphological structure, irregular mineralization, visually indistinguishable boundaries with host rocks. They are characterized by different shape, size of ore bodies, placement in the considered space, mineralization and physical and mechanical properties of rocks [10-12]. The totality of these features determines the degree of complexity of the geological and morphological structure of complex mineral sites. The development of innovative methods for assessing the complexity of such areas and their use to significantly reduce losses and dilution during the development of complex-structured minerals is an urgent and priority task of mining science and industry.
The purpose of the research is mining and technological justification of complete extraction of conditioned ores from complex-structured blocks of benches by means of mixing a layer of substandard ores of set sizes. In this case the extraction of useful components into concentrate with acceptable deviations from the required ones is provided.
Methods
To assess the geological and morphological structure of complex-structured ore blocks (CSOB) of the bench and its use in the effective development of these blocks, their mining and geological models are created. The mining and geological model contains data of interval sampling of exploration well cores for the content of useful components and harmful impurities, information on well depths and geometric parameters of disparate ore bodies. On the basis of these data, taking into account the requirements presented by the customer to the commercial ore, the boundaries between conditioned and substandard ores are established, ore bodies in the ore block of the bench are delineated, and the CSOB of the bench is typified.
Analysis of literature sources on modeling [13-15] and typification [16-18] of complex-structured blocks [19-21], their theoretical understanding [22-24] show that complex-structured blocks of benches can be divided into only two types by the nature of ore bodies location and their geometric parameters [10]. At the same time, the spatial arrangement of fixed ore bodies in the ore block of the bench and their fragmentation (separation of ore bodies in the block by the host rocks – unconfined ores) are considered as determining features [25-27].
Ore bodies are defined as volumes of conditioned ore reserves established by analyzing blast hole samples and other geophysical methods. They do not always coincide with the balance sheet ore reserves, as they are identified as a result of additional exploration. In addition, the host rocks in contact with the ore bodies represent substandard ores.
The first type of CSOB benches includes blocks composed of scattered continuous ore bodies of various shapes and sizes with rectilinear contacts with rock interlayers (Fig.1).

Fig.1. Types of complex-structured bench blocks composed of discontinuous continuous ore bodies: a, b – angle of bodies dip from 0 to π/2; C, D – angle of bodies dip from π/2 to π h – block height; a, 2a – block width; Si – cross-sectional area of the i-th ore body; li – length of contact lines of the i-th ore body with host rocks
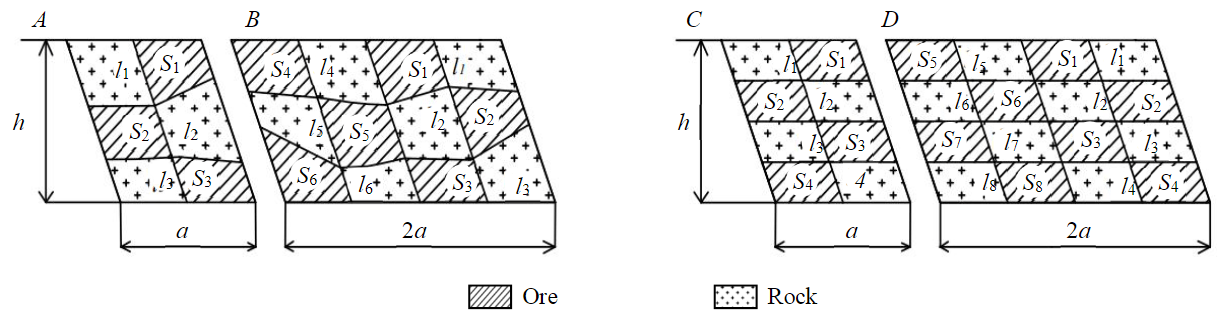
Fig.2. Types of complex-structured blocks composed of dispersed ore bodies: a, b – trapezoidal bodies; c, d – parallelogram bodies
Datamine, Surpac, Micromine, etc. software products provide only straight-line contacts in block model. They extend from one block boundary (mining unit) to another and form angles varying from 0 to π with the horizon.
The first type of CSOB benches may be represented by horizontal, inclined or vertical bedded ore bodies of relatively mature thickness (contact lines are parallel) or lens-shaped inclusions of variable thickness, between which interlayers of host rocks (unconfined ores) are placed.
The second type of CSOB benches is represented by blocks composed of dispersed ore bodies in the form of geometric figures of various shapes and sizes, such as polygons with rectilinear contacts with the host rocks (Fig. 2). The contact lines are located inside the block (mining unit). This type of complex blocks may consist of nested ore bodies of various shapes and sizes, etc.
The given types of CSOB (Fig.1, 2) serve as a basis for determining their technological characteristics, analytically interconnecting all the identified geometric parameters of the geological and morphological structure of the blocks. Only in this case they will objectively reflect the natural state of the studied object and contribute to a more complete extraction of minerals from the subsurface through the appointment of the most effective technologies of drilling and blasting and excavation-loading operations in the conditions of complex-structured blocks.
Discussion of results
Mining and geological characteristics of complex-structured ore blocks of the bench
The most common and relatively easy to measure values – the ore saturation coefficient of the block and the index of complexity of the geological structure of the block – can be considered as the sought mining and geological characteristics.
The given schemes of ore bodies placement of complex-structured blocks (Fig.1, 2) are characterized by the level of their mineral saturation. This property for the considered section of the block can be estimated by the ore saturation coefficient of the block
where Sb is the area of the considered cross-section of the complex-structured block, m2; n is the number of ore bodies.
The average ore saturation factor for the whole complex-structured block is determined by the following dependence:
where m is the number of cuts; j is the index of the current block cut.
The number of sections depends on the extent of the complex block. Each section covers a zone with an extent equal, as a rule, to the distance between wells in a row.
When determining the numerical values of kos it is necessary to take into account that in open-pit mining with the use of modern mobile mining and loading equipment the height of a complex-structured mining bench usually does not exceed 10 m, and the smallest width of the drift along the pillar is 8-10 m. In case of explosive crushing of such blocks, excavation of the mineral layer with thickness below 2.5 m is economically unprofitable and sometimes technically impossible. Therefore, the lower value of the considered indicator can be taken as 0.25, and the upper one – 0.75. This means that in most cases the ore saturation coefficient of a complex-structured block lies within the range of 0.25-0.75.
Complex-structured blocks are subdivided by the degree of ore-saturation into more ore-saturated (kos = 0.75-0.6); moderately ore-saturated (kos = 0.6-0.4); less ore-saturated (kos = 0.4-0.25).
The less ore saturated a block is, the more difficult it is to mine it without small quantitative and qualitative losses. However, as the experience of mining enterprises shows, at the same value of the ore saturation index, different final results of mineral recovery from various complex-structured blocks are achieved. The determining parameters of the block in this case are the size of the area of individual ore bodies and the lines of their contacts with the host rocks (substandard ores) in the volume under consideration, the ratio of which characterizes the degree of complexity of the structure of the CSOB and is calculated using the complexity factor of the geological and morphological structure of the block
where t' is the thickness of the layer of host rocks (or ores) falling into the ore mass (or into the shipped rock) during excavation, m.
When excluding the sum signs from dependence (1), the complexity factor of the structure of the i-th ore body in the block is determined.
The block complexity index kcf expresses the ratio of the total surface area of the adjacent layer of admixed rock or lost ore to the total surface area of ore bodies in a given section. In other words, equation (1) defines the level of quantitative and qualitative losses of ore during its extraction from the CSOB of the bench in fractions of one unit. Depending on the content of the numerator, this indicator represents losses, dilution, or both. The smaller the sum of the areas of ore bodies, the greater the complexity factor of the geological and morphological structure of the block, and vice versa. This circumstance reflects quite fully the real state of affairs at mining enterprises.
If the thickness of the near-contact layer of admixed rock or lost ore for all ore bodies of the CSOB is a constant value, it can be taken beyond the sign of the sum. Then quantitative and qualitative losses will be proportional to the ratio of the sums of contact line lengths to the total area of ore bodies in a given block section:
where µ is some proportionality factor, µ = t' (m).
As can be seen from equation (1), the index of complexity of the geological structure of ore blocks depends on the thickness of the admixed layer of rock or ore. Calculations show that for complex-structured deposits the considered criterion at the value of t', equal to 0.25 m (a tenth of the smallest thickness of the ore layer), varies within 0.1-0.3. And the greater kcf , the more complex the block structure and the greater the source of losses. Based on this position complex ore blocks of benches, according to the nature of geological and morphological structure, can be divided into complex-structured (kcf = 0.1-0.2) and more complex-structured (kcf = 0.2-0.3). If the value exceeds 0.3, selective extraction of minerals from the CSOB for economic reasons becomes very problematic, as the current costs of extraction reach irrecoverable amounts.
Analysis of mining and geological characteristics of the CSOB
The results of using the developed method of determining the mining and geological characteristics of the CSOB are presented in Table 1 by numerical values. The height of blocks was taken equal to the height of the bench, i.e. h = 10 m, width of blocks a = 9, 2a = 18 m. Areas of ore bodies Si and lengths of contact lines of ore bodies with host rocks (or substandard ores) li were assumed to be close to those in open pits of non-ferrous metallurgy of CIS countries.
Table 1
Mining and geological characteristics of CSOBs composed of discontinuous continuous ore bodies
|
A |
B |
C |
D |
|
S1(l1) = 21.12 (13.25) |
S1(l1) = 17.69 (7.64) |
S1(l1) = 34.43 (23.89) |
S1(l1) = 13.23 (8.69) |
|
S2(l2) = 26.93 (15.94) |
S2(l2) = 50.09 (28.23) |
S2(l2) = 13.89 (8.92) |
S2(l2) = 44.29 (33.48) |
|
|
S3(l3) = 29.35 (18.17) |
|
S3(l3) = 19.34 (10.54) |
|
∑Si(∑li) = 48.05 (29.19) |
∑Si(∑li) = 97.13 (54.04) |
∑Si(∑li) = 48.32 (32.81) |
∑Si(∑li) = 76.86 (52.71) |
|
kcf = 0.157; kcf = 0.148 |
kcf = 0.108; kcf = 0.141; kcf = 0.155 |
kcf = 0.173; kcf = 0.160 |
kcf = 0.164; kcf = 0.189; kcf = 0.136 |
|
kos = 0.534; kcf = 0.152 |
kos = 0.539; kcf = 0.139 |
kos = 0.536; kcf = 0.169 |
kos = 0.427; kcf = 0.171 |
Table 2
Mining and geological characteristics of CSOBs composed of dispersed ore bodies
|
A |
B |
C |
D |
|
S1(l1) = 14.59 (8.58) |
S1(l1) = 15.52 (11.5) S2(l2) = 14.50 (13.58) |
S1(l1) = 11.25 (7.16) |
S1(l1) = 11.25 (9.82) S2(l2) = 11.25 (11.66) |
|
S2(l2) = 14.41 (12.20) |
S3(l3) = 12.01 (10.3) S4(l4) = 14.95 (8.39) |
S2(l2) = 11.25 (11.66) |
S3(l3) = 11.25 (14.32) S4(l4) = 11.25(7.16) |
|
S3(l3) = 12.08 (7.64) |
S5(l5) = 17.25 (16.95) S6(l6) = 15.10 (8.19) |
S3(l3) = 11.25 (11.66) |
S5(l5) = 11.25 (7.16) S6(l6) = 11.25 (14.32) |
|
|
|
S4(l4) = 11.25 (7.16) |
S7(l7) = 11.25 (11.66) S8(l8) = 11.25 (9.82) |
|
∑Si(∑li) = 41.08 (28.42) |
∑Si(∑li) = 89.33 (68.91) |
∑Si(∑li) = 45 (37.64) |
∑Si(∑li) = 90 (85.92) |
|
kcf = 0.147; kcf = 0.212 kcf = 0.158 |
kcf = 0.185; kcf = 0.235 kcf = 0.215; kcf = 0.14 kcf = 0.245; kcf = 0.135 |
kcf = 0.159; kcf = 0.260 kcf = 0.260; kcf = 0.159 |
kcf = 0.218; kcf = 0.259 kcf = 0.318; kcf = 0.159 kcf = 0.159; kcf = 0.318 kcf = 0.259; kcf = 0.218 |
|
kos = 0.456; kcf = 0.173 |
kos = 0.496; kcf = 0.193 |
kos = 0.500; kcf = 0.209 |
kos = 0.500; kcf = 0.238 |
The values of complexity coefficients of structure of separate ore bodies and blocks are presented for variants A, B, C, D, containing ore bodies of different configurations, sizes and various mutual arrangement (Fig.1, 2).
Analysis of the data in Tables 1, 2 shows that both ore blocks are moderately ore-saturated (kos = 0.427-0.539) and complex-structured (kcf = 0.139-0.193). The exception is the CSOBs composed of dispersed ore bodies (C, D), for which kcf = 0.209-0.238. Consequently, these blocks are more complex. Blocks of the second type have a relatively higher value (kcf = 0.173-0.238) than blocks of the first type (kcf = 0.139-0.171). This predetermines a higher level of quantitative and qualitative mineral losses in blocks of the second type, which is quite natural.
The highest value of kcf has ore bodies S2, S3 (kcf = 0.260) of block B and ore bodies S3, S6 (kcf = 0.318) of block D of the second type of CSOB, i.e. at extraction of these ore bodies quantitative and qualitative losses make 26.0 and 31.8 % respectively.
Analytical definition of the main indicators of mineral processing
For mining and technological justification of complete extraction of ores from complex blocks without losses and dilution, it is necessary to consider the main indicators of the ore dressing process. The content of useful component in concentrate β is the ratio of the mass of useful component Muc to the mass of concentrate Mc, and the content of useful component in tailings δ is the ratio of the mass of useful component in tailings Mut to the mass of tailings Mt :
The numerical values of β and δ are established by chemical analysis.
Concentrate yield γc is calculated as the ratio of concentrate mass Mc to feedstock mass Mf, and tailings yield γt is the ratio of their mass Mt to feedstock mass Mf:
The recovery of useful component to concentrate εc and the recovery of useful component to tailings εt are calculated as follows:
These enrichment parameters are usually established by direct measurements and calculations [9, 28, 29]. To determine them theoretically, it is necessary to make an equation of the balance of the mass of ore entering the plant and enrichment products, as well as an equation of the balance of useful components in ore, concentrate, and tailings:
By solving equations (2) together, the following relations can be obtained:
- for concentrate yield
- tailings outlet
- to extract the useful component into a concentrate
- for recovery of a useful component in tailings
As can be seen, with known values α, β, δ by the formulas (3)-(6) the main indicators that characterize the process of mineral processing are easily calculated.
Since the masses of ore, concentrate and tailings can be measured, the recovery and yields of the beneficiation products can also be assumed to be known, as they are calculated using formulas (3)-(6).
The extraction of a useful component into the enrichment products and its yields are mutually linked by the following relationships:
From formulas (3)-(6) it follows that
These results are quite natural and confirm the validity of expressions (3)-(6). Analytical dependences (3)-(6) for determining the main enrichment parameters differ slightly in form from the known ones [30-32]. However, they are obtained by joint solution of the equations of the mass balance of ore raw materials and the balance of useful components during their processing, as well as clearly argued, which eliminates the roughness inherent in the known formulas. It follows from the dependencies that in the ideal technology of mineral raw materials beneficiation useful components in the tailings should be absent, so εc = 1, and the yield of concentrate (3) is equal to the ratio of the content of a useful component in the feedstock to the same in the concentrate. The more metal in the concentrate, the lower the concentrate yield, these conclusions are confirmed by the practice of mining and processing enterprises.
Conclusions are also confirmed by the enrichment indicators of the most common copper, lead and zinc ores with the content of useful component in the raw material αCu = 0.40-1.0, αРb = 0.8-3.0, αZn = 1.0-5.5 %; in the concentrate βCu = 20.0, βPb = 45.0, βZn = 40.0 %, in the tailings δCu = 0.06, δPb = 0.07, δZn = 0.08 %. The results of calculations are shown in Table 3 and Fig. 3. With the increase in the content of useful component in the ore, the performance of their enrichment improves, which is the case in real life. Using equations (3)-(6), it is possible to predict the mineral beneficiation indicators of interest at different values of α, β and δ.
Table 3
Ore beneficiation rates at different grades of the useful component in the ore
|
Copper ore, % |
Lead ore, % |
Zinc ore, % |
||||||||||||
|
α |
εc |
γc |
εt |
γt |
α |
εc |
γc |
εt |
γt |
α |
εc |
γc |
εt |
γt |
|
0.40 |
85.26 |
1.71 |
14.74 |
98.29 |
0.80 |
91.39 |
1.62 |
8.61 |
98.38 |
1.00 |
92.18 |
2.30 |
7.82 |
97.70 |
|
0.45 |
86.93 |
1.96 |
13.07 |
98.04 |
1.00 |
93.14 |
2.07 |
6.86 |
97.93 |
1.40 |
94.47 |
3.31 |
5.53 |
96.69 |
|
0.50 |
88.26 |
2.21 |
11.74 |
97.79 |
1.20 |
94.31 |
2.52 |
5.69 |
97.48 |
1.80 |
95.75 |
4.31 |
4.25 |
95.69 |
|
0.55 |
89.36 |
2.46 |
10.64 |
97.54 |
1.40 |
95.15 |
2.96 |
4.85 |
97.04 |
2.20 |
96.56 |
5.31 |
3.44 |
94.69 |
|
0.60 |
90.27 |
2.71 |
9.73 |
97.29 |
1.60 |
95.77 |
3.41 |
4.23 |
96.59 |
2.60 |
97.12 |
6.31 |
2.88 |
93.69 |
|
0.65 |
91.04 |
2.96 |
8.96 |
97.04 |
1.80 |
96.26 |
3.85 |
3.74 |
96.15 |
3.00 |
97.53 |
7.31 |
2.47 |
92.69 |
|
0.70 |
91.70 |
3.21 |
8.30 |
96.79 |
2.00 |
96.65 |
4.30 |
3.35 |
95.70 |
3.40 |
97.84 |
8.32 |
2.16 |
91.68 |
|
0.75 |
92.28 |
3.46 |
7.72 |
96.54 |
2.20 |
96.97 |
4.74 |
3.03 |
95.26 |
3.80 |
98.09 |
9.32 |
1.91 |
90.68 |
|
0.80 |
92.78 |
3.71 |
7.22 |
96.29 |
2.40 |
97.23 |
5.19 |
2.77 |
94.81 |
4.20 |
98.29 |
10.32 |
1.71 |
89.68 |
|
0.85 |
93.22 |
3.96 |
6.78 |
96.04 |
2.60 |
97.46 |
5.63 |
2.54 |
94.37 |
4.60 |
98.46 |
11.32 |
1.54 |
88.68 |
|
0.90 |
93.61 |
4.21 |
6.39 |
95.79 |
2.80 |
97.65 |
6.08 |
2.35 |
93.92 |
5.00 |
98.60 |
12.32 |
1.40 |
87.68 |
|
0.95 |
93.97 |
4.46 |
6.03 |
95.54 |
3.00 |
97.82 |
6.52 |
2.18 |
93.48 |
5.40 |
98.72 |
13.33 |
1.28 |
86.67 |
|
1.00 |
94.28 |
4.71 |
5.72 |
95.29 |
|
|
|
|
|
5.50 |
98.74 |
13.58 |
1.26 |
86.42 |
Justification of complete extraction of ores from complex blocks of benches
To solve the task at hand, it is necessary to pay attention to the principles of delineation of conditioned ores, which is carried out on the basis of the maximum permissible minimum value of useful components in the ore a, adopted for technological and economic reasons.
Ore volumes with the content of useful component below the specified level (< α) are considered substandard and are included in the host rocks. At the same time, from the geology of deposits, in particular ores of non-ferrous, noble and rare metals [33-35], it is known that the decrease in the content of the useful component with distance from the center of the ore body occurs gradually. This means that the content of the useful component of substandard ores directly in contact with the conditioned ores is insignificantly different from the normative. However, as the specified volume increases, the grade of the useful component in the total shipped ore decreases. The content of useful component in such ore mass in 3D is determined by the formula:
where Vco – volume of conditioned ore, m3; Vso – volume of the added layer of substandard ore, m3; λ – relative content of the useful component in the added volume of substandard ore, at the distance from the contour of the ore body equal to 0.25; 0.4; 0.5 m; λ is 0.75; 0.6; 0.5, respectively.
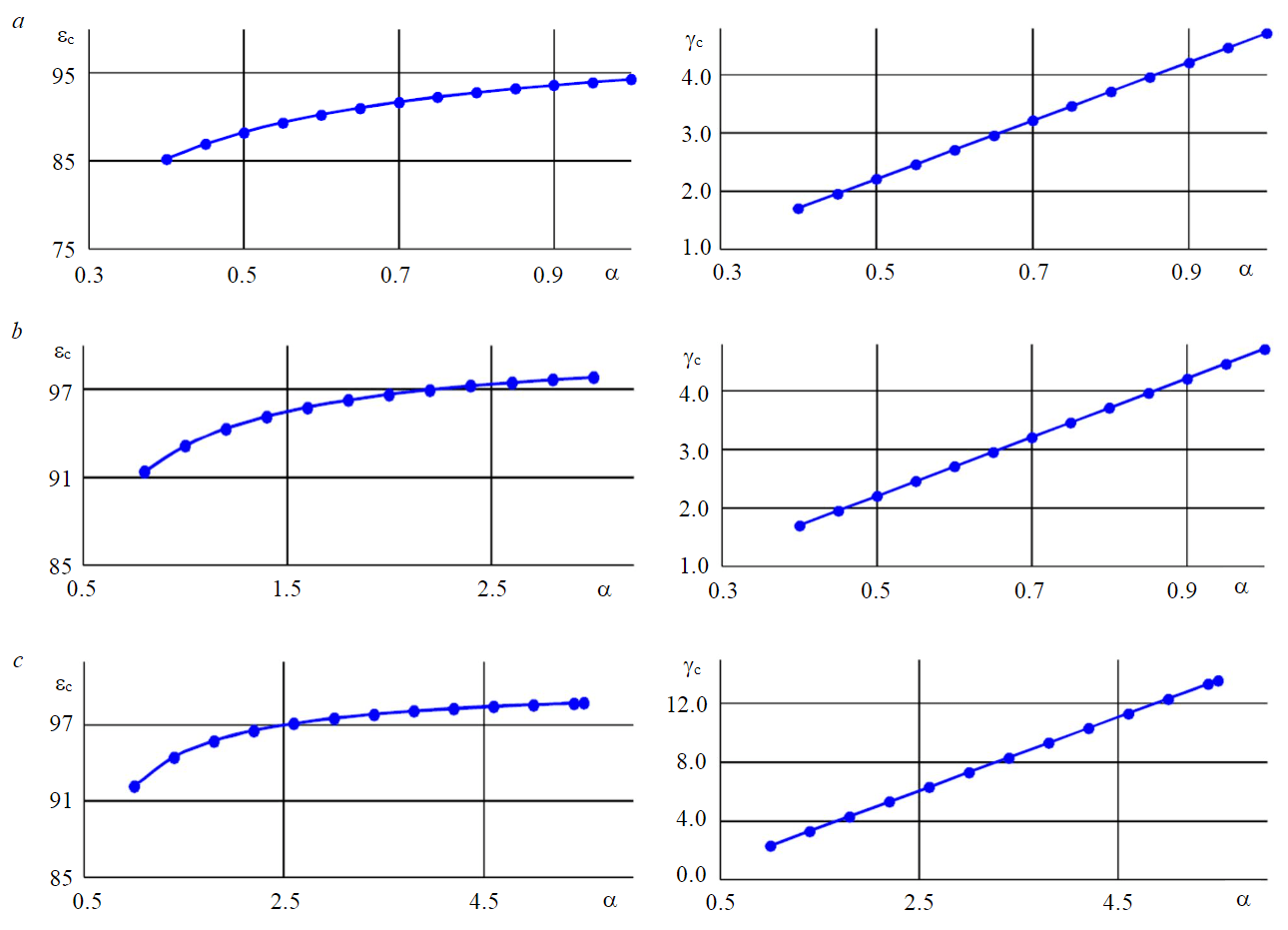
Fig.3. Graphs of change of enrichment indicators (εc, γc) of copper (a), lead (b) and zinc (c) ores depending on the content of useful component in ore
At given values of areas of ore bodies, lengths of their contact lines with substandard ores (see Fig.1, 2) the content of useful component in shipped ore in 2D-format is calculated according to the following dependence:
where t' is the thickness of admixed layers of substandard ores.
Usually, the minimum thickness t of the ore layer of complex-structured blocks at the open pits of non-ferrous metallurgy of the CIS countries is 2.5 m (Fig.4). The area of the extracted ore body per unit thickness of the ore block at this cross-section is tl, the area of admixed layers of substandard ore is 2t'l, and the area of all shipped ore is (t + 2t')l. The grade of the useful component in the shipped ore in a linear format is determined using the expression
As can be seen from equations (7)-(9), the content of the useful component in the shipped ore from complex-structured blocks is very dependent on the patterns of decreasing content of the useful component in the ore as it moves away from the center of the ore body and the size of the zones of substandard ore. Taking into account the combination of these parameters, it is possible to establish their most favorable values for the full development of established mineral reserves without losses and dilution.

Fig.4. Scheme to determine the content of useful component in the shipped ore
1 – conditioned ore; 2 – substandard ore
In general, the volumes of conditioned and mixed substandard ores at a given cross-section of complex-structured blocks (see Fig.1, 2) are determined by the following formulas:
where zi – depth of excavation of conditioned and substandard ores by excavation and loading equipment, m; ti – thickness of admixed substandard ores, m.
Acceptable (rational) is the volume of substandard ore that provides the required content of useful component in the shipped ore in accordance with expressions (7)-(9).
Testing of the methodology for determining the copper grade in shipped ore from complex blocks was carried out in the conditions of Aktogay copper ore deposit, mined by 10-meter high benches. The minimum thickness of the mined ore layer is 2.5 m. The open pit ore production capacity is 24.3 million t per annum, the current copper grade is 0.56 %, the established cut-off grade is 0.45 %, the copper grade in concentrate is 20 %, in tailings is 0.06 %, and the recovery in concentrate is 86 %.
Three variants of complete extraction of conditioned mineral reserves together with some part of substandard ores of complex-structured blocks at the minimum thickness of the ore body of 2.5 m are considered. Other values of variables: variant 1 t' = 0.25 m, λ = 0.75; variant 2 t' = 0.4, λ = 0.6; variant 3 t' = 0.5, λ = 0.5. The content of useful components in the shipped ore and their recovery into concentrate for copper, lead and zinc ores are given in Table 4.
Table 4
Mineral content in the shipped ore and their recovery into concentrate at different sizes of admixed layers of substandard ores, %
|
Metal |
Options |
|||||||
|
Initial |
1 |
2 |
3 |
|||||
|
α |
εc |
α |
εc |
α |
εc |
α |
εc |
|
|
Copper |
0.45 |
86.93 |
0.43 |
86.35 |
0.41 |
85.49 |
0.39 |
84.70 |
|
0.60 |
90.27 |
0.58 |
89.83 |
0.54 |
89.19 |
0.51 |
88.60 |
|
|
0.75 |
92.28 |
0.72 |
91.93 |
0.68 |
91.42 |
0.64 |
90.94 |
|
|
0.95 |
93.97 |
0.91 |
93.69 |
0.86 |
93.29 |
0.81 |
92.91 |
|
|
Lead |
1.00 |
93.14 |
0.96 |
92.84 |
0.90 |
92.39 |
0.86 |
91.98 |
|
1.60 |
95.77 |
1.53 |
95.58 |
1.44 |
95.30 |
1.37 |
95.04 |
|
|
2.20 |
96.97 |
2.11 |
96.83 |
1.99 |
96.63 |
1.89 |
96.44 |
|
|
2.80 |
97.65 |
2.68 |
97.54 |
2.53 |
97.38 |
2.40 |
97.23 |
|
|
Zinc |
1.40 |
94.47 |
1.34 |
94.23 |
1.26 |
93.86 |
1.20 |
93.52 |
|
2.60 |
97.12 |
2.49 |
96.98 |
2.35 |
96.79 |
2.23 |
96.60 |
|
|
4.20 |
98.29 |
4.03 |
98.21 |
3.79 |
98.09 |
3.60 |
97.97 |
|
|
5.40 |
98.72 |
5.18 |
98.65 |
4.88 |
98.56 |
4.63 |
98.47 |
|
The content of the useful component in the shipped copper ore increases with its content in the conditioned ore in all variants and decreases with distance from the contour of the ore body (Table 4). The similar tendency is characteristic for copper recovery in concentrate. However, these changes are insignificant. To assess their impact on the final result – recovery of useful component in concentrate – it is necessary to consider the deviations of indicators in the cases under consideration.
Absolute and relative deviations of these indicators are presented in Table 5. Relative deviation of useful component content in shipped ore depending on its content in conditioned ore in all variants remains at the same level – 4.17; 9.7; 14.29 %. Relative deviation of extraction of useful component in concentrate in the first variant varies from 0.67 to 0.29 %, in the second – from 1.65 to 0.72 %, in the third – from 2.56 to 1.1 %. These indicators with the change of parameters of admixed layers of substandard ores depending on the content of useful component in the conditioned ore indicate that the relative deviation of extraction of useful component in the concentrate from the required is insignificant and lies within acceptable limits. This means that at complete extraction of conditioned ores from complex blocks of benches by mixing a layer of substandard ores of certain sizes the required quality of shipped ore mass and increase of its volume are ensured.
Thus, under the new approach to the development of complex blocks, the expected diluting part of substandard ores is transferred to the category of recoverable reserves. As a result, both the volume of recoverable ore and the enhanced recovery of useful components in the concentrate increase. Such an increase of useful components may reach 10-15 % of the total volume of extraction.
Table 5
Deviations of investigated parameters from the conditioned ones at different sizes of admixed layers of substandard ores, %
|
Metal |
Options |
||||||
|
1 |
2 |
3 |
|||||
|
Δα' |
Δεc |
Δα' |
Δεc |
Δα' |
Δεc |
||
|
Copper |
0.02/4.17 |
0.58/0.67 |
0.04/9.70 |
1.44/1.65 |
0.06/14.29 |
2.23/2.56 |
|
|
0.03/4.17 |
0.44/0.48 |
0.06/9.70 |
1.08/1.19 |
0.09/14.29 |
1.67/1.85 |
||
|
0.03/4.17 |
0.35/0.38 |
0.07/9.70 |
0.86/0.93 |
0.11/14.29 |
1.34/1.45 |
||
|
0.04/4.17 |
0.28/0.29 |
0.09/9.70 |
0.68/0.72 |
0.14/14.29 |
1.06/1.12 |
||
|
Lead |
0.04/4.17 |
0.30/0.33 |
0.10/9.70 |
0.75/0.81 |
0.14/14.29 |
1.17/1.25 |
|
|
0.07/4.17 |
0.19/0.20 |
0.16/9.70 |
0.47/0.49 |
0.23/14.29 |
0.73/0.76 |
||
|
0.09/4.17 |
0.14/0.14 |
0.21/9.70 |
0.34/0.35 |
0.31/14.29 |
0.53/0.55 |
||
|
0.12/4.17 |
0.11/0.11 |
0.27/9.70 |
0.27/0.28 |
0.40/14.29 |
0.42/0.43 |
||
|
Zinc |
0.06/4.17 |
0.25/0.26 |
0.14/9.70 |
0.61/0.65 |
0.20/14.29 |
0.95/1.01 |
|
|
0.11/4.17 |
0.13/0.14 |
0.25/9.70 |
0.33/0.34 |
0.37/14.29 |
0.51/0.53 |
||
|
0.18/4.17 |
0.08/0.08 |
0.41/9.70 |
0.20/0.21 |
0.60/14.29 |
0.32/0.32 |
||
|
0.23/4.17 |
0.06/0.07 |
0.52/9.70 |
0.16/0.16 |
0.77/14.29 |
0.25/0.25 |
||
Note. Absolute deviations are given in the numerator and relative deviations in the denominator.
If the annual capacity of copper ore mine is 10 million t, and the reduction of operational losses is only 5 %, the increment of recovered ore will reach 500 thousand t. At a copper content of 0.45 % ore at a cost of 6,500 dollars/t, the economic effect will be 14,625 thousand dollars.
Conclusion
As a result of the studies carried out, a new typification of complex-structured ore blocks of the bench was carried out. The first type includes blocks composed of scattered continuous ore bodies of various shapes and sizes with rectilinear contacts with rock interlayers. The second type of benches is represented by blocks composed of scattered ore bodies in the form of polygons with rectilinear contacts with host rocks.
The ore saturation coefficient of the block and the index of complexity of the geological structure of the block are considered as the desired mining and geological characteristics of complex-structured ore blocks, analytical dependencies of these characteristics are presented.
Analysis of the numerical values of the characteristics showed that both ore blocks are moderately ore-saturated (kos = 0.427-0.539) and complex-structured (kcf = 0.139-0.193). The exceptions are variants B and D of complex-structured blocks composed of dispersed ore bodies. They are more complex-structured.
Theoretical dependencies for determining the main indicators of mineral processing are derived. They are obtained by solving the equations of the mass balance of ore raw materials and the balance of useful components during their processing.
To justify the complete extraction of ores from complex-structured blocks of benches, analytical dependences for determining the content of useful component in the shipped ore α', which is a mixture of conditioned ore with the content of useful component α and the admixed layer of substandard ore with the content of useful component α'' are proposed.
According to the values of the content of useful component in the shipped ore, the relative deviations of its extraction into concentrate were determined at different variants of changing the parameters of the admixed layer of substandard ores depending on the content of useful component in the conditioned ore. They are insignificant and lie within acceptable limits.
With the new approach to the development of complex blocks, the estimated diluting portion of substandard ore is transferred to the category of recoverable reserves. Both the volume of recoverable ore and the enhanced recovery of useful components into concentrate are increased. The increment of useful components can reach 10-15 % of the total production volume, thus creating prerequisites for a significant increase in the completeness of mineral extraction from the subsurface.
References
- Shabarov A.N., Kuranov A.D. Basic development trends in mining sector in complicating geotechnical conditions. Gornyi zhurnal. N 5, p. 5-10 (in Russian). DOI: 10.17580/gzh.2023.05.01
- Trubetskoi K.N., Peshkov A.A., Matsko N.A. Determination of the application area of methods of steeply falling deposits development with the use of preformed open pit mining space. Gornyi zhurnal. 1994. N 1, p. 51-59 (in Russian).
- Trushko V.L., Protosenya A.G. Prospects of Geomechanics Development in the Context of New Technological Paradigm. Journal of Mining Institute. Vol. 236, p. 162-166. DOI: 10.31897/PMI.2019.2.162
- Yakovlev V.L. Innovative basis of the strategy of integrated development of mineral resources. Ekaterinburg: Uralskoe otdelenie RAN, 2018, p. 360 (in Russian).
- Kantemirov V.D., Iakovlev A.M., Titov R.S. Applying geoinformation technologies of block modelling to improve the methods of assessing quality indicators of minerals. News of the Higher Institutions. Mining Journal. N 1, p. 63-73 (in Russian). DOI: 10.21440/0536-1028-2021-1-63-73
- Cheban A., Sekisov G. Complex structural ore blocks and their systematization. Transbaikal State University Journal. Vol. 26. N 6, p. 43-53 (in Russian). DOI: 10.21209/2227-9245-2020-26-6-43-53
- Lobyntsev A.K., Fomin S.I. Assessment of the influence of mining factors degree on the standard of prepared reserves when designing open-pit mining of complex structured ore deposits. Mineral Mining & Concervation. N 5, p. 40-43 (in Russian). DOI: 10.26121/RON.2021.52.15.004
- Kushnarev P.I. Hidden losses and dilution. Zoloto i tekhnologii. 2017. N 3 (37), p. 82-87 (in Russian).
- Rakishev B.R. Technological resources for improving the quality and completeness of use of the mineral raw materials. News of the National Academy of Sciences of the Republic of Kazakhstan. Series of Geology and Technical Sciences. Vol. 2. N 422, p. 116-124.
- Rakishev B.R. Development of the Bozshakol and Aktogay copper ore deposits in Kazakhstan. Gornyi zhurnal. N 1, p. 89-93 (in Russian). DOI: 10.17580/gzh.2019.01.18
- Tolovkhan B., Demin V., Amanzholov Zh. et al. Substantiating the rock mass control parameters based on the geomechanical model of the Severny Katpar deposit, Kazakhstan. Mining of Mineral Deposits. 2022. Vol. 16. Iss. 3, 123-133. DOI: 10.33271/mining16.03.123
- Suiekpayev Y.S., Sapargaliyev Y.M., Dolgopolova A.V. et al. Mineralogy, geochemistry and U-Pb zircon age of the Karaotkel Ti-Zr placer deposit, Eastern Kazakhstan and its genetic link to the Karaotkel-Preobrazhenka intrusion. Ore Geology Reviews. 2021. Vol. 131. N 104015. DOI: 10.1016/j.oregeorev.2021.104015
- Wellmann F., Caumon G. Chapter One – 3-D Structural geological models: Concepts, methods, and uncertainties. Advances in Geophysics. Elsevier. 2018. Vol. 59, p. 1-121. DOI: 10.1016/bs.agph.2018.09.001
- Dagasan Y., Renard P., Straubhaar J. et al. Pilot Point Optimization of Mining Boundaries for Lateritic Metal Deposits: Finding the Trade-off Between Dilution and Ore Loss. Natural Resources Research. 2019. Vol. 28. Iss. 1, p. 153-171. DOI: 10.1007/s11053-018-9380-9
- Masoumi I., Kamali G., Asghari O., Emery X. Assessing the Impact of Geologic Contact Dilution in Ore/Waste Classification in the Gol-Gohar Iron Ore Mine, Southeastern Iran. Minerals. 2020. Vol. 10. Iss. 4. N 336. DOI: 10.3390/min10040336
- Vasiukhina D. 3D geological modeling for mineral resource assessment of the Galeshchynske iron ore deposit, Ukraine. Geoinformatics: Theoretical and Applied Aspects 2020, 11-14 May 2020, Kyiv, Ukraine. European Association of Geoscientists & Engineers, 2020. Vol. 2020, p. 5. DOI: 10.3997/2214-4609.2020geo067
- Remezova O.O., Khrushchov D.P., Vasylenko S.P., Yaremenko O.V. Innovative approaches to information modeling of placer deposits. Geoinformatics, 11-14 May 2021. European Association of Geoscientists & Engineers, 2021. Vol. 2021, p. 6. DOI: 10.3997/2214-4609.20215521100
- Bala G., Kurylo M., Virshylo I. Modeling and Estimation of Iron Ore Reserves in the Leapfrog on the Example of the Azovsky Block Deposit. 16th International Conference Monitoring of Geological Processes and Ecological Condition of the Environment, 15-18 November 2022, Kyiv, Ukraine. European Association of Geoscientists & Engineers, 2022. Vol. 2022, p.5. DOI: 10.3997/2214-4609.2022580256
- Zeqiri R. Nickel discretization and quality review in Gllavica mine, Kosovo. Mining of Mineral Deposits. 2021. Vol. 15. Iss. 1, p. 35-41. DOI: 10.33271/mining15.01.035
- Morales N., Seguel S., Cáceres A. et al. Incorporation of Geometallurgical Attributes and Geological Uncertainty into Long-Term Open-Pit Mine Planning. Minerals. 2019. Vol. 9. Iss. 2. N 108. DOI: 10.3390/min9020108
- Osterholt V., Dimitrakopoulos R. Simulation of Orebody Geology with Multiple-Point Geostatistics – Application at Yandi Channel Iron Ore Deposit, WA, and Implications for Resource Uncertainty. Advances in Applied Strategic Mine Planning. Cham: Springer, 2018, p. 335-352. DOI: 10.1007/978-3-319-69320-0_22
- Cowan E.J. Deposit-scale structural architecture of the Sigma-Lamaque gold deposit, Canada – insights from a newly proposed 3D method for assessing structural controls from drill hole data. Mineralium Deposita. 2020. Vol. 55. Iss. 2, p. 217-240. DOI: 10.1007/s00126-019-00949-6
- Xing Jin, Gongwen Wang, Ping Tang et al. 3D geological modelling and uncertainty analysis for 3D targeting in Shanggong gold deposit (China). Journal of Geochemical Exploration. 2020. Vol. 210. N 106442. DOI: 10.1016/j.gexplo.2019.106442
- Renguang Zuo, Kreuzer O.P., Jian Wang et al. Uncertainties in GIS-Based Mineral Prospectivity Mapping: Key Types, Potential Impacts and Possible Solutions. Natural Resources Research. 2021. Vol. 30. Iss. 5, p. 3059-3079. DOI: 10.1007/s11053-021-09871-z
- Dowd P.A., Dare-Bryan P.C. Planning, Planning, Designing and Optimising Production Using Geostatistical Simulation. Advances in Applied Strategic Mine Planning. Cham: Springer, 2018, p. 421-449. DOI: 10.1007/978-3-319-69320-0_26
- Rakishev B., Rakisheva Z., Auezova A., Orynbay A. Automated determination of rock crushing zones in the collapse. Mining of Mineral Deposits. 2022. Vol. 16. Iss. 3, p. 109-114. DOI: 10.33271/mining16.03.109
- Lukichev S.V., Nagovitsyn O.V., Shishkin A.S. Break line and shotpile surfaces modeling in design of large-scale blasts. Mining goes Digital: Proceedings of the 39th International Symposium “Application of Computers and Operations Research in the Mineral Industry”, 4-6 June 2019, Wroclaw, Poland. London: CRC Press, 2019, p. 279-285. DOI: 10.1201/9780429320774
- Pelikh V.V., Salov V.M., Burdonov A.E., Lukyanov N.D. Model of baddeleyite recovery from dump products of an apatite-baddeleyite processing plant using a CVD6 concentrator. Journal of Mining Institute. Vol. 248, p. 281-289. DOI: 10.31897/PMI.2021.2.12
- Mutalova M., Khakimova D. Investigation of the recovery of useful components from slags by the flotation International Journal of Advanced Technology and Natural Sciences. 2020. Vol. 1. N 2, p. 26-30 (in Russian). DOI: 10.24412/2181-144X-2020-2-26-30
- Opalev A.S., Alekseeva S.A. Methodological substantiation of the choice for optimal modes of equipment operation during the stage-wise concentrate removal in iron ores beneficiation. Journal of Mining Institute. Vol. 256, p. 593-602. DOI: 10.31897/PMI.2022.80
- Pelevin A.E. Using the results of the phase composition of magnetit ore for prediction of the concentrate yield. Mining Informational and Analytical Bulletin. N 5-1, p. 131-144 (in Russian). DOI: 10.25018/0236_1493_2022_51_0_131
- Golik V.I., Razorenov Y.I., Brigida V.S., Burdzieva O.G. Mechanochemical technology of metal mining from enriching tails. Bulletin of the Tomsk Polytechnic University. Geo Аssets Engineering. Vol. 331. N 6, p. 175-183 (in Russian). DOI: 10.18799/24131830/2020/6/2687
- Ovseychuk V. Classification of ore formations of Streltsovsky type uranium deposits. Transbaikal State University Journal. Vol. 27. N 8, p. 35-47 (in Russian). DOI: 10.21209/2227-9245-2021-27-8-35-47
- Turtygina N.A., Okhrimenko A.V. Quantitative assessment of natural variation in quality of copper ore in roof of intrusion. Mining Informational and Analytical Bulletin. N 8, p. 146-156 (in Russian). DOI: 10.25018/0236-1493-2019-08-0-146-156
- Zaskind E.S., Konkina O.M. Sulfide Cu-Ni and PGM deposit typification for forecasting and prospecting. National Geology. N 2, p. 3-15 (in Russian). DOI: 10.24411/0869-7175-2019-10010
