Adaptation of transient well test results
- 1 — Associate Professor Perm National Research Polytechnic University ▪ Orcid
- 2 — Associate Professor Perm National Research Polytechnic University ▪ Orcid
- 3 — Ph.D. Associate Professor Institute of Mechanics, Chinese Academy of Sciences ▪ Orcid
Abstract
Transient well tests are a tool for monitoring oil recovery processes. Research technologies implemented in pumping wells provide for a preliminary conversion of measured parameters to bottomhole pressure, which leads to errors in determining the filtration parameters. An adaptive interpretation of the results of well tests performed in pumping wells is proposed. Based on the original method of mathematical processing of a large volume of field data for the geological and geophysical conditions of developed pays in oil field, multidimensional models of well flow rates were constructed including the filtration parameters determined during the interpretation of tests. It is proposed to consider the maximum convergence of the flow rate calculated using a multidimensional model and the value obtained during well testing as a sign of reliability of the filtration parameter. It is proposed to use the analysis of the developed multidimensional models to assess the filtration conditions and determine the individual characteristics of oil flow to wells within the pays. For the Bashkirian-Serpukhovian and the Tournaisian-Famennian carbonate deposits, the influence of bottomhole pressure on the well flow rates has been established, which confirms the well-known assumption about possible deformations of carbonate reservoirs in the bottomhole areas and is a sign of physicality of the developed multidimensional models. The advantage of the proposed approach is a possibility of using it to adapt the results of any research technology and interpretation method.
Introduction
Transient well tests (WT) are the most valuable tool for monitoring the hydrocarbon extraction processes at all stages of the field development [1, 2]. Currently, several different technologies for conducting these tests are known [3]. Measurements of bottomhole pressure after shutting down the well can be performed mainly in the flowing wells [4, 5]. The presence of downhole pumping equipment in the well does not allow lowering a pressure gauge to the bottom hole [6], so testing is conducted either by recording the wellhead parameters (annular pressure, dynamic level), which are subsequently converted to bottomhole pressure, or by recording the rate of pressure change at the pump intake [7]. It is generally accepted that the technology based on measuring the wellhead parameters is reduced to plotting of the level recovery curve (LRC), pressure at the pump inlet – pressure build-up curve (PBC) [8]. The choice of testing technology is determined, as a rule, by the arrangement of the downhole pumping equipment in the well that is the object of study [1]. It should be borne in mind that both technologies for testing pumping wells involve the recalculation of the measured parameters, which, undoubtedly, can lead to errors [9, 10].
This testing technology is based on measurements in wells after they have been shut down, and testing is considered as high-quality if the bottomhole pressure is almost completely built-up to the formation pressure value [11, 12]. In medium- and low-yield wells, pressure build-up can occur for an exceptionally long time period, which leads to such undesirable effects as the failure to sufficiently build-up and technological difficulties in putting the well into operation after a long-term shutdown [13-16]. Currently, the methods for determining the hydrodynamic parameters of reservoir systems that do not involve long-term shutdown of wells have become widespread; for example, the method of pressure stabilization curve (PSC) recording [17, 18] or the method based on production analysis (PA) [19, 20].
Thus, at present various methods for determining the filtration parameters differing both in the technological features of measurements, and in mathematical fundamentals and principles of interpretation are used in oil production practice [21]. When implementing several test technologies in one well, different parameter values are often obtained [22, 23].
In oil fields of the Perm Territory, a parallel test is often performed which involves simultaneous taking of the LRC and PBC readings due to possible failures of sensors under the pumps as well as technological problems in recording the wellhead parameters. For example, due to foam formation in the annulus, it is not always possible to determine the level of liquid [24, 25]. At the same time, the interpretation of the LRC and PBC data obtained in one period in the same well often leads to obtaining different values of the determined parameters [26]. As an example, the Figure shows a comparison of permeability values determined by processing the parallel obtained LRC and PBC. The permeability values determined using two technologies differ, especially in the range of high values, despite a quite high correlation coefficient r.
The above methods for determining the filtration parameters (permeability, etc.) are indirect, solvable within the framework of the second (inverse) problem of subsurface hydromechanics [1]. The most obvious way to assess the reliability of indirect methods is to compare them with the results of direct, immediate measurements. However, it is impossible to measure the phase permeability during the pay development in the drainage zone of a specific well, which, in essence, is the per-meability determined by well testing [27, 28]. Therefore, the development and substantiation of indirect approaches for adaptive well testing results and assessment of reliability of the determined filtration parameters of reservoir systems should be considered as the most important task of efficient and reliable monitoring of the development of hydrocarbon fields [29].
Methodology
The paper presents the results of developing a methodology for adapting and assessing the reliability of the reservoir parameters determined by the LRC, PBC, PSC and PA methods when testing oil-producing wells in the fields of the Perm Territory.
To solve the problem, digital data sets of a large volume were used – the results of numerous field tests (over 9,000 determinations) of wells operating in the fields of the region. Only highly informative standard tests were accepted for analysis; data characterized by the presence of interference, noise, technology violations, etc. were excluded from the total sample.
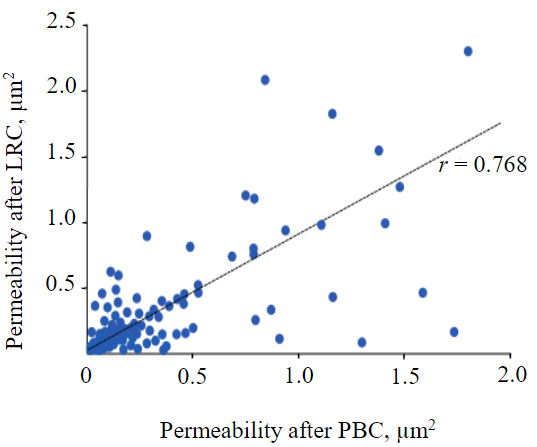
Dependence of permeability values of terrigenous Visean deposits obtained from interpretation of PBC and LRC data
In the fields under consideration, the main pays are confined to carbonate deposits of the Tournaisian-Famennian and Bashkirian ages as well as to terrigenous Visean deposits. It should be noted that there are major differences in the properties of terrigenous and carbonate pays and between different carbonate pays. In the course of further research, the pays were classified under three groups according to their geological age.
The main idea of the proposed approach is as follows. All the parameters that are determined during the WT interpretation (permeability, skin factor, formation pressure) ultimately determine the productive characteristics of the well and its flow rate and can be used as input data in the individual inflow equations – models for determining the flow rates. Thus, construction of a series of individual models of well flow rates, including the hydrodynamic parameters determined by a certain test technology, and assessment of their performance will allow solving the problem. The parameters which, when substituted into the equation, allow achieving the maximum compliance of the calculated (model) flow rate with its actual value should be considered reliable.
When constructing well flow models, an approach was used allowing the most complete account of the individual geological and geophysical characteristics of the fields under consideration – a multidimensional mathematical modelling. This tool is extensively used to solve other technological problems, for example, in the assessment of the results of geotechnical measures [30, 31], the assessment of the hydrodynamic association between wells [11], and the efficiency of wells [32, 33], etc.
To construct the models, the most representative sample was used including all the results of WT for all the implemented technologies as well as the full range of field geological information. It should be noted that all the field data, except those subject to adaptation, are characterized by simplicity, reliability, and the necessary regularity of practical determination: casing pressure Рcas; saturation pressure Psat; test time Tt; liquid flow rate Ql; water cut W; porosity coefficient averaged over the section kpor; effective oil-saturated pay thickness h; oil viscosity μo; oil volumetric coefficient b; gas factor Gf.
The construction of multidimensional mathematical models is accomplished in special software products that are extensively used to solve similar scientific and practical tasks [24, 34].
Of particular interest is the analysis of the process proper of constructing models and the type of resulting equations. Since the modelled parameter is the flow rate, the analysis of the equations for its forecast will make it possible to identify the characteristics that control the flow rate in individual conditions under study. Moreover, the earlier a certain characteristic is included in the model, the greater its impact on the predicted value. Thus, the multidimensional mathematical modelling of the flow rates in this case will make it possible not only to solve the target problem – adaptation of well test results based on the use of different technologies, but also to study the conditions of liquid filtration in the fields of the region as well as to develop the individual inflow (flow rate) equations.
The first stage of mathematical modelling of flow rates is a comparison of the average values of all the used indicators according to test technologies, the interpretation methods based on the data on all the developed pays (three groups of reservoirs were studied – Visean terrigenous, carbonate Tournaisian-Famennian and Bashkirian deposits). For this purpose, a well-known statistical tool was used – the Student’s test. Subsequently, for a more complete statistical analysis, a comparison was made of the distributions of indicator values determined using different test technologies by means of another well-known tool – Pearson statistics. A comprehensive assessment of differences in test technologies was performed using a stepwise linear discriminant analysis (SLDA). After a comprehensive assessment performed using the SLDA, a linear discriminant function (LDF) was constructed by compiling a matrix of the centred sums of squares and mixed products, from which the sample matrix was computed. Subsequently, to determine the coefficients of linear discriminant functions, the inverse sample covariance matrices were constructed, from which the boundary values of the discriminant functions were calculated dividing the sample into subsets. The classification reliability is also calculated using the Pearson criterion.
Before constructing the models, a comprehensive statistical assessment of the used source data is performed, which allows further confident use of the multidimensional mathematical modelling to solve the problem.
It is assumed that the presence of statistically significant multivariate associations is a quantitative justification for the use of field data. It should be noted that the multidimensional model is constructed jointly using all the data obtained by the LRC, PBC, PSC, and PA technologies. This allows avoiding subjectivity when conducting further tests.
Results
An algorithm has been developed for the practical use to adapt the well test results, which includes several stages.
1. Selection of the calculation model in accordance with the developed pay:
- for terrigenous pays
at R = 0.808; р < 0.000000;
- for carbonate pays of Tournaisian-Famennian age
at R = 0.740; р < 0.000000;
- for carbonate pays of Bashkirian age
at R = 0.674; р < 0.000000.
2. Collection of field data – values of indicators used in the adopted model.
3. Calculation of the model value of liquid flow rate using the test interpretation results (LRC/PBC/PSC/PA).
4. Comparison of model and actual liquid flow rate. The results of interpretation based on the technology with model flow rate that has a minimal deviation from the actual value should be considered as the most reliable.
Practical use of the developed algorithm for adapting WT results
At one of producing wells in the oil field (pay Tl-Bb) in the Perm Territory, the test was performed with simultaneous implementation of two technologies characterized by parallel recording of wellhead (LRC) and deep (PBC) parameters. At the same time, the interpretation of the results of deep and wellhead measurements led to significantly different filtration characteristics:
Interpretation of data from the implemented LRC and PBC technologies demonstrates different results, and the assessment of the most reliable value can be made using the developed adaptation algorith m.
Field data
|
Parameter |
Value |
|
|
PBC |
LRC |
|
|
Actual liquid flow rate Ql, m3/day |
46 |
|
|
Reservoir pressure Рres, MPa |
13.74 |
|
|
Casing pressure Рcas, MPa |
0.59 |
|
|
Bottomhole pressure Рbth, MPa |
10.83 |
9.33 |
|
Build-up time of PBC, LRC Тt, h |
118 |
145.6 |
|
Water cut W, % |
55 |
|
|
Pay thickness h, m |
7.2 |
|
|
Skin factor S, rel. units |
12.3 |
–3.34 |
|
Piezoconductivity factor αp, cm2/s |
4296 |
877.6 |
At the first stage, a model is selected that corresponds to the pay operated by the well under study. Since the analysed producing well operates the Tula-Bobrikovsky pay, equation (1) is used for further calculations.
At the second stage, all the necessary data included in equation (1) as source data are collected. The source data for calculating the model flow rate values are presented in the Table.
At the third stage, the model value of liquid flow rate is calculated using the results of the PBC interpretation
Model value of liquid flow rate is calculated using the results of the LRC interpretation
At the fourth stage, a comparison of model and actual liquid flow rates is made. As can be seen from the calculated flow rates, the minimum deviation from the actual value is recorded when using the results of the PBC technology. Accordingly, the permeability value obtained from the interpretation data of the PBC technology is more reliable than when processing data of the LRC technology.
Discussion
This article describes the results of research aimed at developing a series of multidimensional mathematical models of well flow rates in individual geological and geophysical conditions of the developed pays in the deposits of three geological ages. The developed models can be used to solve different problems, both practical, and theoretical that are of scientific interest.
The practical value of the developed models consists in a possibility of their use for adapting the filtration parameters of the pays when implementing different well test technologies in pumping wells. The adaptation process in compliance with the proposed approach is quite simple, the practical determination of the source data for its implementation is not difficult, and no special software pro-ducts are required for computations.
Analysis of the composition of mathematical models made it possible to determine the individual conditions for liquid inflow for the pays, which determines the theoretical significance of the tests accomplished. From the theory of mathematical statistics, it is known that the analysis of multiple regression equations allows evaluating the influence of the independent variable on the predicted value as well as the direction of this influence. However, in this case, only the assessment of the influence can be regarded as well grounded without considering its direction, since the input para-meters of the models are not strictly independent, there is a correlation between them. It is obvious that in a single reservoir-well hydrodynamic system there are no completely independent parameters. When constructing the models, the stepwise regression analysis is applied, and the combination of the input parameters does not always correspond to their individual influence on the predicted value. The inclusion of each subsequent factor in the model also adjusts the resulting model. Thus, the ana-lysis of equations (1)-(3) allows drawing the following conclusions:
- The equations obtained for the three pays are quite different in the set of parameters included, which confirms the specificity and individuality of the geological and geophysical conditions for the development of their reserves.
- In the first positions in the flow rate equations constructed for carbonate deposits of both the Bashkirian-Serpukhovian and Tournaisian-Famennian ages, bottomhole pressure is included, i.e., bottomhole pressure is a parameter that forms the production rate of wells in these reservoirs [35, 36]. This pattern confirms the well-known hypothesis about the influence of the value of bottomhole pressure on the productive characteristics of carbonate reservoirs due to their probable deformation in the bottomhole area. This points to advisability of a comprehensive justification of well operation modes under the given conditions.
- The parameter characterizing the state of the pay in the bottomhole area, skin factor, is included only in the model for the terrigenous Tula-Bobrikovsky deposits. This confirms the assumption that a change (especially decrease) of permeability in the bottomhole area is a phenomenon that is most characteristic of the terrigenous deposits [25]. In the practice of WT interpretation, it is known that the range of variations in skin factors for the terrigenous reservoirs is much broader than for the carbonate ones.
- Gas factor and saturation pressure of oil with gas – the parameters characterizing the process of oil degassing – are included only in the model for the Tournaisian-Famennian deposits, which can be accounted for by the maximum gas saturation values of reservoir oil in these deposits for the region [1].
Conclusion
The study is intended to adapt the results of transient well tests when applying different technologies for their implementation.
The proposed approach is based on the use of targeted multidimensional mathematical models. The construction of the models proper is based on the processing of digital sets of a large volume – databases of production companies including the results of numerous field tests of wells throughout the history of their operation. A distinctive feature of the constructed models is the absence of difficulties in the practical determination of the input parameters and the simplicity of mathematical calculations. The main characteristic of the proposed approach is the possibility of using it not only in a comparative analysis of the reliability of the results of interpretation of the considered technologies (LRC, PBC, PSC, and PA), but also in providing grounds for the method of interpreting the test materials, choosing the software products and the models used.
The scientific novelty of the conducted tests is the determination of individual patterns of oil inflow for the considered developed pays, identification of the main factors determining the flow rates of wells in terrigenous and carbonate reservoirs of oil fields in the Perm Territory.
The original mathematical processing of digital sets of large volume – field data – is a current trend in development of the theory and practice of oil and gas engineering.
References
- Davydova A.E., Shchurenko A.A., Dadakin N.M. et al. Well testing design development in carbonate reservoir. Bulletin of the Tomsk Polytechnic University. Geo Аssets Engineering. 2019. Vol. 330. N 6, p. 68-79 (in Russian). DOI: 10.18799/24131830/2019/6/2128
- Sergeev V.L., Nguyen Ph.T.H. Models and algorithms for adaptive interpretation of combined well test data of intelligent wells. Bulletin of the Tomsk Polytechnic University. Geo Аssets Engineering. 2018. Vol. 329. N 10, p. 67-75 (in Russian). DOI: 10.18799/24131830/2018/10/2106
- Xin Du, Zhiwei Lu, Dongmei Li et al. A novel analytical well test model for fractured vuggy carbonate reservoirs considering the coupling between oil flow and wave propagation. Journal of Petroleum Science and Engineering. 2019. Vol. 173, p. 447-461. DOI: 10.1016/j.petrol.2018.09.077
- Jun He, Kegang Ling. Measuring permeabilities of Middle-Bakken samples using three different methods. Journal of Natural Gas Science and Engineering. 2016. Vol. 31, p. 28-38. DOI: 10.1016/j.jngse.2016.03.007
- Martyushev D.A. Improving the geological and hydrodynamic model of a carbonate oil object by taking into account the permeability anisotropy parameter. Journal of Mining Institute. 2020. Vol. 243, p. 313-318. DOI: 10.31897/PMI.2020.3.313
- Davydova А.E., Shchurenko A.A., Dadakin N.M. et al. Optimization of carbonate reservoir well testing. Perm Journal of Petroleum and Mining Engineering. 2018. Vol. 17. N 2, р. 123-135 (in Russian). DOI: 10.15593/2224-9923/2018.2.32.3
- Martyushev D.A., Ponomareva I.N., Galkin V.I. Conditions for Effective Application of the Decline Curve Analysis Method. Energies. 2021. Vol. 14. N 6461. DOI: 10.3390/en14206461
- Martyushev D.A., Slushkina A.Yu. Assessment of informative value in determination of reservoir filtration parameters based on interpretation of pressure stabilization curves. Bulletin of the Tomsk Polytechnic University. Geo Аssets Engineering. 2019. Vol. 330. N 10, p. 26-32 (in Russian). DOI: 10.18799/24131830/2019/10/2295
- Sergeev V.L., Dong Van Hoang, Khagay D.E., Ignatenko A.V. Diagnostic criteria for identification of filtration flows during horizontal well tests. Bulletin of the Tomsk Polytechnic University. Geo Аssets Engineering. 2020. Vol. 331. N 2, p. 181-187 (in Russian). DOI: 10.18799/24131830/2020/2/2504
- Jirjees A.Y., Abdulaziz A.M. Influences of uncertainty in well log petrophysics and fluid properties on well test interpretation: An application in West Al Qurna Oil Field, South Iraq. Egyptian Journal of Petroleum. 2019. Vol. 28. Iss. 4, p. 383-392. DOI: 10.1016/j.ejpe.2019.08.005
- Bulygin D.V., Nikolaev A.N., Elesin A.V. Hydrodynamic evaluation of the efficiency of flow deflecting technologies in conditions of formation of man-made filtration channels. Georesources. 2018. Vol. 20. N 3. Part 1, p. 172-177. DOI: 10.18599/grs.2018.3.172-177
- 12. Elesin A.V., Kadyrova A.Sh., Nikiforov A.I. Definition of the reservoir permeability field according to pressure measurements on wells with the use of spline function. Georesources. 2018. Vol. 20. N 2, p. 102-107. DOI: 10.18599/grs.2018.2.102-107
- Galkin V.I., Martyushev D.A., Ponomareva I.N., Chernykh I.A. Developing features of the near-bottomhole zones in productive formations at fields with high gas saturation of formation oil. Journal of Mining Institute. 2021. Vol. 249, p. 386-392. DOI: 10.31897/PMI.2021.3.7
- Dvoynikov M.V., Budovskaya M.E. Development of a hydrocarbon completion system for wells with low bottomhole temperatures for conditions of oil and gas fields in Eastern Siberia. Journal of Mining Institute. 2022. Vol. 253, p. 12-22. DOI: 10.31897/PMI.2022.4
- 15. Decheng Zhang, Ranjith P.G., Perera M.S.A., Zhang C.P. Influences of test method and loading history on permeability of tight reservoir rocks. Energy. 2020. Vol. 195. N 116902. DOI: 10.1016/j.energy.2020.116902
- Hamada G., Joseph V. Developed correlations between sound wave velocity and porosity, permeability and mechanical properties of sandstone core samples. Petroleum Research. 2020. Vol. 5. Iss. 4, p. 326-338. DOI: 10.1016/j.ptlrs.2020.07.001
- Mirhashemi M., Khojasteh E.R., Manaman N.Sh., Makarian E. Efficient sonic log estimations by geostatistics, empirical petrophysical relations, and their combination: Two case studies from Iranian hydrocarbon reservoirs. Journal of Petroleum Science and Engineering. 2022. Vol. 213. N 110384. DOI: 10.1016/j.petrol.2022.110384
- Ruiliang Guo, Qichao Xie, Xuefeng Qu et al. Fractal characteristics of pore-throat structure and permeability estimation of tight sandstone reservoirs: A case study of Chang 7 of the Upper Triassic Yanchang Formation in Longdong area, Ordos Basin, China. Journal of Petroleum Science and Engineering. 2020. Vol. 184. N 106555. DOI: 10.1016/j.petrol.2019.106555
- Mason H.E., Smith M.M., Carroll S.A. Calibration of NMR porosity to estimate permeability in carbonate reservoirs. International Journal of Greenhouse Gas Control. 2019. Vol. 87, p. 19-26. DOI: 10.1016/j.ijggc.2019.05.008
- Jiazheng Qin, Shiqing Cheng, Peng Li et al. Interference well-test model for vertical well with double-segment fracture in a multi-well system. Journal of Petroleum Science and Engineering. 2019. Vol. 183. N 106412. DOI: 10.1016/j.petrol.2019.106412
- Cheng Jing, Xiaowei Dong, Wenhao Cui et al. Artificial neural network–based time-domain interwell tracer testing for ultralow-permeability fractured reservoirs. Journal of Petroleum Science and Engineering. 2020. Vol. 195. N 107558. DOI: 10.1016/j.petrol.2020.107558
- 22. Zaitsev M.V., Mikhailov N.N., Tumanova E.S. Non-linear filtration models and the effect of nonlinearity parameters on flow rates in low-permeability reservoirs. Georesources. 2021. Vol. 23. N 4, p. 44-50. DOI: 10.18599/grs.2021.4.5
- 23. Kutukova N.M., Shuster V.L. Modern Methods for Studying of the Heterogeneous Structure of Complex Carbonate Reservoirs and Erosion Basement. Moscow University Geology Bulletin. 2021. Vol. 76. N 1, p. 87-93. DOI: 3103/S0145875221010051
- Smetkina M.A., Melkishev O.A., Prisyazhnyuk M.A. Refining the Values of Permeability when Adapting the Hydrodynamic Model. Perm Journal of Petroleum and Mining Engineering. 2020. Vol. 20. N 3, р. 223-230 (in Russian). DOI: 10.15593/2712-8008/2020.3.3
- Shamsiev M.N. The results interpretation of thermogasdynamic studies of vertical gas wells incomplete in terms of the reservoir penetration degree. Georesources. 2018. Vol. 20. N 1, p. 39-43. DOI: 10.18599/grs.2018.1.39-43
- ShelepovV., Tyukavkina O.V. Geophysical methods for determining the reservoir properties of structurally complex lower-middle jurassic reservoirs within hydrocarbon fields in the latitudinal ob region. Moscow University Geology Bulletin. 2020. Vol. 75. N 2, p. 145-149. DOI: 10.3103/S0145875220020088
- Pandey R.K., Kumar A., Mandal A. A robust deep structured prediction model for petroleum reservoir characterization using pressure transient test data. Petroleum Research. 2022. Vol. 7. Iss. 2, p. 204-219. DOI: 10.1016/j.ptlrs.2021.09.003
- 28. Kryganov P.V., Afanaskin I.V., Volpin S.G. et al. Searching for reservoirs with bypassed oil using well tests and mathema-tical modeling. Journal of Petroleum Science and Engineering. 2021. Vol. 206. N 109077. DOI: 10.1016/j.petrol.2021.109077
- 29. Ali M.A., Umer R., Khan K.A., Cantwell W.J. Application of X-ray computed tomography for the virtual permeability prediction of fiber reinforcements for liquid composite molding processes: A review. Composites Science and Technology. 2019. 184. N 107828. DOI: 10.1016/j.compscitech.2019.107828
- 30. Iktissanov V.A. Description of steady inflow of fluid to wells with different configurations and various partial drilling-in. Journal of Mining Institute. 2020. Vol. 243, p. 305-312. DOI: 10.31897/PMI.2020.3.305
- 31. Galkin V.I., Ponomareva I.N., Cherepanov S.S. et al. New approach to the study of the resultsof hydraulic fracturing (on the example of Bobrikovsky deposits of the Shershnevsky field). Bulletin of the Tomsk Polytechnic University. Geo Аssets Engineering. 2020. Vol. 331. N 4, p. 107-114 (in Russian). DOI: 10.18799/24131830/2020/4/2598
- 32. Virstyuk A.Yu., Mikshina V.S. Application of regression analysis to evaluate the efficiency of oil well operating with the paraffin oil. Bulletin of the Tomsk Polytechnic University. Geo Аssets Engineering. 2020. Vol. 331. N 1, p. 117-124 (in Russian). DOI: 10.18799/24131830/2020/1/2453
- Xiangzeng Wang, Xiangyang Qiao, Naizhe Mi, Ruogu Wang. Technologies for the benefit development of low-permeability tight sandstone gas reservoirs in the Yan'an Gas Field, Ordos Basin. Natural Gas Industry B. 2019. Vol. 6. Iss. 3, p. 272-281. DOI: 10.1016/j.ngib.2018.11.017
- Abrosimov А.А., Shelyago E.V., Yazynina I.V. Justification of Representative Data Volume of Porosity and Permeability Properties for Obtaining Statistically Reliable Petrophysical Connections. Journal of Mining Institute. 2018. Vol. 233, p. 487-491. DOI: 10.31897/PMI.2018.5.487
- Wenrui Hu, Yi Wei, Jingwei Bao. Development of the theory and technology for low permeability reservoirs in China. Petroleum Exploration and Development. 2018. Vol. 45. Iss. 4, p. 685-697. DOI: 10.1016/S1876-3804(18)30072-7
- Yassin M.R., Alinejad A., Asl T.S., Dehghanpour H. Unconventional well shut-in and reopening: Multiphase gas-oil interactions and their consequences on well performance. Journal of Petroleum Science and Engineering. 2022. Vol. 215. Part B. N 110613. DOI: 10.1016/j.petrol.2022.110613
